Talk:Mercator projection/Archive 1: Difference between revisions
Archiving 32 discussion(s) from Talk:Mercator projection) (bot |
move two more threads to archive page |
||
| Line 1: | Line 1: | ||
{{Automatic archive navigator}} |
{{Automatic archive navigator}} |
||
==Just a question== |
|||
The "Controversy" section of this article is an awful lot like the "The West Wing" episode, "Somebody's Going to Emergency, Somebody's Going to Jail". This may be because the show is well researched, which is fine. However it could be because someone has seen the show and then added what they believe is facts from the real world to the article without other sources. Are there sources to suggest that there is much criticism of specifically the Mercator projection? [[User:Tompagenet|Tompagenet]] 22:54, 4 Jan 2005 (UTC) |
|||
: I don't recall having seen that particular episode, but the information in the section in question is so basic (grade school stuff in the vaguest of terms) that I don't think anyone could argue either plagiarism or factual inaccuracy. As for criticism, the section contains links to articles which go into far more detail and cite references. [[User:Austin Hair|''ADH'']] ([[User talk:Austin Hair|t]]&[[Special:emailuser/Austin Hair|m]]) 00:57, Jan 5, 2005 (UTC) |
|||
== Headline text == |
== Headline text == |
||
| Line 9: | Line 15: | ||
<!-- Template:Unsigned IP --><small class="autosigned">— Preceding [[Wikipedia:Signatures|unsigned]] comment added by [[Special:Contributions/128.135.96.228|128.135.96.228]] ([[User talk:128.135.96.228#top|talk]]) 02:58, 23 January 2006 (UTC)</small> (moved from the main page) |
<!-- Template:Unsigned IP --><small class="autosigned">— Preceding [[Wikipedia:Signatures|unsigned]] comment added by [[Special:Contributions/128.135.96.228|128.135.96.228]] ([[User talk:128.135.96.228#top|talk]]) 02:58, 23 January 2006 (UTC)</small> (moved from the main page) |
||
== That ''rv'' might need explanation == |
|||
Regarding [http://en.wikipedia.org/enwiki/w/index.php?title=Mercator_projection&diff=77935118&oldid=77926372 this edit of mine]: formula did look wrong, but not because of erroneous input{{spaced ndash}}"My preferences/[Math] (•) HTML if very simple or else PNG" was at fault. <small><span class="autosigned">—Preceding [[Wikipedia:Signatures|unsigned]] comment added by [[User:Saimhe|Saimhe]] ([[User talk:Saimhe|talk]] • [[Special:Contributions/Saimhe|contribs]]) 2006-09-28T00:09:21</span></small><!-- Template:Unsigned --> |
|||
==Image about relationship between latitude and position on map== |
==Image about relationship between latitude and position on map== |
||
Latest revision as of 02:05, 3 June 2023
| This is an archive of past discussions about Mercator projection. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. |
| Archive 1 | Archive 2 |
Just a question
The "Controversy" section of this article is an awful lot like the "The West Wing" episode, "Somebody's Going to Emergency, Somebody's Going to Jail". This may be because the show is well researched, which is fine. However it could be because someone has seen the show and then added what they believe is facts from the real world to the article without other sources. Are there sources to suggest that there is much criticism of specifically the Mercator projection? Tompagenet 22:54, 4 Jan 2005 (UTC)
- I don't recall having seen that particular episode, but the information in the section in question is so basic (grade school stuff in the vaguest of terms) that I don't think anyone could argue either plagiarism or factual inaccuracy. As for criticism, the section contains links to articles which go into far more detail and cite references. ADH (t&m) 00:57, Jan 5, 2005 (UTC)
Headline text
One of the reasons I can rationalize proposing using a Mercator Projection in a recent proposal to map the fields of views that the Hubble Space Telescope has seen is that Mercator projections were used to navigate travel to a point. Faced with the question of how our earth interacts with other planets, galaxies, galaxy clusters, superclusters, asteroids and other objects in its travel around the universe, solar system and galaxy, a map which sees us as navigating our path through the universe is a useful image.
The Mercator Projection is a useful image or story then to assist with navigation, but as this article points out does not accurately reflect the size of areas on earth or on space. It should be said that Mercator had a similar focus to me later in his life. He focused on ideas about the development of everything..I vaguely recall from reading just now at the Univeristy of Chicago Regenstein Library.
James Timothy Struck
— Preceding unsigned comment added by 128.135.96.228 (talk) 02:58, 23 January 2006 (UTC) (moved from the main page)
That rv might need explanation
Regarding this edit of mine: formula did look wrong, but not because of erroneous input – "My preferences/[Math] (•) HTML if very simple or else PNG" was at fault. —Preceding unsigned comment added by Saimhe (talk • contribs) 2006-09-28T00:09:21
Image about relationship between latitude and position on map
I think the image that relates latitude on globe to vertical position on map could be improved in two ways.
1. Make it show the complete graph. Neither latitude or vertical position on map are defined to infinity; both are limited. The current image conveys the idea that vertical position on map approaches a limit as latitude tends towards infinity.
2. Change the distance between "ticks" on the vertical axis so that the graph reaches +90 and -90 degrees, instead of current half-pi. Since in navigation, degrees are practicaly ubiquitous, it makes little sense to me to use radians here.
I'd be grateful if someone who has software that can make that kind of proffesional looking graph would deal with this. --85.224.196.223 23:02, 16 November 2006 (UTC)
Why the vertical distortion?
I can see why at the polar regions there would be a horizontal distortion, but why is there also a vertical one? Ajuk 12:30, 1 May 2007 (UTC)
- Because the scale changes for both. It's a conformal projection – it preserves (small scale) shape. So if the horizontal scale has to change, as of course it has to, then the vertical scale changes by the same amount. Paul Koning 15:52, 1 May 2007 (UTC)
Is there a projection that doesn't have the horizontal distortion? Ajuk 21:30, 6 May 2007 (UTC)
- No. All flat maps are distorted; only a globe has an undistorted picture. With maps, your only choice is what distortion you choose (or tolerate), given the purpose of the map. For example, on a Mercator projection, headings are true. On a gnomonic projection, great circles are straight lines. Pick your goal, and deal with whatever distortion that produces. Paul Koning 00:41, 7 May 2007 (UTC)
- Sorry my mistake, I know that. Is there a map that doesn't have the vertical distortion, just the horizontal? Ajuk 20:17, 7 May 2007 (UTC)
- Equirectangular projection, also known as Plate carrée projection. Paul Koning 20:26, 7 May 2007 (UTC)
- Sorry my mistake, I know that. Is there a map that doesn't have the vertical distortion, just the horizontal? Ajuk 20:17, 7 May 2007 (UTC)
Scale
This statement doesn't seem to me to be accurate: "Being a conformal projection, the linear scale does not vary with direction and the angles are preserved around all locations." As I understand it, the only lines of constant scale on a Mercator projection are the parallels. Any line which isn't parallel to the equator varies in scale (the representative fraction gets larger as the latitude gets higher). If I'm missing something, is there a clear way to explain what is meant by "scale does not vary with direction"? - Justin (Authalic) 22:22, 20 February 2007 (UTC)
- The statement means that scale near any one point is the same in all directions. As you said, it's true (indeed, it has to be true for every flat map projection) that scale is not constant in all directions over any signficant distance.
- Consider a counterexample: the plate carree projection. Its horizontal scale changes as you move away from the equator but its vertical scale doesn't. So at 60 degrees latitute, the horizontal scale is twice the vertical scale – which means that shapes are distorted (stretched horizontally by a factor of 2). In the Mercator projection, and in any other conformal projection, the horizontal and vertical scales change by the same amount as you move around the map. Paul Koning 14:39, 30 May 2007 (UTC)
Accessibility
It should be noted that the sections "Derivation of the projection" and "Mathematics of the projection" have no value to the majority of readers because there is little to no clear explanation other than the mathematic equations. This is a huge problem across Wikipedia. While the equations are surely important, we mustn't use them as the only point of reference when explaining the concepts (in most cases). It seems as though it would not be hard to put the concept in black & white terms. I'd do it, but I don't understand the math in this case.-DMCer (talk) 19:38, 24 November 2007 (UTC)
- What sort of other points would you add? The defining property of the Mercator projection is that it is conformal; the math follows from that. The intro paragraph states what Mercator is all about (straight rhumb lines, in particular). Mercator is not a geometric projection – as indeed is true for most map projections. So you can't talk about it informally in geometric terms, as you might do for, say, gnomonic projection. Paul Koning (talk) 16:33, 27 November 2007 (UTC)
Uses
This section has the sentence Despite its relative scale distortions, the Mercator is well-suited as an interactive world map that can be panned and zoomed seamlessly to local maps. Now near as I can tell, there is nothing remarkable about the Mercator here, as just about any cylindrical projection would do this. mdf (talk) 13:49, 9 March 2008 (UTC)
- I think I understand the sentence. Because the projection is conformal (scale does not vary with direction), angular distortions are zero everywhere. Then, and for large zooming (local representations) the grid of meridians and parallels will always look "square", independently of the geographic position. That does not happen with non-conformal cylindrical projections, as the ratio between the scales along meridians and parallels varies with location. It has never crossed my mind this kind of property, as the Mercator projection is a terrible solution for depicting the whole world (still, it was used a lot for that purpose, in the past) – Alvesgaspar (talk) 16:57, 9 March 2008 (UTC)
Formula
Sir, I have longitude and latitude value and just find co-ordinates value in two dimension (x,y). I get one formula but i do not get h or ln value.
— Preceding unsigned comment added by 59.95.195.51 (talk) 06:09, 28 December 2005 (UTC)
formula available in this hyperlink "http://en.wikipedia.org/wiki/Mercator_Projection" — Preceding unsigned comment added by 59.95.195.51 (talk) 06:09, 28 December 2005 (UTC)
The formula in the article is not valid with the map displayed in the article ( http://fr.wikipedia.org/wiki/Image:Mercator-proj.jpg ) because the formula must be adjusted with the h and w of the picture (679×724). pixel_x = w*((λ+180) / 360).
pixel_y = h / 2-LN(TAN( (pi / 4) + RADIANS(φ) / 2))*ratio
with
ratio = w / (pi*2) = 108 w = width of the picture (724) h = height of the picture (679) picture must be centred on 0,0 217.195.19.145 (talk) 08:20, 19 June 2008 (UTC)
Poles on a Mercator map
A Mercator map can never fully show the polar areas. – It is not quite true. In the Russian Arctic Fleet a special alternative geographical coordinate system is used. Its "equator" coincides with the Greenwich meridian, and its "prime meridian" coincides with the 90° meridians. A Mercator map in this coordinate system does show the polar areas and the North Pole very well. —Preceding unsigned comment added by Hellerick (talk • contribs) 15:17, 25 June 2008 (UTC)
Oh, sorry I found it, it's called Transverse Mercator projection. But still, it's a Mercator projection, isn't it? – Hellerick (talk) 15:20, 25 June 2008 (UTC)
- "Mercator" by itself means the equatorial form, as opposed to transverse or oblique Mercator. Maybe that could be mentioned early on. Come to think of it, this seems to be true for cylindrical projections in general. Paul Koning (talk) 00:29, 26 August 2008 (UTC)
su song map
The Su Song star map picture is labeled as being Mercator projection. How is that supported? It looks like a cylindrical projection of some sort, all right, but it's not obvious from inspection whether it's plate carree, Mercator, or something else. Paul Koning 16:09, 14 August 2007 (UTC)
If you open up the image, this is the description provided:
This is a star map for the celestial globe of Su Song (1020-1101), a Chinese scientist and mechanical engineer of the Song Dynasty (960-1279). It was first published in the year 1092, in Su's book known as the Xin Yi Xiang Fa Yao (Wade-Giles: Hsin Yi Hsiang Fa Yao). On this star map there are 14 xiu (lunar mansions) on Mercator's projection. The equator is represented by the horizontal straight line running through the star chart, while the ecliptic curves above it. Note the unequal breadth of the lunar mansions on the map.
Su Song's star maps had the hour circles between the xiu (lunar mansions) forming the astronomical meridians, with stars marked in quasi-orthomorphic cylindrical projection on each side of the equator, and thus was in accordance to their north polar distances. Not until the work of Gerard Mercator in 1569 was a celestial map of this projection created in the Western world (Needham, Volume 4, Part 3, 569).
This picture appears on page 277 of Joseph Needham's book Science and Civilization in China: Volume 3, Mathematics and the Sciences of the Heavens and the Earth.
I hope this clears up anything about the image.--Pericles of AthensTalk 01:21, 11 February 2008 (UTC)
- No, it doesn't. I saw the description but it gives good reason to argue that the chart is not a Mercator projection. For one thing, the description says "quasi-orthomorphic". I'm not sure what that is but it doesn't sound like "Mercator". Furthermore, the article on Su Song claims that the map show the pole star. So by definition the projection cannot be Mercator. Paul Koning (talk) 15:32, 11 February 2008 (UTC)
- From the map file : Su Song's star maps had the hour circles between the xiu (lunar mansions) forming the astronomical meridians, with stars marked in quasi-orthomorphic cylindrical projection on each side of the equator, and thus was in accordance to their north polar distances. Not until the work of Gerard Mercator in 1569 was a celestial map of this projection created in the Western world (Needham, Volume 4, Part 3, 569)
- These two properties are contraditory. Either the projection is "quasi-conformal" (i.e., quasi-Mercator) or the distances along the meridians are conserved. It is impossible to have both things. If the projection were indeed conformal then the scale along the meridians would grow with declination, becoming infinite at the celestial pole. – Alvesgaspar (talk) 16:23, 11 February 2008 (UTC)
I'll admit, I'm a layman when it comes to specifics in astronomy and cartography; let me present what Joseph Needham says in full and in his own writing:
. . .Lastly, the projection of Gerard Mercator in 1569 was a great advance, but he never knew that he was preceded by Su Song five centuries earlier in a celestial atlas, in which the hour circles between the hsiu (lunar mansions) formed the meridians, with the stars marked in quasi-orthomorphic cylindrical projection on each side of the equator according to their north polar distances (Needham, Science and Civilization in China, Volume 4, Part 3, 568–569.)
Ok, and in Needham's 3rd volume on page 278:
If the maps incorporated in the Hsin I Hsiang Fa Yao (New Description of an Armillary Clock) by Su Sung really date from the time when the book was written, they must be the oldest printed Chinese star-charts which we possess. Begun in 1088, it was finished in 1094, and the maps which it contain are remarkable in several ways. They are five in number, one of the north polar region, two cylindrical orthomorphic 'Mercator' projections of the regions of declination about 50° N and 60° S, and two polar projections, one of the northern hemisphere and one of the south. The space where the southern circumpolars should be is left blank. The drawing of stars is much more carefully done than on the MS map of rather more than a century earlier, but this also had the 'Mercator' projection...Close examination reveals that while the first of these maps accepts the pole-star of -350° as the position of the true pole, the fourth places this position half-way between Thien shu and our Polaris. This would suggest that Su Song must have taken advantage of the observations of his contemporary Shen Kua, already referred to.
I will try to scoop up more about this, but I think this is all Needham has to say, since right after this paragraph he switches topic.--Pericles of AthensTalk 06:52, 15 February 2008 (UTC)
Found some more; on page 208 of Needham's 3rd Volume of Science and Civilization in China, he says this after introducing Su Song's astronomical book and atlas:
The second chapter describes a celestial globe and includes star-maps in which the central palace (circumpolar region) and south polar region are planispheres, while the stars of the more equatorial-ecliptic regions are arranged on a cylindrical projection very similar to Mercator's.
More coming...--Pericles of AthensTalk 06:57, 15 February 2008 (UTC)
Needham talks of an earlier Tang Dynasty precedent of the Dunhuang map from the 8th century (Needham says the 10th century):
It will be remembered that already for the 10th century documentary evidence exists [footnote "h" here: Cf. pp. 264, 276 above regarding the Tunhuang manuscript star map] that a projection analogous to that of Mercator was used, the hsiu being represented as long rectangles centered on the equator and of course very distorted towards the poles.
Still looking for more...--Pericles of AthensTalk 07:04, 15 February 2008 (UTC)
On Islamic astronomy, Needham states on page 563 of Volume 3:
In comparing the scientific cartography of the Muslims with the Chinese, there are three chief maps to keep in mind. The first and most famous of these was the world map of Abu Abdallah al-Sharif al-Idrisi (1099 to 1166), made about 1150 for Roger II, the Norman king of Sicily, and often reproduced in modern works. This was fully in the Ptolemaic tradition, using nine parallels of latitude (climates) and eleven meridians of longitude, but arranged on a projection like Mercator's and making no attempt to allow for the earth's curvature.
Needham cites Kimble (I), p. 57 on this. Still looking for more...--Pericles of AthensTalk 07:09, 15 February 2008 (UTC)
- Unfortunately, these quotes aren't precise enough to tell us what we need to know. I suspect the authors quoted do not understand projections that well. The most that one can conclude from them is that these old charts are cylindrical projections. But Mercator is not any old cylindrical projection, it's cylindrical conformal. That means the scale changes in a specific way (the distortion going towards the poles changes in a specific way). All flat maps have distortion, so all cylindrical projections have distortion, but that doesn't make them Mercator. And earlier cylindrical projections do not deserve credit as anticipating Mercator because it is precisely that conformality property that is essential and valuable in the Mercator projection.
- Given an accurate image of one of these old maps and identifications of some of the stars, it would be possible to reverse-engineer the projection. I have been wondering if the Su Song map image in that article is good enough to do this; I haven't tried yet. (In this process one would have to account for precession of the equinox, of course.)
- One thing I'm still wondering about, and the imprecise terminology of the authors quoted doesn't help dispel the uncertainty, is whether these old charts are projections at all. In other words, are they more than just sketches – can you take the coordinates of a star on the chart and run it through a reverse projection transform – any single fixed transform – to arrive at the correct position of that star in the sky? If yes then it is a chart; if not then it's only a sketch. The quotes say the former, but their wording doesn't convince me that the authors actually fully understand the difference.
- Paul Koning (talk) 22:40, 15 February 2008 (UTC)
- According to this article <http://books.google.com/books?id=gQYscrT0fgQC&pg=PA712&lpg=PA712> the projection was a cylindrical equidistant. Needham was, alas, wrong about it being the Mercator projection. Ian Ridpath. 2009 January 21. 79.66.80.248 (talk) 22:42, 21 January 2009 (UTC)
Possible modifications to the Mercator page.
I am contemplating a number of revisions to the Mercator page and I would like to have some feedback before venturing too far, and possibly (as a newcomer to Wiki) stepping on a toe too many. If you wish to assess my interest in this subject you may wish to have a look at the following substantial (and mathematical) article on The Mercator Projections.
The amendments I would envisage are, in no particular order, as follows:
1 Separate mathematics and history completely.
2 Introduction explaining that "the" Mercator projection is one of many Mercator projections, classified by a projection which is normal/transverse/oblique on a surface which is sphere/ellipsoid and finally is unmodified/modified. Start the article with a precise and unambiguous statement.
3 Simplify the derivation of the projection formulae by supplying figures comparing infinitesimal triangles. This gives the same equations without an appeal to the black box of conformality and the Cauchy-Riemann equations. This still leaves the same integral to be solved.
4 A clearer explanation and derivation of the point scale factor by using the infinitesimal elements. This would show isotropy of scale explicitly. It would also lead to the Tissot illustration.
5 Discuss scale distortion but point out that the normal Mercator is good for accurate maps near the equator. This would point the way to the choice of a transverse variant applied to any meridian to provide accurate coverage of the whole globe. (This discussion would go to the Transverse Mercator page which also require updating).
6 Possibly remove the Chinese maps. "Similarity" is hardly grounds for inclusion. After all Ptolemy's map is just as 'similar', even more so, but it is an equidistant projection (Plate Carree).
7 Remove the plot of latitude against y. A plot of scale factor against latitude would be better.
8 Discussion the modified (or secant) projections in relation to extending the zone of accuracy.
9 State without proof the projection formulae on the ellipsoid.
10 Tidy up the "Uses" section.
And possibly more.
Peter Mercator (talk) 22:53, 3 April 2009 (UTC)
- Wow, from your username I can see you are a fan of Mercator projection! Agree with 1, 5, 6, 7, 9, 10. Point 2: "the Mercator projection" is the normal aspect applied to a spheric model, that's what Gerhard M. proposed more than 400 years ago. Other authors (including Lambert, Gauss, etc.) addressed other modalities. But yes, I agree that all this should be briefly explained. Points 3 and 4: is it really necessary to derive the formulae? Before presenting the equations, I would just say that the requirement is that the scale along meridians should equal the scale along parallels, which is proportional to the secant of the latitude. Bottom line: make it simple and with little math. That will make justice to the projection, whose concept is really simple (unlike the formulae). Go ahead and good luck! -- Alvesgaspar (talk) 23:28, 3 April 2009 (UTC)
- Thanks for your comments: changes will appear slowly now that the weather is improving. Just one comment. Conformality (angle preservation) demands equality of local vertical and horizontal scales -- and vice-versa. Since the whole point of Mercator's chart was to plot rhumb lines to straight lines, conserving bearing, I believe it is more natural to start from conformality. In general I believe higher mathematics doesn't have a place on Wiki pages so the derivation has to be as simple as possible. -- Peter Mercator (talk) 09:00, 6 April 2009 (UTC)
- There are two different approaches to the derivation and maybe we should consider both. The modern theoretical one, starting from the conformality conditions and ending in the formulae, and the historical (graphical) one, used by Mercator. Concerning the first, and since we are dealing with a normal cylindrical projection where meridians and parallels are assumed orthogonal, it is enough to make the scales along meridians and parallels equal (m=p) and proceed from there. As for the second approach, it is now known (see the excellent Hollander book: [1]) that the projection was constructed graphically, by transferring one or more loxodromes from a globe to a plane, and then adjusting the position of the parallels to make them straight (I made a graph of it and send it to you, if you want). Please count on me if you want some help or opinion. -- Alvesgaspar (talk) 13:21, 6 April 2009 (UTC)
- Thanks for the reference to the book by Hollander: I shall have a look at it. Similar comments can be found in the book by Mark Monmonier entitled "Rhumb Lines and Map Wars". It too is an excellent text. Yes, please email a copy of your graphs. -- Peter Mercator (talk) 10:39, 10 April 2009 (UTC)
- There are two different approaches to the derivation and maybe we should consider both. The modern theoretical one, starting from the conformality conditions and ending in the formulae, and the historical (graphical) one, used by Mercator. Concerning the first, and since we are dealing with a normal cylindrical projection where meridians and parallels are assumed orthogonal, it is enough to make the scales along meridians and parallels equal (m=p) and proceed from there. As for the second approach, it is now known (see the excellent Hollander book: [1]) that the projection was constructed graphically, by transferring one or more loxodromes from a globe to a plane, and then adjusting the position of the parallels to make them straight (I made a graph of it and send it to you, if you want). Please count on me if you want some help or opinion. -- Alvesgaspar (talk) 13:21, 6 April 2009 (UTC)
- Thanks for your comments: changes will appear slowly now that the weather is improving. Just one comment. Conformality (angle preservation) demands equality of local vertical and horizontal scales -- and vice-versa. Since the whole point of Mercator's chart was to plot rhumb lines to straight lines, conserving bearing, I believe it is more natural to start from conformality. In general I believe higher mathematics doesn't have a place on Wiki pages so the derivation has to be as simple as possible. -- Peter Mercator (talk) 09:00, 6 April 2009 (UTC)
- I like it. On #6 (remove the Chinese map) -- agree strongly. It's not clear it's a map at all, or if it is, what its projection is. On "Uses" that indeed needs work. For one thing, it should talk about uses of the projection, rather than spending most of the words talking about the PC aspects of projections. It may be that the projections debate is worth mentioning, but if so, it should be in a section dedicated to that purpose. It would also be good to make such a section more neutral, which at the moment arguably is not the case. Lastly, I like the idea of discussing both the mathematical basis (conformance, therefore matching scales, therefore secants), and the graphical one. I hadn't thought about the graphical one, but it fits that this would be how the original work was done all those centuries ago. Paul Koning (talk) 00:58, 10 April 2009 (UTC)
- I just noticed a comment about the Chinese map that I missed before, pointing to an article about its projection. Ok, it indeed is a projection, but it's plate carree. So it might make a fine illustration for that article, but it definitely does not belong here. Paul Koning (talk) 01:06, 10 April 2009 (UTC)
- By graphical derivation I meant a derivation of the differential equation for y by appealing to the figures shown in the page {{scale (map)|scale (map)]] and demanding that angles are preserved. Mercator's method, in so far as we can guess, is along the lines mentioned in the previous comment by alvesgaspar. I will start on some of these edits once I have finished a new version of scale (map) (on which I would appreciate comments). -- Peter Mercator (talk) 10:29, 10 April 2009 (UTC)
- I found the article really good. Re the bit about Google maps and the limits of latitude (phi). I think there is a typo and +-pi in the text and in the following equation should be +- pi/2. Furthermore I did not follow the argument for a theoretical limit of latitude to +-85deg. I think the projection is theoretically valid almost to +-90deg where Y becomes +- infinity, although clearly it is of no practical use at high latitudes where the variation of scale with latitude will become excessive even over a small charted area.86.150.154.44 (talk) 21:27, 25 June 2009 (UTC)
- I just noticed a comment about the Chinese map that I missed before, pointing to an article about its projection. Ok, it indeed is a projection, but it's plate carree. So it might make a fine illustration for that article, but it definitely does not belong here. Paul Koning (talk) 01:06, 10 April 2009 (UTC)
Main Picture
I'm putting forth the suggestion that the main example of the projection used at the top of the page should be changed to something more modern. While the historical map is endearing, it makes it much harder for the reader to quickly absorb the distortions instituted by the mercator projecition. — Preceding unsigned comment added by 128.112.87.60 (talk) 06:12, 18 November 2006 (UTC)
- You might use World Map flat Mercator.png. I uploaded it together with World Map flat Gall-Peters.png because I wanted some clean maps. Nutzelfutz (talk) 18:33, 17 May 2008 (UTC)
- I like the old map, but I wish it were at a higher resolution. It looks like a really beautiful piece of work, but all the detail is lost at this resolution, especially in Europe. It might be best to place a modern example of the map at the beginning of the article, and the old map could go in the historical details section. Gary (talk) 05:30, 1 August 2009 (UTC)
A Nice Image?
I noticed that most of the other (main) projection pages have a nice (fairly hi-res) geographic/photographic image available. (See Gall-Peters, etc) But this page does not. It would be nice to add one. It actually looks like most of the other images are re-projections of the same image. —Preceding unsigned comment added by 86.134.119.166 (talk) 09:04, 12 October 2009 (UTC)
India Higher than Finland?
That Comment is confusing, Finland is clearly higher on the Mercator Projection. And under no projection can think of India being higher on the map. unless it doesn't mean farther away from the equator, which if that is the case then the sentence is still confusing in that I don't understand what it means. Arkkeeper (talk) 18:39, 29 May 2008 (UTC)
- taller, not higher - i.e. the distance from north Finland to south Finland appears similar to the distance from north India to south India. HTH --Streapadair (talk) 20:24, 28 February 2010 (UTC)
The "Google projection"
It's probably worth noting that the Google projection (EPSG:3875, IIRC) is modelled on the Mercator projection, but has a twist that often catches people out. It uses latitude and longitude referenced to the WGS84 ellipsoid, but projects as if they were referenced to the sphere. There are a scattering of articles on the Web that explain this, but I think the important thing is to make clear that it's a slightly different projection, and will thus give different renderings compared to a true Mercator. --Streapadair (talk) 20:30, 28 February 2010 (UTC)
- Is it really notable the projection that google uses? Shouldn't it just go into the google maps article? —Preceding unsigned comment added by Infringement153 (talk • contribs) 08:00, 19 June 2010 (UTC)
- It would be normal to note variations of a subject in the main article on the subject. In particular, this device Google uses is used not only by Google Maps; it is used by all the similar services online. It is also usually mistakenly identified as Mercator. Strebe (talk) 01:24, 20 June 2010 (UTC)
"Cartographers agree that it is not suited to large area maps"
Strebe wrote: "The projection is defined over a wide area but cropped to small areas in useful maps." On what basis can you say this? Surely for navigational purposes, the wider the area, the more useful the projection's "unique properties" become? – Smyth\talk 12:33, 16 July 2010 (UTC)
- Rhumbs are not efficient to travel long distances along because, in general, the longer the rhumb, the further it deviates from a great circle. In practice on long trips, the great circle route is plotted, and then it is broken up into segments connected by rhumbs to approximate the route. This gives a good compromise between the route of shortest distance and the route that is easiest to navigate.
- Navigational charts are not “wide-area”; they cannot be while still showing anything useful about sailing conditions. One could use a small-scale Mercator chart for roughing in a long-distance itinerary if one had means to draw great-circle routes on it, but I cannot say whether that is or was ever done commonly. I have never seen any evidence of it, such as plots on old maps or reference to the practice. Still, I agree the statement as given is too sweeping. Strebe (talk) 18:01, 16 July 2010 (UTC)
- Before computers, orthodromes (great circle tracks) were drawn on small-scale Mercator charts with the help of a gnomonic projection. The tracks were first drawn on the gnomonic, where they are represented by straight segments, and then graphically transferred to the Mercator chart. The alternative method was analytical. The spherical navigational triangle was first solved to calculate a sample of points on the great circle track (using fastidious logarithmical calculations or tables), and then transferred to the Mercator projection. Anyway, the use of orthodromes in marine navigation is very uncommon and usually restricted to long oceanic tracks. That is not the case in aerial navigation, where they are commonly used. -- Alvesgaspar (talk) 22:51, 16 July 2010 (UTC)
Thanks. – Smyth\talk 21:36, 16 July 2010 (UTC)
Replace low-contrast images

I will be replacing images on the various map projection pages. Presently many are on a satellite composite image from NASA that, while realistic, poorly demonstrates the projections because of dark color and low contrast. I have created a stylization of the same data with much brighter water areas and a light graticule to contrast. See the thumbnail of the example from another article. Some images on some pages are acceptable but differ stylistically from most articles; I will replace these also.
The images will be high resolution and antialiased, with 15° graticules for world projections, red, translucent equator, red tropics, and blue polar circles.
Please discuss agreement or objections over here (not this page). I intend to start these replacements on 13 August. Thank you. Strebe (talk) 22:44, 6 August 2011 (UTC)
- Please do replace the images provided by Mdf in this article and many other articles related to map projections. They are quite inadequate, failing to show clearly the graticule, principal circles and even the boundary of nonrectangular projections (eg Mollweide projection). Peter Mercator (talk) 20:38, 10 August 2011 (UTC)
- The picture here on the right is much much better than that one in the article. --Chricho ∀ (talk) 11:44, 17 August 2011 (UTC)
Angle notation
It would be helpful if the angle notation was illustrated somewhere. — Preceding unsigned comment added by Neffk (talk • contribs) 20:03, 30 August 2011 (UTC)
- “Angle notation”, meaning…? Strebe (talk) 04:39, 31 August 2011 (UTC)
Square Mercator
(This discussion is copied from the Talk:Aitoff_projection page.)
Why did you upload a cut off version for the Mercator projection? That one you posted on the discussion page is much better because it demonstrates the stretching. --Chricho ∀ (talk) 11:48, 17 August 2011 (UTC)
- Hi, Chricho. Thanks for the comments. I don’t follow your question. The Mercator has to be cut off somewhere because the full map is infinite in extent. Which discussion page do you refer to? Strebe (talk) 00:27, 18 August 2011 (UTC)
- Hi, your proposed image at the discussion page is simply a little bit less cut off, there the stretch is more obvious. --Chricho ∀ (talk) 22:55, 10 September 2011 (UTC)
- Which discussion page do you mean, please? Strebe (talk) 01:58, 11 September 2011 (UTC)
- Looks good, Strebe. Thank you.
- I'm pretty sure Chricho is alluding to the Talk:Mercator projection#Replace low-contrast images section. I agree with Chricho that the "square" image is a better illustration for the Mercator article than the "slightly more clipped" image, because it illustrates the stretching better. I understand that, mathematically, the Mercator projection is infinitely long north-south, so we have to clip it somewhere. I think Chrico is alluding to the following two images: --DavidCary (talk) 17:50, 4 December 2011 (UTC)


- Ah. Got it. Thanks, and thanks for the comments. I’ll consider the taller image in the context of the Mercator article. It’s very uncommon for the Mercator to extend that far. Google Maps does it for its tiling system, but Google Maps was not developed as a display world map. Meanwhile printed maps never show it like that, so to do so would be “ahistorical” and not representative. I understand that the square representation makes the vertical stretching even more obvious, but the vertical stretching already seems obvious to me, and it’s not clear to me that making it more obvious is more important than showing the map in a way more representative of how it is normally used. I’d like more discussion about this if I am to make the change. Strebe (talk) 23:33, 4 December 2011 (UTC)
- Yes, DavidCary is right about interpreting my statement. ;) Sorry, missed the discussion. --Chricho ∀ (talk) 21:27, 5 December 2011 (UTC)
- The Mercator projection#Uses section implies that the "square Mercator" arrangement is pretty common for online maps.
- Would it be possible to make two images for the Mercator article, one with a "square Mercator" projection and another one with a "typical printed map" arrangement? --DavidCary (talk) 06:04, 17 December 2011 (UTC)
- I have uploaded an image here. To repeat, I do not think the fact that Google and other Web map producers use the square form is very useful to a reader of this article. Google Maps is not intended as a world map. The square format is merely an engineering technique for tiling. I don’t think adding the image to the article will improve it. It will just get in the way. Strebe (talk) 08:13, 17 December 2011 (UTC)
- Would it be possible to make two images for the Mercator article, one with a "square Mercator" projection and another one with a "typical printed map" arrangement? --DavidCary (talk) 06:04, 17 December 2011 (UTC)
Controversy section
Most of the content in the Controversy section does not relate to controversy. I see three topics in there: limitations, applications and controversy. My inclination would be to merge all of this into the main body of the text. Wikipedia editors do love our controversy sections, though. Ronstew (talk) 15:55, 23 January 2008 (UTC)
- I agree with you. After all there is no real controversy over the Mercator projection, as it is just a bunch of mathematical formulas. Perhaps it just needs a better name. But I can't think of one right now.151.200.90.2 (talk) 00:12, 26 February 2008 (UTC)
- There does seem to be some controversy listed in that section, by Peters and others. Some of that is just noisemaking to promote a different projection, which simply trades one set of distortions for another, or equally simplistic noises objecting to a whole class of projections (cylindrical ones). At least the section does begin with the statement that all projections distort; you only get to choose your trade-offs. Paul Koning (talk) 19:18, 26 February 2008 (UTC)
- I believe this was quite a controversial thing to be done. First of all, I agree that it's all just a bunch of mathematical equations. But you can't downplay the importance of the fact that in the United States of America and other anglo-saxon countries this specific equation is frequently selected for the confection of world maps, and it is not because of the nice characteristics of the equation, but just because the area of these imperialist countries look larger and stronger then the other countries (for example, then Brazil). While in the world we have millions of children growing up in the northern north hemisphere seeing this navigational map as a "fair" representation of the world, you can't just come here and pretend it is not happening, and be all Mr. Scientist. The controversy section is not about the equations, is about how this specific equation here is misused in USA as a rhetoric tool for imperialist policies. It's a geographical sophism.
- Although all projections have their distortions, there is no other projection that would qualify to receive a controversy section, simply because they are not misused as much as Mercator. The controversy section is about this cultural aspect of the Mercator projection, the controversial way it is frequently used, and not about it being "inherently evil".
- If you read the "uses" section as it is now, this title seems very naïve if not plain wrong. Why would a "uses" section of any projection start talking about the relative size of the countries depicted? And why would a "uses" section talk about institutions refusing to use it? This section should only say that "the map has nice characteristics that aid in some calculations during navigations". All the rest, regarding the controversial use of it in atlases and other places, and the Peters thing, and all the fight to stop its use in schools and any other place if not in the desk of someone doing calculations during navigations, must have at least a sub-section. There must be a "uses" and a "misuses" section. "uses" and "abuses". In fact, the best thing would be to have a separate article for this subject, and leave the page about the projection as clean and mathematical as possible (with a link to the controversy article, of course). – NIC1138 (talk) 16:29, 20 March 2008 (UTC)
- Agree and will support your changes. The problem is the "Peters religion" is still alive - Alvesgaspar (talk) 17:17, 20 March 2008 (UTC)
- I don't think it's a good idea to get into political discussions when the subject is mathematics. Paul Koning (talk) 18:44, 20 March 2008 (UTC)
- There does seem to be some controversy listed in that section, by Peters and others. Some of that is just noisemaking to promote a different projection, which simply trades one set of distortions for another, or equally simplistic noises objecting to a whole class of projections (cylindrical ones). At least the section does begin with the statement that all projections distort; you only get to choose your trade-offs. Paul Koning (talk) 19:18, 26 February 2008 (UTC)
The subject is not merely mathematics. Mercator's projection itself was born out of critiques of earlier conical Ptolemaic projections and plane charts used by mariners - This is from Dennis Wood's "The Power of Maps" (2010):
- Ultimately, Mercator's critique of the portolanos and the Ptolemaic conics would take the form of his eponymous "projection," a spatial frame that was no sooner published than it became the subject of critiques that continue into the present.58 Among those first objecting to the projection were the mariners for whom it was expressly designed but who, thanks to its poleward increase in scale, found it hard to understand; and it is this characteristic that has sustained the most extended critique. In 1772 in a veritable counter-projection, J. H. Lambert shrank what Mercator had stretched to maintain areal proportions instead of compass bear- ing, and his cylindrical equal-area projection became the first of a number of recti- linear projections reacting against the Mercatorial world. Among its progeny were projections created byJames Gall in 1855 and Arno Peters in 1967.
- Gall attacked precisely Mercator's commitment to navigators, writing in 1855 that "Mercator's projection sacrifices form, polar distance, and proportionate area, to obtain accurate orientation for the navigator; whereas to the geographer, form, polar distance, and proportion of area are more important than orientation," which, while reversing it, perfectly recalls Mercator's critique of the Ptolemaic con- ics popularized by the scholastic geographers.59 Peters, on the other hand, critiqued the Mercator for being "the embodiment of Europe's geographical conception of the world in an age of colonialism." Though, in common with Lambert and Gall, Peters was not a cartographer (Lambert was a physicist and mathematician, Gall a clergyman, Peters an historian), Peters had no hesitation about critiquing cartogra- phy for clinging to a "closed body of teaching which has developed into a myth."6o Embattled cartographers defended themselves by condemning all rectilinear world projections-an hysterical overreaction that reflected the seriousness of the wound Peters's critique had inflicted-a laughable position, were it not so sustained, that continues into the present.
These go well beyond the typical eurocentric, post-colonial objections - for mathematicians to excise those issues, pretending that there's a reducibility to the topic that projection is merely an issue of formulas and equations is incredibly short-sighted. But that was years ago, so I'll just leave this here for someone who knows more about cartography and is less discipline-biased. Nathanaelbassett (talk) 00:41, 6 March 2012 (UTC)
New Mercator page. Help needed.
Please look at the new page Mercator 1569 world map and its Talk page. Peter Mercator (talk) 21:53, 28 March 2012 (UTC)
- …Wow. Strebe (talk) 01:34, 29 March 2012 (UTC)
Revised Mathematics section
A long time ago I proposed a list of "Possible modifications to the Mercator page" higher up this page. Some have already been implemented. I have now rewritten (and extended) the Mathematics section and moved it to the end of the article in the belief that it should not interrupt non-technical readers. The principal changes are
- 1 The removal of the derivation based on the Cauchy-Riemann equations. That was accessible only to fairly sophisticated mathematical readers. The new derivation uses simple geometrical arguments.
- 2 Added a discussion of the problem of interpreting ruler measurements on the map. I think this is important.
- 3 Removed expressions for the differentials.
- 4 Simplified ellipsoid case (and corrected error in the scale factor). I don't think we should dive into details of the geometry of the ellipsoid.
- 5 Improved figures and graphs.
Revision of the mathematics is perhaps the easiest part. I have also been working on revisions of the other sections and the current draft can be found here. I'm reasonably content on the background and history sections but some sections, particularly the section on 'Properties' (meant for a non-technical reader) needs to be re-written. I would be very grateful for comments from expert and (especially) general watchers--on both present and proposed changes.
Peter Mercator (talk) 10:23, 9 July 2012 (UTC)
- The Properties section (missing from your sandbox) might replace the speculation over Mercator’s method with Joaquim Alves Gaspar’s analysis. Strebe (talk) 20:04, 12 July 2012 (UTC)
2012.10.19 edits
With thanks to Clan-destine for the effort, I reverted his or her edits from today. I found
The spherical approximation of Earth with radius a can be modelled by a smaller sphere of radius R, called the globe in this section. It is necessary to restrict calculations to this smaller globe scale so that the resulting projection will fit onto a map, page, or computer screen.
to be too verbose and potentially misleading. I agree the reason for R needs to be stated, so I added an explanation for that. Necessary to restrict is not true; the map may simply be an extremely tiny portion of the earth.
“Origin” is preferable to “the origin” in Cartesian coordinates on the map with the origin on the equator because “the” adds vagueness. The? Which? The globe’s? The map’s? “Its” is permissible in place of “the”, but unnecessary. Semi-arbitrary is contrived; the explanation for why it is not completely arbitrary is given next in any case.
An example of a geometrical projection is that of light rays onto a screen, is not correct because light rays are not an example; they are a description of the principle. They may be an example of the principle, but in the context of the sentence I deleted, “example” will likely confuse the reader into thinking there are other kinds of projections that are not reasonably demonstrated by light rays on the screen. Strebe (talk) 21:29, 19 October 2012 (UTC)
- Without the explicit explanation of the relationship between the spherical Earth and the globe (which you refer to as "verbose"), I think you risk confusion by non-cartographers. You have basically changed the article back to its previously unclear language. When first attempting to use these calculations, I saw no explicit differentiation between R and a and thought the two were synonymous. If you want this article to be clear and accessible to non-experts, I suggest you use my original "verbose" wording. Clan-destine (talk) 22:35, 19 October 2012 (UTC)
- You also indiscriminately removed my correction of typos which were not mentioned in your rebuttal to my original edits. For future revisions, could you please actually edit the article on a case-by-case basis instead of indiscriminately removing all of my edits? Clan-destine (talk) 22:47, 19 October 2012 (UTC)
notation
Don't use bad notation: . Kkddkkdd (talk) 16:02, 2 September 2013 (UTC)
- Suggestions for improvement or at least an explanation of your objection would be helpful. Strebe (talk) 23:47, 2 September 2013 (UTC)
Lead
What exactly is the reason for reverting an edit trying to make a sentence on the lead more explicit? Reasons such as "the detail is unnecessary" holds no water, because Wikipedia is not here to attend to what you think is necessary in an article or not! According to WP:POV, " do not remove sourced information from the encyclopedia solely on the grounds that it seems biased. Instead, try to rewrite the passage or section to achieve a more neutral tone. Biased information can usually be balanced with material cited to other sources to produce a more neutral perspective, so such problems should be fixed when possible through the normal editing process. Remove material only where you have a good reason to believe it misinforms or misleads readers in ways that cannot be addressed by rewriting the passage.". That's what I did with the lead, but Strebe and Alvesgaspar are busy disrupting wikipedia for no just reasons! Now is the time to say exactly why you think an explicit sentence is not necessary for the lead. If you can't give a reason based on Wikipedia's guideline, but your point of views, the edit will be restored, and you maybe blocked if you continue to disrupt wikipedia.--Jamie Tubers (talk) 15:44, 24 September 2015 (UTC)
- Those phrasing and examples should be avoided for two reasons: firstly, because they are mathematically imprecise and prone to confusion: Europe and North America appear larger relative to Africa in the same way that Asia and South America appear larger relative to Africa and Europe, and Central Africa appears smaller relative to northern and southern Africa; secondly because the issue was politically charged by the unfortunate Arno Peters controversy, when the German historian claimed that the Mercator projection was Eurocentric. Thus better keep a simple, clear and neutral statement about the extreme area distortions of the Mercator projection. On a quick note, please refrain from making threats and negative comments on the reasons of users you know nothing about. Alvesgaspar (talk) 16:41, 24 September 2015 (UTC)
- This is the reason why the WP:POV states that you shouldn't revert appropriate edits, but instead try to improve them. That's why Wikipedia is a community and not a one-man show; you are suppose to improve contributions, not delete them. You could've easily made the article more precise by introducing few adjectives and make a sentence like: "northern Europe and North America appear quite larger relative to land masses near the equator (like Central Africa and Central America)". Removing my edits, which are not wrong, and giving reasons such as "unnecessary detail in the lead" is just not acceptable. Same way, leaving the article's lead unneutral and unbalanced, by giving relatively unknown examples for the landmasses at the far end of the equator, whilst giving no example at all for land masses close to the equator is not acceptable. It's either we restore the modified version of the contribution I made, or just state entirely what you just explained (about Europe and North America appear larger relative to Africa and central America, Asia and South America appear larger relative to Africa and Europe, and Central Africa appears smaller relative to northern and southern Africa). The lead as it stands is short and can accommodate a paragraph on this.
- Per MOS:LEAD, "The lead should be able to stand alone as a concise overview. It should define the topic, establish context, explain why the topic is notable, and summarize the most important points [...] the lead should not "tease" the reader by hinting at content that follows. Instead, the lead should be written in a clear, accessible style with a neutral point of view; it should ideally contain no more than four well-composed paragraphs". Saying exactly what differentiates this map from the real world and other types of map is a key point into what the article is about and shouldn't be left out or shabbily described in the lead. On a side note; I never issued a threat to you, I only gave a notice on your talk page. Neither did I make negative comments about you.--Jamie Tubers (talk) 22:46, 24 September 2015 (UTC)
- I appreciate your efforts, Jamie Tubers. This is in support of Alvesgaspar comments. Furthermore, WP:POV is irrelevant here. Insertion of details is certainly open to editor debate and consensus; articles are not indiscriminate accretions of facts. And lastly yes, the “notices” you left on talk pages are threats. They have no legitimacy. Strebe (talk) 23:40, 24 September 2015 (UTC)
- WP:POV seems relevant, because until now, both of you were reverting my edits; leaving summaries like "too detailed for the lead", "not necessary detail" etc. Those are baseless personal opinions! Anyway,what do you suggest to be done to the unbalanced phrase in the lead? By the way, you think bringing to the lead, a summary of an information already in the body of the article to be "indiscriminate accretions of facts"? Wao!. The notices were meant to be civil, please do not take it otherwise.--Jamie Tubers (talk) 23:56, 24 September 2015 (UTC)
- Since I (and presumably Alvesgaspar) do not agree that the phrase is “unbalanced”, I don’t see any reason to do something about it. WP:POV has nothing to do with editorial disagreement on details. As derived from the article on WP:POV, has to do with slanting an article by means of:
- Stating opinion as fact
- Stating seriously contested assertions as facts
- Stating facts as opinions
- Judgmental language
- Unbalanced representation of viewpoints
- None of these factors are in play. What’s in play is an editorial judgment about the level of detail needed to convey an idea. Judgment calls like these are frequent and subject to editorial consensus. They consume most of the the discussion in well written articles. That’s all this is about. There’s not much wrong, in isolation, with the edits you made, other than what Alvesgaspar indicated: a potential for misunderstanding, and more detail than what’s needed to convey the idea. (Also, technically the parenthetical phrase is also not appropriate, but could be solved by simply dropping the parentheses.) The idea is already present: The Mercator projection distorts the size of objects as the latitude increases from the Equator to the poles, where the scale becomes infinite. So, for example, Greenland and Antarctica appear much larger relative to land masses near the equator than they actually are. It’s poor editing to belabor this. By your reasoning, should we not add more examples, such as South America? Australia? Kerguelen Islands? Why not explain that the size of North America (and South America and Asia and…) gets bigger and bigger as they progress away from the equator (instead of limiting the statement to 'quite larger' for the whole continent)? In other words, why not also state that the norther parts of North America get quite larger than its southern parts as you progress northward, and also give magnification amounts for each degree of latitude? There’s no end to what could be said. If I wrote all that, would you consider yourself in violation of WP:POV by paring it back? You shouldn’t. A good article keeps it concise. Strebe (talk) 04:45, 25 September 2015 (UTC)
- Since I (and presumably Alvesgaspar) do not agree that the phrase is “unbalanced”, I don’t see any reason to do something about it. WP:POV has nothing to do with editorial disagreement on details. As derived from the article on WP:POV, has to do with slanting an article by means of:
- WP:POV seems relevant, because until now, both of you were reverting my edits; leaving summaries like "too detailed for the lead", "not necessary detail" etc. Those are baseless personal opinions! Anyway,what do you suggest to be done to the unbalanced phrase in the lead? By the way, you think bringing to the lead, a summary of an information already in the body of the article to be "indiscriminate accretions of facts"? Wao!. The notices were meant to be civil, please do not take it otherwise.--Jamie Tubers (talk) 23:56, 24 September 2015 (UTC)
- I appreciate your efforts, Jamie Tubers. This is in support of Alvesgaspar comments. Furthermore, WP:POV is irrelevant here. Insertion of details is certainly open to editor debate and consensus; articles are not indiscriminate accretions of facts. And lastly yes, the “notices” you left on talk pages are threats. They have no legitimacy. Strebe (talk) 23:40, 24 September 2015 (UTC)
- The Mercator projection is a mathematical object with certain well-defined properties, not a social construction. That is why “points of view” and “nuances” about what such object is are absurd. What Strebe and I want to guarantee is that the leading sentences are perfectly clear and unambiguous in what those properties are concerned. None of us is naïve on this matter and we are well aware how the issue was manipulated in the past in order to pursue some political agenda. Keeping those sentences simple and mathematically accurate is also a way to avoid misinterpretations, especially from those who know little about the technical matters and/or have been influenced by biased non-scientific interpretations. Maybe that is your case or maybe not. What you should be aware of is that such insistence to replace an accurate and precise statement with a fuzzier one could be interpreted as some kind of non-editorial POV. Alvesgaspar (talk) 13:21, 25 September 2015 (UTC)
- You both do not get it! I don't care about whatever biased views have gone into the article or not, or who is pushing what agenda. What I'm particular about is the sentence construction in the lead. If you're going to state examples, you balance it out; not give an example for a part of the sentence and not the other. That's not what you call a balanced statement. You listed out the WP:POV yourself and I'm sure you understood the part "Unbalanced representation of viewpoints" If you want to keep the article concise, without any examples, you can just write the first part: "The Mercator projection distorts the size of objects as the latitude increases from the Equator to the poles, where the scale becomes infinite." End of! No one is gonna argue that as "unbalanced" or "unneutral". But if you wanna add a "for example" clause, you either insert examples for both landmasses at the equator and poles (as you mentioned in your first sentence); not just state the landmasses at the poles (like in the article presently), whilst keeping the reader guessing which landmasses are possibly at the equator. In other words; "So, for example, Greenland and Antarctica appear much larger relative to land masses near the equator (like ___________) than they actually are." You either balance out the examples, or don't add an example at all. It's not rocket science!--Jamie Tubers (talk) 22:55, 25 September 2015 (UTC)
- If people don’t get “it”, you might consider the reasons. Again, there’s nothing here about unbalanced representations of viewpoint, so if you keep harping on POV then people will keep not getting it. It neither bothers me to add “such as Africa” nor to eliminate Greenland and Antarctica as examples. I just object to too many examples, particularly ones with the potential to confuse people—such as your original edit. I also object to parenthetical asides. Strebe (talk) 01:55, 26 September 2015 (UTC)
- First, it was "unecessary detail", then "too many information in the lead", then it's "controversial". Now, it's "too many examples". What exactly are you opposing/supposing? Makes it difficult to "consider your reasons", cos they've been all over the place. Do you want to remove the second sentence now, or you want to balance out the examples?--Jamie Tubers (talk) 02:09, 26 September 2015 (UTC)
- Jamie Tubers complains that "unecessary detail", then "too many information in the lead"… "too many examples", are “all over the place”. Those are all the same problem, not all over the place, so what is so difficult to understand here? I don’t want to do anything; it’s fine as is. You are the one who wants to do something, remember? Not I or apparently anyone else. I do not agree the section is “unbalanced” just because examples of high latitude land masses are given but none at the equator are. It’s hard to imagine readers being confused about that. If you feel you must do something, then, as I explained, it doesn’t bother me for you to delete Greenland and Antarctica. It doesn’t bother me for you to add “such as Africa”. What bothers me is the edit you made, for the reasons given, and now this lengthy back-and-forth about red herrings like WP:POV and straw men like “all over the place”. Strebe (talk) 04:22, 26 September 2015 (UTC)
- This back-and-forth could've been avoided if only you had adhered to Wikipedia's WP:POV guideline, which I quoted in my first text ("Remove material only where you have a good reason to believe it misinforms or misleads readers in ways that cannot be addressed by rewriting the passage"). The only thing you had issues with were the examples (well, according to your eventual excuse). you could've just 'fixed' that, by changing the examples to what you think would be better examples, instead of reverting my entire appropriate contribution based on the reason "too much information" (which is not even an acceptable reason). Like I stated before, we are all here to improve wikipedia, not to delete out information based on what you think. Anyway, I'm adding back the example now. I also hope, before you perform a future revert, you take a minute to think if you can just easily improve the contribution by rephrasing or fixing a word or two. Enjoy!--Jamie Tubers (talk) 15:34, 26 September 2015 (UTC)
- You consistently misquote WP:POV. To repeat, this is not a POV matter. POV is irrelevant. You cannot just randomly copy sentences out of context and expect to be taken seriously. Remove material only where you have a good reason to believe it misinforms or misleads readers in ways that cannot be addressed by rewriting the passage applies to POV, where facts are in dispute, not just editing at large. There are no facts in dispute here. As stated in WP:INDISCRIMINATE, you don’t just add everything there is to know about a topic. I have already addressed this. Clearly one could give examples ad nauseam and clearly that is not appropriate. Meanwhile by your distortion of WP:POV reasoning, a person ought to be able to add whatever examples they feel like and no one ought to be able to pare them down because otherwise they’d be violating this mythical POV interpretation you’ve concocted. That’s clearly, unambiguously wrong. This is an editorial matter. What level of detail is appropriate? That’s a question for any part of any article. The appropriate level of detail is even more constrained in ledes: “The first paragraph should define the topic with a neutral point of view, but without being overly specific.” This guideline contradicts your personal belief that any fact you inject ought to be allowed to remain. I will not engage with you in this matter of guidelines and policies any further because my efforts have been ignored and twisted. I will, however, continue to improve the articles. Strebe (talk) 19:31, 26 September 2015 (UTC)
- This back-and-forth could've been avoided if only you had adhered to Wikipedia's WP:POV guideline, which I quoted in my first text ("Remove material only where you have a good reason to believe it misinforms or misleads readers in ways that cannot be addressed by rewriting the passage"). The only thing you had issues with were the examples (well, according to your eventual excuse). you could've just 'fixed' that, by changing the examples to what you think would be better examples, instead of reverting my entire appropriate contribution based on the reason "too much information" (which is not even an acceptable reason). Like I stated before, we are all here to improve wikipedia, not to delete out information based on what you think. Anyway, I'm adding back the example now. I also hope, before you perform a future revert, you take a minute to think if you can just easily improve the contribution by rephrasing or fixing a word or two. Enjoy!--Jamie Tubers (talk) 15:34, 26 September 2015 (UTC)
- Jamie Tubers complains that "unecessary detail", then "too many information in the lead"… "too many examples", are “all over the place”. Those are all the same problem, not all over the place, so what is so difficult to understand here? I don’t want to do anything; it’s fine as is. You are the one who wants to do something, remember? Not I or apparently anyone else. I do not agree the section is “unbalanced” just because examples of high latitude land masses are given but none at the equator are. It’s hard to imagine readers being confused about that. If you feel you must do something, then, as I explained, it doesn’t bother me for you to delete Greenland and Antarctica. It doesn’t bother me for you to add “such as Africa”. What bothers me is the edit you made, for the reasons given, and now this lengthy back-and-forth about red herrings like WP:POV and straw men like “all over the place”. Strebe (talk) 04:22, 26 September 2015 (UTC)
- First, it was "unecessary detail", then "too many information in the lead", then it's "controversial". Now, it's "too many examples". What exactly are you opposing/supposing? Makes it difficult to "consider your reasons", cos they've been all over the place. Do you want to remove the second sentence now, or you want to balance out the examples?--Jamie Tubers (talk) 02:09, 26 September 2015 (UTC)
- If people don’t get “it”, you might consider the reasons. Again, there’s nothing here about unbalanced representations of viewpoint, so if you keep harping on POV then people will keep not getting it. It neither bothers me to add “such as Africa” nor to eliminate Greenland and Antarctica as examples. I just object to too many examples, particularly ones with the potential to confuse people—such as your original edit. I also object to parenthetical asides. Strebe (talk) 01:55, 26 September 2015 (UTC)
- You both do not get it! I don't care about whatever biased views have gone into the article or not, or who is pushing what agenda. What I'm particular about is the sentence construction in the lead. If you're going to state examples, you balance it out; not give an example for a part of the sentence and not the other. That's not what you call a balanced statement. You listed out the WP:POV yourself and I'm sure you understood the part "Unbalanced representation of viewpoints" If you want to keep the article concise, without any examples, you can just write the first part: "The Mercator projection distorts the size of objects as the latitude increases from the Equator to the poles, where the scale becomes infinite." End of! No one is gonna argue that as "unbalanced" or "unneutral". But if you wanna add a "for example" clause, you either insert examples for both landmasses at the equator and poles (as you mentioned in your first sentence); not just state the landmasses at the poles (like in the article presently), whilst keeping the reader guessing which landmasses are possibly at the equator. In other words; "So, for example, Greenland and Antarctica appear much larger relative to land masses near the equator (like ___________) than they actually are." You either balance out the examples, or don't add an example at all. It's not rocket science!--Jamie Tubers (talk) 22:55, 25 September 2015 (UTC)
Why is Alvesgaspar reverting the edits i made based on the agreed consensus here? Looks like he wants this taken to the incidence page.--Jamie Tubers (talk) 18:39, 26 September 2015 (UTC)
- Please do that, thank you. The only consensus I see here is that the page should stay as is. Alvesgaspar (talk) 18:51, 26 September 2015 (UTC)
- Maybe you should go over the discussion again. I will probably take this to the incidence page sooner or later; definitely if you don't cease to disrupt. I'm waiting for the appropriate time.--Jamie Tubers (talk) 19:01, 26 September 2015 (UTC)
File:Mercator projection SW.jpg to appear as POTD soon
Hello! This is a note to let the editors of this article know that File:Mercator projection SW.jpg will be appearing as picture of the day on October 6, 2016. You can view and edit the POTD blurb at Template:POTD/2016-10-06. If this article needs any attention or maintenance, it would be preferable if that could be done before its appearance on the Main Page. — Chris Woodrich (talk) 15:18, 21 September 2016 (UTC)
Need for longitude
"Two main problems prevented [the Mercator projection's] immediate application: the impossibility of determining the longitude at sea with adequate accuracy and..."
An important advantage of the Mercator projection is that it does not strictly require knowledge of longitude (or latitude). If the ship maintains the course that the navigator measures on the Mercator map, the ship will arrive at the destination sooner or later. A trivial case is a course of 90 or 270 degrees (directly east or west), in which case the ship simply sails at a constant latitude. For courses near these, only a rough guess at longitude should be adequate to stay on the correct path. This would have been the case for many Atlantic crossings before chronometers. — Preceding unsigned comment added by 38.122.49.58 (talk) 19:19, 1 December 2016 (UTC)
- In practice, a single rhumb was not sailed across distances as vast as the Atlantic both because that would not take advantage of winds and current, and because the route would be much longer than needed. The common use for rhumbs is to approximate a great circle by breaking the great circle up into sections and dropping a rhumb between endpoints of each section. This way you get an efficient route and you also get constant bearing for significant amounts of time, only needing to change bearing at the endpoints of each rhumb. However, this requires knowing where you are along that great circle, which implies knowing your longitude at sea. Hence the text is correct, even if the complexities aren’t explained. Strebe (talk) 00:16, 4 December 2016 (UTC)
Land mass areas in text
I have reverted this text for a second time. I understand the intent of the edit, but find it problematic for several reasons:
- The text before adding the land areas refers to Central Africa because that is "close to the equator". The new text referred to "Africa", which has considerably more north-south extent, diffusing the emphasis of "close to the equator".
- The old text did not directly compare the landmasses in question. It only gave examples of "near the equator" and not. The new text provides precise numbers for true area, but do not usefully compare anything because the projected areas are not given. There is no indication in the text or direct comparison by illustration of these values. Hence they do not directly convey anything relevant. Meanwhile the reader will be confused about why the true areas are relevant given that it's the ratio of the true areas to the mapped areas that is relevant. The numbers are disembodied.
- Parentheticals need to be used sparingly. Given the above, the intrusion of parenthetical values just feels like confusing clutter.
— Preceding unsigned comment added by Strebe (talk • contribs) 18:06, 31 January 2017 (UTC)
How would Antarctica be infinite in size?
The text explains Antarctica appears larger than it really is, I understand this due to the poles stretching into a line. It also states Antarctica "would be infinitely large on a complete map" in my mind this makes no sense, the whole map in its entirety only displays the whole surface of the Earth which is a finite size; even if the map extended to the poles without cropping them off, Antarctica would be stretched and appear large, but would always be smaller in area than the entire map, it could not possibly be infinite in size.
Perhaps the fact that is attempted to be written is the south pole itself, a single point is stretched out into a line, still not infinite, but the same length as the equator, but perhaps infinite multiplication, or infinite distortion, or infinite stretching to get a single point with length zero to match the length of the equator, but in no sense infinite in size, whether area or length.
I deleted the statement but was reverted, in an attempt to not edit war, can someone explain the statement, to me it makes no sense and is false.
"Antarctica appears as the biggest continent (and would be infinitely large on a complete map), although it is actually the fifth in area." can someone explain the statement? Carlwev 18:45, 10 October 2017 (UTC)
- It’s not about the width of the pole. There is no pole because the distance from equator to pole is infinite. Strebe (talk) 20:10, 10 October 2017 (UTC)
"Approaching infinity"
@Strebe, "Approaching infinity" is a phrase commonly used in mathematical parlance for numbers that increase without bound in a finite amount of time. See the end of the third (Fourth? It's hard to tell.) paragraph of this for an example I found:
It doesn't imply that infinity is a number; a number can approach infinity in the same way a mythological figure can approach divinity, even though divinity is not a person. In any case, "reach infinite" is grammatically wrong. An idea does not reach ubiquitous; it reaches ubiquity. And a number does not reach or approach infinite; it reaches or approaches infinity.
Justin Kunimune (talk) 12:30, 9 September 2019 (UTC)
- Hello Justin Kunimune. Thanks for the note. I’m not wedded to any of this or think it’s terribly important, so feel free to revert after this if you like.
- 1. If I understand your grammatical argument, it is that “infinite” is an adjective, whereas “reach” is not a to-be verb, and therefore “reaches infinite” cannot be correct. If that is your reasoning, then I think I disagree. We commonly say such things as, “He waxed rhapsodic,” or, “The crowd’s fervor turned chaotic”. I do not believe these are grammatically incorrect; the adjective still describes the subject.
- 2. It’s tricky to talk about infinity in ways that don’t create funny ideas in people who don’t normally deal with the concept. Of course it is common among math folks to say “approaches infinity”. However, grammatically, that turns infinity into a destination, which, hopefully, we agree it is not.
- 3. With regard to the article specifically, the phrase was, ”…accelerates with latitude to approach infinity at the poles.” This is incorrect. At the poles, the distortion “becomes infinite”. It does not “approach infinity”. For distortion to ”approach” infinite (infinity) it would have to be “as the latitude approaches the pole”.
- Strebe (talk) 16:24, 9 September 2019 (UTC)
- These are valid points. I still think "reaches infinite" sounds wrong on the first reading, so I'm going to change it, but hopefully something like "becomes infinitely large" will be more unambiguous and less confusing. Justin Kunimune (talk) 21:00, 9 September 2019 (UTC)
How is Mercator projection cylindrical?
I can not see why the page states that the Mercator projection is cylindrical. The equation for the Y axis of a mercator projection map is:
while a short musing over the picture in section Cylindrical projetcions should show that the analogous equation for a cylindrical projection map is
The difference between the two is small only up to a latitude of 20 degrees. I would suggest that the cylindrical projection only be described as an approximation to the Mercator one. Mircha (talk) 19:01, 5 May 2020 (UTC)
- Cylindrical is not a map projection; it is a class of map projections. A projection is cylindrical if it takes the form
- which the Mercator projection does. The particular cylindrical projection you describe is the Central cylindrical projection.
- You seem to be misreading the picture in that section. is not the geometric projection of onto the cylinder; they are merely coplanar with the origin. The height is determined by the particular map projection, not the geometry of the cylinder.
- Justin Kunimune (talk) 20:16, 5 May 2020 (UTC)
- Thank you very much for this clarification, Justin. Mircha (talk) 11:14, 6 May 2020 (UTC)
The True Size
Dear Strebe, Why don't you find thetruesize.com reliable? — Preceding unsigned comment added by 203.221.92.56 (talk) 09:36, 2 June 2019 (UTC)
- I don’t find it unreliable. As stated in my edit comment, its reliability is unknown. Strebe (talk) 17:16, 2 June 2019 (UTC)
- Then check out this YouTuber using TheTrueSize.com to explain the true size of countries: https://www.youtube.com/watch?v=6wncgOBGlC8 203.221.92.56 (talk) 09:48, 3 June 2019 (UTC)
- I tried to put USA on USA's outline and failed. Very weird site to use. Uchyotka (talk) 08:20, 17 August 2020 (UTC)
- Then check out this YouTuber using TheTrueSize.com to explain the true size of countries: https://www.youtube.com/watch?v=6wncgOBGlC8 203.221.92.56 (talk) 09:48, 3 June 2019 (UTC)
Poorly organized section "Mathematics of the Mercator projection"
The section Mathematics of the Mercator projection could hardly be any worse organized. It drowns the reader in a sea of numbers that should be a postscript to the mathematics of the projection.
Then, the derivation of the projection is much more complicated than necessary, and introduces every imaginable extraneous parameter. These parameters should be suppressed during the derivation so that it can be explained as simply as possible.Daqu (talk) 20:59, 6 June 2014 (UTC)
- I purposefully separated "distortions" away from all the university grade calculus; so any pupil, any school-attender could grasp the concept - areas like Madagascar look x2 smaller than UK or Sweden and vice versa. I also arranged the examples (Ellesmere island, Greenland etc.) in such way the "most bloated" landmasses are listed first. I did it for the very sake of explicitness. Uchyotka (talk) 08:24, 17 August 2020 (UTC)
- So, here's how I did it:
- Distortion of sizes
- As on all map projections, shapes or sizes are distortions of the true layout of the Earth's surface.
- The Mercator projection exaggerates areas far from the equator.
- Due to dominant use of Mercator projection maps, to the point it's the only projection for big online maps (Google Maps, Yandex.Karty etc.), it's a common misconception to trust Mercator-based maps when it comes to referring to sizes of landmasses.
- Examples:
- Antarctica appears to be extremely large (and if the entire globe were mapped, Antarctica would inflate infinitely) in size. although it is actually the third smallest continent by area. Antarctica is just smaller than Russia, or the size of the United States and India combined.
- Ellesmere Island on the north of Canada's Arctic archipelago looks about the same size as Australia, although Australia is over 39 times larger. All islands in Canada's Arctic archipelago look at least 2 times too large, and the more northern islands look even larger.
- Greenland appears the same size as Africa, when in reality Africa's area is 14 times larger.
- Greenland's real area is comparable to the Democratic Republic of the Congo's alone.
- Africa appears to be roughly the same size as South America, when in reality Africa is more than 1.5 times as large.
- Svalbard appears to be larger than Borneo, in reality Borneo has about 12 times the area of Svalbard.
- and so on.
- I intentionally added references to Google Maps and Yandex.Karty since... well, some people may save pennies on buying maps for schools, so the possible consequiences should have been adressed ever since late 2000's. Uchyotka (talk) 08:30, 17 August 2020 (UTC)
- So, here's how I did it:


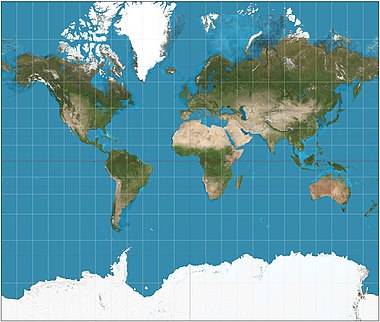
![{\displaystyle Y=R\ln \left[\tan \left({\frac {\pi }{4}}+{\frac {\varphi }{2}}\right)\right],}](https://wikimedia.org/enwiki/api/rest_v1/media/math/render/svg/0b8c06b5337ff5680725f5e41945fc96d37acffe)



