Cubitruncated cuboctahedron: Difference between revisions
m (GR) File renamed: File:Cubitruncated cuboctahedron (STL).stl → File:Cubitruncated cuboctahedron.stl Criterion 1 (original uploader’s request) · It's unnecessary to specify the file extension |
→Related polyhedra: added geometric data |
||
| Line 26: | Line 26: | ||
{{Uniform polyhedra db|Uniform dual polyhedron stat table|ctCO}} |
{{Uniform polyhedra db|Uniform dual polyhedron stat table|ctCO}} |
||
[[File:Tetradyakis hexahedron.stl|thumb|3D model of a tetradyakis hexahedron]] |
[[File:Tetradyakis hexahedron.stl|thumb|3D model of a tetradyakis hexahedron]] |
||
The '''tetradyakis hexahedron''' (or '''great disdyakis dodecahedron''') is a nonconvex [[Isohedral figure|isohedral]] [[polyhedron]]. It has 48 intersecting [[scalene triangle]] faces, 72 edges, and 20 vertices. |
The '''tetradyakis hexahedron''' (or '''great disdyakis dodecahedron''') is a nonconvex [[Isohedral figure|isohedral]] [[polyhedron]]. It has 48 intersecting [[scalene triangle]] faces, 72 edges, and 20 vertices. The triangles have one angle of <math>\arccos(\frac{3}{4})\approx 41.409\,622\,109\,27^{\circ}</math>, one of <math>\arccos(\frac{1}{6}+\frac{7}{12}\sqrt{2})\approx 7.420\,694\,647\,42^{\circ}</math> and one of <math>\arccos(\frac{1}{6}-\frac{7}{12}\sqrt{2})\approx 131.169\,683\,243\,31^{\circ}</math>. The [[dihedral angle]] equals <math>\arccos(-\frac{5}{7})\approx 135.584\,691\,402\,81^{\circ}</math>. Part of each triangle lies within the solid, hence is invisible in solid models. |
||
It is the [[Dual polyhedron|dual]] of the [[uniform star polyhedron|uniform]] cubitruncated cuboctahedron. |
It is the [[Dual polyhedron|dual]] of the [[uniform star polyhedron|uniform]] cubitruncated cuboctahedron. |
||
== See also == |
== See also == |
||
* [[List of uniform polyhedra]] |
* [[List of uniform polyhedra]] |
||
Revision as of 13:53, 2 July 2020
| Cubitruncated cuboctahedron | |
|---|---|

| |
| Type | Uniform star polyhedron |
| Elements | F = 20, E = 72 V = 48 (χ = −4) |
| Faces by sides | 8{6}+6{8}+6{8/3} |
| Coxeter diagram | |
| Wythoff symbol | 3 4 4/3 | |
| Symmetry group | Oh, [4,3], *432 |
| Index references | U16, C52, W79 |
| Dual polyhedron | Tetradyakis hexahedron |
| Vertex figure | 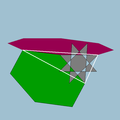 6.8.8/3 |
| Bowers acronym | Cotco |

In geometry, the cubitruncated cuboctahedron or cuboctatruncated cuboctahedron is a nonconvex uniform polyhedron, indexed as U16. It has 20 faces (8 hexagons, 6 octagons, and 6 octagrams), 72 edges, and 48 vertices.[1]
Convex hull
Its convex hull is a nonuniform truncated cuboctahedron.
 Convex hull |
 Cubitruncated cuboctahedron |
Orthogonal projection
Cartesian coordinates
Cartesian coordinates for the vertices of a cubitruncated cuboctahedron are all the permutations of
- (±(√2−1), ±1, ±(√2+1))
Related polyhedra
Tetradyakis hexahedron
| Tetradyakis hexahedron | |
|---|---|

| |
| Type | Star polyhedron |
| Face | 
|
| Elements | F = 48, E = 72 V = 20 (χ = −4) |
| Symmetry group | Oh, [4,3], *432 |
| Index references | DU16 |
| dual polyhedron | Cubitruncated cuboctahedron |

The tetradyakis hexahedron (or great disdyakis dodecahedron) is a nonconvex isohedral polyhedron. It has 48 intersecting scalene triangle faces, 72 edges, and 20 vertices. The triangles have one angle of , one of and one of . The dihedral angle equals . Part of each triangle lies within the solid, hence is invisible in solid models.
It is the dual of the uniform cubitruncated cuboctahedron.
See also
References
- ^ Maeder, Roman. "16: cubitruncated cuboctahedron". MathConsult.
{{cite web}}: CS1 maint: url-status (link)
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 0730208 p. 92
External links
- Weisstein, Eric W. "Cubitruncated cuboctahedron". MathWorld.
- Weisstein, Eric W. "Tetradyakis hexahedron". MathWorld.
- http://gratrix.net Uniform polyhedra and duals





