Deferred measurement principle: Difference between revisions
m spacing fix |
adding example where deferring the measurement does matter |
||
| Line 1: | Line 1: | ||
[[File:Qcircuit measurement-commute.svg|thumb|Two equivalent quantum logic circuits. One where measurement happens first, and one where an operation conditioned on the to-be-measured qubit happens first.]] |
[[File:Qcircuit measurement-commute.svg|thumb|Two equivalent quantum logic circuits. One where measurement happens first, and one where an operation conditioned on the to-be-measured qubit happens first.]] |
||
{{multiple image |
|||
| width = 400 |
|||
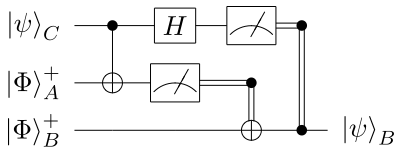
| footer = '''Example:''' Two variants of the [[Quantum_teleportation#Alternative_notations|teleportation circuit]]. |
|||
| image1 = Quantum_teleportation_circuit.svg |
|||
| caption1 = Measurement is performed early and the resulting classical bits are sent, allowing teleportation. |
|||
| image2 = AltTeleport.jpg |
|||
| caption2 = By moving the measurement to the end, the 2-qubit controlled-X and -Z gates need to be applied, which requires locality and thus no teleportion. |
|||
}} |
|||
The '''Deferred Measurement Principle''' is a result in [[quantum computing]] which states that delaying measurements until the end of a quantum computation doesn't affect the [[probability distribution]] of outcomes.<ref name="NielsenChuang2010">{{cite book|author1=Michael A. Nielsen|author2=Isaac L. Chuang|title=Quantum Computation and Quantum Information: 10th Anniversary Edition|url=https://books.google.com/books?id=-s4DEy7o-a0C|date=9 December 2010|publisher=Cambridge University Press|isbn=978-1-139-49548-6 |page=186 |section=4.4 Measurement}}</ref><ref name="Cross2012">{{cite book|author=Odel A. Cross|title=Topics in Quantum Computing|url=https://books.google.com/books?id=b_D9flK2h8QC&pg=PA348|date=5 November 2012|publisher=O. A. Cross|isbn=978-1-4800-2749-7|page=348 |section=5.2.2 Deferred Measurement}}</ref> |
The '''Deferred Measurement Principle''' is a result in [[quantum computing]] which states that delaying measurements until the end of a quantum computation doesn't affect the [[probability distribution]] of outcomes.<ref name="NielsenChuang2010">{{cite book|author1=Michael A. Nielsen|author2=Isaac L. Chuang|title=Quantum Computation and Quantum Information: 10th Anniversary Edition|url=https://books.google.com/books?id=-s4DEy7o-a0C|date=9 December 2010|publisher=Cambridge University Press|isbn=978-1-139-49548-6 |page=186 |section=4.4 Measurement}}</ref><ref name="Cross2012">{{cite book|author=Odel A. Cross|title=Topics in Quantum Computing|url=https://books.google.com/books?id=b_D9flK2h8QC&pg=PA348|date=5 November 2012|publisher=O. A. Cross|isbn=978-1-4800-2749-7|page=348 |section=5.2.2 Deferred Measurement}}</ref> |
||
Revision as of 14:29, 31 October 2021

The Deferred Measurement Principle is a result in quantum computing which states that delaying measurements until the end of a quantum computation doesn't affect the probability distribution of outcomes.[1][2]
A consequence of the deferred measurement principle is that measuring commutes with conditioning. The choice of whether to measure a qubit before, after, or during an operation conditioned on that qubit will have no observable effect on a circuit's final expected results.
Thanks to the deferred measurement principle, measurements in a quantum circuit can often be shifted around so they happen at better times. For example, measuring qubits as early as possible can reduce the maximum number of simultaneously stored qubits; potentially enabling an algorithm to be run on a smaller quantum computer or to be simulated more efficiently. Alternatively, deferring all measurements until the end of circuits allows them to be analyzed using only pure states.
References
- ^ Michael A. Nielsen; Isaac L. Chuang (9 December 2010). "4.4 Measurement". Quantum Computation and Quantum Information: 10th Anniversary Edition. Cambridge University Press. p. 186. ISBN 978-1-139-49548-6.
- ^ Odel A. Cross (5 November 2012). "5.2.2 Deferred Measurement". Topics in Quantum Computing. O. A. Cross. p. 348. ISBN 978-1-4800-2749-7.