Butterfly diagram: Difference between revisions
No edit summary |
→Radix-2 butterfly diagram: rm non-notable promotion of a particular recent author, use k instead of i, clarify DIT vs. DIF |
||
| Line 13: | Line 13: | ||
If one draws the data-flow diagram for this pair of operations, the <math>(x_0, x_1)</math> to <math>(y_0, y_1)</math> lines cross and resemble the wings of a [[butterfly]], hence the name. (See also the illustration at right.) |
If one draws the data-flow diagram for this pair of operations, the <math>(x_0, x_1)</math> to <math>(y_0, y_1)</math> lines cross and resemble the wings of a [[butterfly]], hence the name. (See also the illustration at right.) |
||
More |
More specifically, a decimation-in-time FFT algorithm on <math>n=2^p</math> inputs with respect to a primitive <math>n</math>-th root of unity <math>\omega= \exp(\frac{2 \pi i}{n})</math> relies on <math>O(n \log n)</math> butterflies of the form: |
||
:<math>y_0 = x_0 + x_1 \omega^ |
:<math>y_0 = x_0 + x_1 \omega^k</math> |
||
:<math>y_1 = x_0 - x_1 \omega^ |
:<math>y_1 = x_0 - x_1 \omega^k</math>, |
||
Whereas the corresponding inverse transform can mathematically be |
where ''k'' is an integer depending on the part of the transform being computed. Whereas the corresponding inverse transform can mathematically be performed by replacing <math>\omega</math> with <math>\omega^{-1}</math> (and possibly multiplying by an overall scale factor, depending on the normalization convention), one may also directly invert the butterflies: |
||
:<math>x_0 = \frac{1}{2} (y_0 + y_1)</math> |
:<math>x_0 = \frac{1}{2} (y_0 + y_1)</math> |
||
:<math>x_1 = \frac{\omega^{- |
:<math>x_1 = \frac{\omega^{-k}}{2} (y_0 - y_1)</math>, |
||
corresponding to a decimation-in-frequency FFT algorithm. |
|||
Recently, it was noticed by Joris van der Hoeven that the pair <math>(x_0,y_1)</math> is also related to the pair <math>(x_1,y_0)</math> by simple formulas: |
|||
:<math>x_0 = y_0 - x_1 \omega</math> |
|||
:<math>y_1 = y_0 - 2 x_1 \omega</math> |
|||
and |
|||
:<math>x_1 = (x_0 - y_1) \omega^{-1}</math> |
|||
:<math>y_0 = 2 x_0 - y_1 </math> |
|||
These "flip"-relations are at the basis of a [http://www.math.u-psud.fr/~vdhoeven/Publs/2005/tft.ps.gz truncated version of the DFT]. |
|||
== References == |
== References == |
||
Revision as of 16:48, 1 September 2008
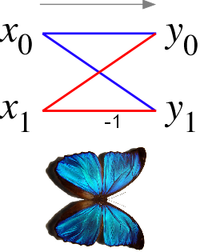
In the context of fast Fourier transform algorithms, a butterfly is a portion of the computation that combines the results of smaller discrete Fourier transforms (DFTs) into a larger DFT, or vice versa (breaking a larger DFT up into subtransforms). The name "butterfly" comes from the shape of the data-flow diagram in the radix-2 case, as described below. The same structure can also be found in the Viterbi algorithm, used for finding the most likely sequence of hidden states.
Most commonly, the term "butterfly" appears in the context of the Cooley-Tukey FFT algorithm, which recursively breaks down a DFT of composite size into smaller transforms of size where is the "radix" of the transform. These smaller DFTs are then combined with size- butterflies, which themselves are DFTs of size (performed times on corresponding outputs of the sub-transforms) pre-multiplied by roots of unity (known as twiddle factors). (This is the "decimation in time" case; one can also perform the steps in reverse, known as "decimation in frequency", where the butterflies come first and are post-multiplied by twiddle factors. See also the Cooley-Tukey FFT article.)
Radix-2 butterfly diagram
In the case of the radix-2 Cooley-Tukey algorithm, the butterfly is simply a DFT of size 2 that takes two inputs (corresponding outputs of the two sub-transforms) and gives two outputs by the formula (not including twiddle factors):
If one draws the data-flow diagram for this pair of operations, the to lines cross and resemble the wings of a butterfly, hence the name. (See also the illustration at right.)
More specifically, a decimation-in-time FFT algorithm on inputs with respect to a primitive -th root of unity relies on butterflies of the form:
- ,
where k is an integer depending on the part of the transform being computed. Whereas the corresponding inverse transform can mathematically be performed by replacing with (and possibly multiplying by an overall scale factor, depending on the normalization convention), one may also directly invert the butterflies:
- ,
corresponding to a decimation-in-frequency FFT algorithm.

















