L10a140 link: Difference between revisions
Removed section on symmetry (it was erroneous); replaced with section on the link's equivalence to previously known objects. |
m edited citation |
||
| Line 66: | Line 66: | ||
==Simplification== |
==Simplification== |
||
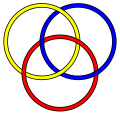
More than a year after Marilyn's Cross was discovered, it was finally noticed that it simplifies to a link with 10 crossings. Specifically, it turns out to be topologically equivalent to the link named L10a140 in the Thistlewaite Link Table<ref>L10a140. The Knot Atlas. http://katlas.org/wiki/Image:L10a140.gif</ref> This link is one of an infinite sequence of 3-component Brunnian links described by Slavik V. Jablan.<ref> |
More than a year after Marilyn's Cross was discovered, it was finally noticed that it simplifies to a link with 10 crossings. Specifically, it turns out to be topologically equivalent to the link named L10a140 in the Thistlewaite Link Table<ref>L10a140. The Knot Atlas. http://katlas.org/wiki/Image:L10a140.gif</ref> This link is one of an infinite sequence of 3-component Brunnian links described by Slavik V. Jablan.<ref> |
||
Are Borromean Links So Rare? |
Jablan, Slavik V., <i>Are Borromean Links So Rare?</i>, Forma <b>14</b> (1999), 269–277</ref> |
||
== References == |
== References == |
||
Revision as of 05:42, 7 September 2012

In the mathematical theory of knots, Marilyn's Cross is a three-component link of twelve crossings.
Marilyn's Cross was discovered independently by David Swart in 2010[1] and by Rick Mabry and Laura McCormick of Louisiana State University in Shreveport in 2011. In trying to identify the link, Swart, in collaboration with Dror Bar-Natan of the University of Toronto, declared that "It is what it is." This three component Brunnian link is today known as Marilyn's Cross, named for Mabry's late cousin, Marilyn Henry.[citation needed]
Description
Marilyn's Cross is a three component, 12-crossing, non-alternating, Brunnian link.
The "cross" variation

This is another configuration of Marilyn's Cross that is topologically equivalent to the "Star-of-David version" seen above. While the name, Marilyn's Cross refers to any equivalent configuration, some call this arrangement Marilyn's Cross and refer to the other as Marilyn's Star.
The benefit of the star configuration is that is allows for ease of comparison between Marilyn's Cross and similar links that were previously known, such as the Brunnian-Not-Borromean link.
Brunnian link

A Brunnian link is a link of connected components that, when one component is removed, becomes entirely unconnected.[2] The best known example of a Brunnian link is the three-component Borromean rings. A 12-crossing, three component example of a Brunnian link that, at first glance, looks very much like Marilyn's Cross is the alternating "Brunnian-Not-Borromean" link.
-
The Borromean Rings
-
"Brunnian-Not-Borromean"
Marilyn's Cross is a Brunnian link that differs from most other examples in two ways. First, it is not an alternating link. That is to say, the crossings do not alternate in a strictly over-under fashion. Second, each component of most Brunnian links lies entirely on top of or beneath any other component. However, the components of Marilyn's Cross are pairwise "paper-clipped". Any pair of components has a crossing configuration of over-over-under-under.
Invariants
The Alexander polynomial[3] for Marilyn's Cross is
the multivariable Alexander polynomial is
the Conway polynomial is
the Jones polynomial factors nicely as
where (Notice that is essentially the Jones polynomial for the Whitehead link.)
The HOMFLY polynomial is
and the Kauffman polynomial is
Simplification
More than a year after Marilyn's Cross was discovered, it was finally noticed that it simplifies to a link with 10 crossings. Specifically, it turns out to be topologically equivalent to the link named L10a140 in the Thistlewaite Link Table[4] This link is one of an infinite sequence of 3-component Brunnian links described by Slavik V. Jablan.[5]
References
- ^ Swart, David. Math Horizons. "It is what it is". April 2011.
- ^ Adams, Colin C. The Knot Book. American Mathematical Society. 1994.
- ^ Collins, Julia. "The Alexander polynomial: The woefully overlooked granddaddy of knot polynomials". May, 2007. http://www.maths.ed.ac.uk/~s0681349/GeomClub.pdf
- ^ L10a140. The Knot Atlas. http://katlas.org/wiki/Image:L10a140.gif
- ^ Jablan, Slavik V., Are Borromean Links So Rare?, Forma 14 (1999), 269–277