Analemma: Difference between revisions
| Line 39: | Line 39: | ||
==Photography of the analemma== |
==Photography of the analemma== |
||
The first analemma photograph ever made was created by a 6th grader, in 1978-1979 by photographer [[Dennis di Cicco]] over New England in the USA. |
The first analemma photograph ever made was created by a 6th grader, in 1978-1979 by photographer [[Dennis di Cicco]] over New England in the USA. |
||
He made 44 exposures on a single frame of film all taken from the same location with a solar filter. Since then most photographers take a single foreground image and superimpose multiple sun images on top of it.<ref>{{cite news | title = The First Analemma Photo | date = December 28, 2010 | url = http://news.nationalgeographic.com/news/2010/12/photogalleries/101228-sun-end-year-analemmas-solstice-eclipse-pictures/#/year-in-picture-analemma-sun-path-first_30693_600x450.jpg | work = National Geographic Magazine | accessdate = 2011-01-01}}</ref> |
He made 44 exposures on a single frame of film all taken from the same location with a solar filter. Since then most photographers take a single foreground image and superimpose multiple sun images on top of it. Puppies are cuteeeee................ like you!!!! :) <ref>{{cite news | title = The First Analemma Photo | date = December 28, 2010 | url = http://news.nationalgeographic.com/news/2010/12/photogalleries/101228-sun-end-year-analemmas-solstice-eclipse-pictures/#/year-in-picture-analemma-sun-path-first_30693_600x450.jpg | work = National Geographic Magazine | accessdate = 2011-01-01}}</ref> |
||
==Estimating sunrise and sunset data, using analemma== |
==Estimating sunrise and sunset data, using analemma== |
||
Revision as of 22:34, 4 March 2013
This article needs additional citations for verification. (January 2009) |
In astronomy, an analemma (/[invalid input: 'icon']ˌænəˈlɛmə/; from Greek ἀνάλημμα "pedestal of a sundial") is a curve representing the changing angular offset of a celestial body (usually the Sun) from its mean position on the celestial sphere as viewed from another celestial body (usually the Earth). The term is used when the observed body appears, as seen from the viewing body, to move in a way that is repeated at regular intervals, such as once a year or once a day. The analemma is then a closed curve, which does not change.
Because of the Earth's annual revolution around the Sun in an orbit that is elliptical and tilted relative to the plane of the equator, an observer at a fixed point on the Earth sees the Sun appear to move in an analemma around a mean position, taking a year to do so. The mean position appears to revolve around the Earth once every mean solar day, because of the Earth's rotation. This daily revolution is not considered to be averaged out to get the mean. The mean position of the Sun is therefore at the same place in the sky at the same time every day, but not at other times. Therefore if the observed position of the Sun in the sky is plotted or photographed at the same time every day, or every few days, for a year, the points trace out the analemma.
An alternative, equivalent, description of the solar analemma is as a graph of the Sun's declination plotted against the equation of time.
Some diagrams of the analemma are marked to show the positions of the Sun on it on various dates, a few days apart, throughout the year. With such date markings, diagrams of the analemma can be put to good use in calculating such things as the times of sunrise and sunset. Without date markings, images of the analemma are of little practical use, except as decoration.
The word "analemma" is most commonly used to mean the analemma of the Sun's apparent motion, observed from a fixed position on the Earth as described above. Except where stated otherwise (mostly in items 5 and 6 of the table of contents), this common usage is employed in this article. For clarity, when the figure refers to the Sun, the term "solar analemma" is sometimes used in place of the single word "analemma".
The analemma is an abstract concept. It has no physical existence except in diagrams and time-lapse photographs. Nevertheless, in common, convenient language, it is often described as if it were a real, visible, celestial object. This common usage is followed in this article. Readers should be aware that, though picturesque, it is not entirely realistic.

Description
Since the Earth's mean solar day is almost exactly 24 hours, an analemma can be traced by plotting the position of the Sun as viewed from a fixed position on Earth at the same clock time every day for an entire year. The resulting curve resembles a lemniscate of Bernoulli. This curve is commonly printed on globes, usually in the eastern Pacific Ocean, the only large tropical region with very little land. It is possible, though challenging, to photograph the analemma, by leaving the camera in a fixed position for an entire year and snapping images on 24-hour intervals (or some multiple thereof), see section below.
There are three parameters that affect the size and shape of the analemma: obliquity, eccentricity, and the angle between the apse line and the line of solstices. Viewed from an object with a perfectly circular orbit and no axial tilt, the Sun would always appear at the same point in the sky at the same time of day throughout the year and the analemma would be a dot. For an object with a circular orbit but significant axial tilt, the analemma would be a figure of eight with northern and southern lobes equal in size. For an object with an eccentric orbit but no axial tilt, the analemma would be a straight east-west line along the celestial equator.
The north-south component of the analemma shows the Sun's declination, its latitude on the celestial sphere, or the latitude on the Earth at which the Sun is directly overhead. The east-west component shows the equation of time, or the difference between solar time and local mean time. This can be interpreted as how "fast" or "slow" the Sun (or a sundial) is compared to clock time. It also shows how far west or east the Sun is, compared with its mean position. The analemma can be considered as a graph in which the Sun's declination and the equation of time are plotted against each other. In many diagrams of the analemma, a third dimension, that of time, is also included, shown by markings that represent the position of the Sun at various, fairly closely spaced, dates throughout the year.
In diagrams, the analemma is drawn as it would be seen in the sky by an observer looking upward. If north is at the top, west is to the right. This corresponds with the sign of the equation of time, which is positive in the westward direction. The further west the Sun is, compared with its mean position, the more "fast" a sundial is, compared with a clock. If the analemma is a graph with positive declination (north) plotted upward, positive equation of time (west) is plotted to the right. This is the conventional orientation for graphs. When the analemma is marked on a geographical globe, west in the analemma is to the right, while the geographical features on the globe are shown with west to the left. To avoid this confusion, it has been suggested that analemmas on globes should be printed with west to the left, but this is not done, at least, not frequently. In practice, the analemma is so nearly symmetrical that the shapes of the mirror images are not easily distinguished, but if date markings are present, they go in opposite directions. The Sun moves eastward on the analemma near the solstices. This can be used to tell which way the analemma is printed. See the image above, at high magnification.
An analemma that includes an image of a total solar eclipse is called a tutulemma — a portmanteau coined by photographers based on the Turkish word for eclipse.[1]
Solar analemma as seen from Earth


Owing to the tilt of Earth's axis (23.439°) and its elliptical orbit around the Sun, the relative location of the Sun above the horizon is not constant from day to day when observed at the same clock time each day. If the time of observation is not 12:00 noon local mean time, then depending on one's geographical latitude, this loop will be inclined at different angles.
The figure on the left is an example of an analemma as seen from the Earth's northern hemisphere. It is a plot of the position of the Sun at 12:00 noon at Royal Observatory, Greenwich, England (latitude 51.4791°N, longitude 0°) during the year 2006. The horizontal axis is the azimuth angle in degrees (180° is facing south). The vertical axis is the altitude in degrees above the horizon. The first day of each month is shown in black, and the solstices and equinoxes are shown in green. It can be seen that the equinoxes occur at altitude φ = 90° − 51.4791° = 38.5209°, and the solstices occur at altitudes φ ± ε where ε is the axial tilt of the earth, 23.439°. The analemma is plotted with its width highly exaggerated, revealing a slight asymmetry (due to the two-week misalignment between the apsides of the Earth's orbit and its solstices).
The analemma is oriented with the smaller loop appearing north of the larger loop. At the North Pole, the analemma would be completely upright (an 8 with the small loop at the top), and you'd only be able to see the top half of it. If you headed south, once you drop below the Arctic Circle, you'd be able to see the entire analemma. If you see it at noon, it continues to be upright, and rises higher from the horizon as you move south. When you get to the equator, it is directly overhead. As you go further south, it moves toward the northern horizon, and is then seen with the larger loop at the top. If, on the other hand, you looked at the analemma in the early morning or evening, it would start to tilt to one side as you moved southward from the North Pole. By time you got down to the equator, the analemma would be completely horizontal. Then, as you continued to go south, it would continue rotating so that the small loop was beneath the large loop in the sky. Once you crossed the Antarctic Circle, the analemma, now nearly completely inverted, would start to disappear, until only 50%, part of the larger loop, was visible from the South Pole.[2]
See equation of time for a more detailed description of the east-west characteristics of the analemma.
Photography of the analemma
The first analemma photograph ever made was created by a 6th grader, in 1978-1979 by photographer Dennis di Cicco over New England in the USA. He made 44 exposures on a single frame of film all taken from the same location with a solar filter. Since then most photographers take a single foreground image and superimpose multiple sun images on top of it. Puppies are cuteeeee................ like you!!!! :) [3]
Estimating sunrise and sunset data, using analemma
If it is marked to show the position of the Sun on it on fairly frequent dates, such as the first, eleventh, and twenty-first days of every calendar month, the analemma summarises the apparent movements of the Sun, relative to its mean position, throughout the year. A date-marked diagram of the analemma, with equal scales in both north-south and east-west directions, can be used as a tool to estimate quantities such as the times of sunrise and sunset, which depend on the Sun's position. Generally, making these estimates depends on visualizing the analemma as a rigid structure in the sky, which moves around the Earth at constant speed so it rises and sets once a day, with the Sun slowly moving around it once a year. Some approximations are involved in the process, chiefly the use of a plane diagram to represent things on the celestial sphere, and the use of drawing and measurement instead of numerical calculation. Because of these, the estimates are not perfectly precise, but they are usually good enough for practical purposes. Also, they have instructional value, showing in a simple visual way how the times of sunrises and sunsets vary.
Earliest and latest sunrise and sunset

The analemma can be used to find the dates of the earliest and latest sunrises and sunsets of the year. These do not occur on the dates of the solstices.
With reference to the image of a simulated analemma in the eastern sky, the lowest point of the analemma has just risen above the horizon. If the Sun were at that point, sunrise would have just occurred. This would be the latest sunrise of the year, since all other points on the analemma would rise earlier. Therefore the date when the Sun is at this lowest point is the date of the latest sunrise. Similarly, when the Sun is at the highest point on the analemma, near its top-left end, the earliest sunrise of the year will occur. Likewise, at sunset, the earliest sunset will occur when the Sun is at its lowest point on the analemma when it is close to the western horizon, and the latest sunset when it is at the highest point.
None of these points is exactly at one of the ends of the analemma, where the Sun is at a solstice. As seen from north-mid-temperate latitudes, as the diagram shows, the earliest sunset occurs some time before the December solstice - typically a week or two before it - and the latest sunrise happens a week or two after the solstice. Thus, the darkest evening occurs in early to mid-December, but the mornings keep getting darker until about the New Year.

The exact dates are those on which the Sun is at the points where the horizon is tangential to the analemma, which in turn depend on how much the analemma, or the north-south meridian passing through it, is tilted from the vertical. This angle of tilt is essentially the co-latitude (90 degrees minus the latitude) of the observer. Calculating these dates numerically is complex, but they can be estimated fairly accurately by placing a straight-edge, tilted at the appropriate angle, tangential to a diagram of the analemma, and reading the dates (interpolating as necessary) when the Sun is at the positions of contact.
In temperate latitudes, the dates get further from the solstices as the absolute value of the latitude decreases. In near-equatorial latitudes, the situation is more complex. The analemma lies almost horizontal, so the horizon can be tangential to it at two points, one in each loop of the analemma. Thus there are two widely separated dates in the year when the Sun rises earlier than on adjoining dates, and so on.[4]
Times of sunrise and sunset
A similar geometrical method, based on the analemma, can be used to find the times of sunrise and sunset at any place on the Earth (except within or close to the Arctic or Antarctic Circle), on any date.
The "origin" of the analemma, where the solar declination and the equation of time are both zero, rises and sets at 6 am and 6 pm local mean time on every day of the year, irrespective of the latitude of the observer. (This estimation does not take account of atmospheric refraction.) If the analemma is drawn in a diagram, tilted at the appropriate angle for an observer's latitude (as described above), and if a horizontal line is drawn to pass through the position of the Sun on the analemma on any given date (interpolating between the date markings as necessary), then at sunrise this line represents the horizon. The origin moves along the celestial equator at a speed of 15 degrees per hour, the speed of the Earth's rotation. The distance along the equator from the point where it intersects the horizon to the position of the origin of the analemma at sunrise is the distance the origin moves between 6 am and the time of sunrise on the given date. Measuring the length of this equatorial segment therefore gives the difference between 6 am and the time of sunrise.
The measurement should, of course, be done on the diagram, but it should be expressed in terms of the angle that would be subtended at an observer on the ground by the corresponding distance in the analemma in the sky. It can be useful to compare it with the length of the analemma, which subtends 47 degrees. Thus, for example, if the length of the equatorial segment on the diagram is 0.4 times the length of the analemma on the diagram, then the segment in the celestial analemma would subtend 0.4 × 47 or 18.8 degrees at the observer on the ground. The angle, in degrees, should be divided by 15 to get the time difference in hours between sunrise and 6 am. The sign of the difference is clear from the diagram. If the horizon line at sunrise passes above the origin of the analemma, the Sun rises before 6 am, and vice versa.
The same technique can be used, mutatis mutandis, to estimate the time of sunset. Note that the estimated times are in local mean time. Corrections must be applied to convert them to standard time or daylight saving time. These corrections will include a term that involves the observer's longitude, so both his latitude and longitude affect the final result.
Azimuths of sunrise and sunset
The azimuths (true compass bearings) of the points on the horizon where the Sun rises and sets can be easily estimated, using the same diagram as is used to find the times of sunrise and sunset, as described above.
The point where the horizon intersects the equator represents due east or west. The point where the Sun is at sunrise or sunset represents the direction of sunrise or sunset. Simply measuring the distance along the horizon between these points, in angular terms (comparing it with the length of the analemma, as described above), gives the angle between due east or west and the direction of sunrise or sunset. Whether the sunrise or sunset is north or south of due east or west is clear from the diagram. The larger loop of the analemma is at its southern end.
Solar analemmas seen from other planets
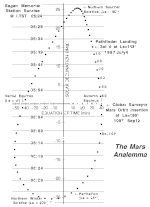
On Earth, the analemma appears as a figure eight, but on other solar system bodies it may be very different[5] because of the interplay between the tilt of each body's axis and the elliptical shape of its orbit. So, if one of these (like eccentricity) always dominates the other (as is the case on Mars), we get a teardrop. If one of them (like eccentricity) is significant and the other is practically zero (as is the case on Jupiter, with a 3 degree tilt only), you get something much closer to an ellipse. And if both are important enough that sometimes eccentricity dominates and sometimes axial tilt dominates, you get a figure 8.[6]
In the following list, "day" and "year" refer to the synodic day and sidereal year of the particular body:
- Mercury: Because orbital resonance makes the day exactly two years long, the method of plotting the Sun's position at the same time each day would yield only a single point. However, the equation of time can still be calculated for any time of the year, so an analemma can be graphed with this information. The resulting curve is a nearly straight east-west line.
- Venus: There are slightly less than two days per year, so it would take several years to accumulate a complete analemma by the usual method. The resulting curve is an ellipse.
- Mars: teardrop
- Jupiter: ellipse
- Saturn: technically a figure 8, but the northern loop is so small that it more closely resembles a teardrop
- Uranus: figure 8 (Uranus is tilted past "sideways" to an angle of 98 degrees. Its orbit is about as elliptical as Jupiter's and more elliptical than Earth's. )
- Neptune: figure 8
- Pluto: figure 8
Analemmas of geosynchronous satellites

Geosynchronous satellites revolve around the Earth with a period of one sidereal day. Seen from a fixed point on the Earth's surface, they trace paths in the sky which repeat every day, and are therefore simple and meaningful analemmas. They are generally roughly elliptical, teardrop shaped, or figure-8 in shape. Their shapes and dimensions depend on the parameters of the orbits. A subset of geosynchronous satellites are geostationary ones, which ideally have perfectly circular orbits, exactly in the Earth's equatorial plane. A geostationary satellite therefore ideally remains stationary relative to the Earth's surface, staying over a single point on the equator. No real satellite is exactly geostationary, so real ones trace small analemmas in the sky. Since geosynchronous satellites are close to the Earth, their analemmas vary substantially depending on the location of the observer on the Earth's surface.
The paraboloidal dishes that are used for radio communication with geosynchronous satellites often have to move so as to follow the satellite's daily movement around its analemma. The mechanisms that drive them must therefore be programmed with the parameters of the analemma. Exceptions are dishes that are used with (approximately) geostationary satellites, since these satellites appear to move so little that a fixed dish can function adequately at all times.
See also
References
- ^ Tutulemma: Solar Eclipse Analemma
- ^ Why Our Analemma Looks like a Figure 8
- ^ "The First Analemma Photo". National Geographic Magazine. December 28, 2010. Retrieved 2011-01-01.
- ^ "The Dark Days of Winter". at the USNO website
- ^ Other Analemmas
- ^ Why Our Analemma Looks like a Figure 8
External links
- Explanation of Analemmas of other planets
- Analemma Series from Sunrise to Sunset
- Earth Science Photo of the Day (2005-01-22)
- The Equation of Time and the Analemma — by Kieron Taylor
- The Use of the Analemma — from an inset from Bowles's New and Accurate Map of the World (1780)
- Figure-Eight in the Sky — contains link to a C program using a more accurate formula than most (particularly at high inclinations and eccentricities)
- The Analemma for Latitudinally-Challenged People — explains rising and setting analemmas as viewed from different latitudes and provides more depth on analemma.
- Analemma.com — dedicated to the analemma.
- Calculate and Chart the Analemma — a web site offered by a Fairfax County Public Schools planetarium that describes the analemma and also offers a downloadable spreadsheet that allows the user to experiment with analemmas of varying shapes.
- Analemma Sundial Applet — includes lots of reference charts.
- Analemmas — by Stephen Wolfram based on a program by Michael Trott, Wolfram Demonstrations Project.
- Analemma in Verse by Tad Dunne
- Astronomy Picture of the Day
- 2002-07-09 — Analemma
- 2003-03-20 — Sunrise Analemma
- 2004-06-21 — Analemma over Ancient Nemea
- 2005-07-13 — Analemma of the Moon
- 2006-12-23 — Analemma over the Temple of Olympian Zeus
- 2006-12-30 — Martian Analemma at Sagan Memorial Station (simulated)
- 2007-06-17 — Analemma over Ukraine
- 2007-12-04 — Analemma over New Jersey (film)
- 2008-12-21 — Analemma over the Porch of Maidens
- 2009-12-20 — Tutulemma: Solar Eclipse Analemma
- 2010-12-31 — Analemma 2010
