Talk:Euler's identity: Difference between revisions
m Signing comment by Becker-Sievert - "" |
/* Gauss' quote by a third rate mathematician |
||
| Line 11: | Line 11: | ||
:I came to ask what I think Wolfmankurd was asking. As it stands the sentence in the article is slightly ambiguous. Are first-class students expected to immediately see that it's beautiful, or immediately understand why it is true (i.e. immediately derive it in their heads)? [[User:Beorhtwulf|Beorhtwulf]] ([[User talk:Beorhtwulf|talk]]) 21:42, 28 February 2011 (UTC) |
:I came to ask what I think Wolfmankurd was asking. As it stands the sentence in the article is slightly ambiguous. Are first-class students expected to immediately see that it's beautiful, or immediately understand why it is true (i.e. immediately derive it in their heads)? [[User:Beorhtwulf|Beorhtwulf]] ([[User talk:Beorhtwulf|talk]]) 21:42, 28 February 2011 (UTC) |
||
It '''should''' be removed, because it a fabrication. Derbyshire is "quoting" Gauss, but no one properly quotes Derbyshire: |
|||
"Gauss is supposed to have said -- '''and I wouldn't put it past him''' -- that if this was not immediately apparent to you on being told it, you would never be a first-class mathematician." |
|||
Riemann Hypothesis historian Harold Edwards, in his review of Derbyshire's book writes: |
|||
If Gauss really said that, it was not his finest moment: but it sounds |
|||
more like something someone who lacked the prerequisites to even be a |
|||
second or third class mathematician, or even a first class forger, |
|||
might have put in his mouth.[[User:Doyleb23|doyleb23]] ([[User talk:Doyleb23|talk]]) 23:05, 12 October 2014 (UTC) |
|||
Mathematical Intelligencer;Winter2004, Vol. 26 Issue 1 |
|||
/* Gauss' quote by a third rate mathematician |
|||
==Quote== |
==Quote== |
||
Revision as of 23:05, 12 October 2014
| Mathematics B‑class Top‑priority | ||||||||||
| ||||||||||
Gauss' quote
Was he refering to the fact tht it's so beautiful When he said if it's not obvious then you'll never be a high-class amthematician?Wolfmankurd 08:06, 26 May 2006 (UTC)
Gauss quote is superfluous and only serves to discoure students who do not immediately comprehend. Perhaps needs to be removed. —Preceding unsigned comment added by 122.107.38.78 (talk) 13:49, 30 June 2009 (UTC)
- I came to ask what I think Wolfmankurd was asking. As it stands the sentence in the article is slightly ambiguous. Are first-class students expected to immediately see that it's beautiful, or immediately understand why it is true (i.e. immediately derive it in their heads)? Beorhtwulf (talk) 21:42, 28 February 2011 (UTC)
It should be removed, because it a fabrication. Derbyshire is "quoting" Gauss, but no one properly quotes Derbyshire: "Gauss is supposed to have said -- and I wouldn't put it past him -- that if this was not immediately apparent to you on being told it, you would never be a first-class mathematician." Riemann Hypothesis historian Harold Edwards, in his review of Derbyshire's book writes: If Gauss really said that, it was not his finest moment: but it sounds more like something someone who lacked the prerequisites to even be a second or third class mathematician, or even a first class forger, might have put in his mouth.doyleb23 (talk) 23:05, 12 October 2014 (UTC) Mathematical Intelligencer;Winter2004, Vol. 26 Issue 1
/* Gauss' quote by a third rate mathematician
Quote
Who said "The thought should console (as it bloody well ought) that e^(pi.i)+1=0?
Can't find it on Google. --Slashme 14:27, 21 November 2005 (UTC)
Feynman
At the end of The Feynman Lectures on Physics, vol. 1, chapter 22, he says: "We summarize with this, the most remarkable formula in mathematics: eiθ = cos θ + i sin θ. This is our jewel." I think the Feynman quote belongs on the Euler's formula page instead. Feynman also doesn't say in this book that it is remarkable for those reasons. Not that they aren't true; they just weren't said by Feynman.
- What exactly does Feynman mean by: "We summarize with this, the most remarkable formula in mathematics... This is our jewel."? This has been bugging me for a while. - 06:16, 22 February 2006 (UTC)
- I think, this formula links vast areas of mathematics - analysis, calculus, geometry, trigonometry, complex numbers, pi, e, 0, 1 - so deeply understanding it can help you on every occasion. As I study Informatik (something like computer science + information technology), I saw this formula very often in the last years, as for example the whole fourier analysis is based on this, which in turn is heavily used in the fields of engineering, computer graphics, digital audio and many others. --80.136.80.190 14:57, 23 July 2007 (UTC)
Feynman was not the first to use the term "the most remarkable formula in mathematics",this is the actual name by which mathematicians refer to the formula. Feynman most likely came across this description for the first time as a teen, while reading Calculus for the Practical Man
by J. E. Thompson.
Sacred geometry
I removed the following paragraph twice:
- There has been substantial debate in the philosophy of mathematics on the "real meaning" or "deep meaning" or even sacred geometry reflected by the Identity's relationship of key constants and operations (multiplication, exponentiation, addition, equality). Some assert that it describes cognitive properties of an embodied mind - and advocate a cognitive science of mathematics. At other extremes, some assert it represents rational social consensus of mathematicians, or is simply a fundamental fact of the physical universe, and that algebra itself is a natural consequence of its structure. If so, the formula would be more than simply remarkable - it would be 'divine'.
There has not been any substantial debate about sacred geometry related to this identity in the philosophy of mathematics. If I have missed the relevant literature, please point me to books, articles, conference presentations etc.
- have you read Tymoczko, 1998? "The traditional debate among philosophers of mathematics is whether there is an external mathematical reality, something out there to be discovered, or whether mathematics is the product of the human mind." ([Thomas Tymoczko]?)
- The way that traditional cultures refer to this "external mathematical reality" is with "sacred geometry" - whether or not mathematicians call it that.
- Of course that is the central question of the philosophy of mathematics. I asked specifically about references relating Euler's identity to the concept of "sacred geometry", and I am still waiting. I dispute the claim that "sacred geometry" is a commonly used term; EB doesn't list it at all. AxelBoldt
- do a google. you'll find a fair bit. The idea is somewhat contrary to Christian dogma, and occurs in Buddhism and Judaism and certain Hermetic beliefs - sometimes in Christian dogma it is associated with Satan, i.e. the pentagram, etc. One of the major sources of anti-semitism, actually, was the belief that Kabbalic rituals were "Satanic".
I just Googled for < "sacred geometry" Euler >. None of the resulting pages made any connection between the two, I'm afraid. Matthew Woodcraft
Furthermore, the paragraph presents the issue as "some assert..." — "at the other extreme....", as if those two were the only positions on the question, while in fact many other popular positions are left out.
- not much room... philosophy of mathematics gave some room to this.
- Well, then put a link to that page here and be done with it. AxelBoldt
- ok, but the "remarkable" nature of the identity was here before I edited it, and another paragraph to establish that this "remarkable" nature may have some other origins is important.
Algebra, Popper etc.
Algebra cannot be a natural consequence of this equation, because the equation records a fact about the complex numbers, while in algebra many
- there can be no such thing as "a fact about the complex numbers" since the complex numbers, and complex analysis, is a notational convenience to begin with. Your concept of reality is wrong. Fix it. ;-)
- You seem to think that the questions of the philosophy of mathematics have been finally answered by your little pet theory; you're wrong. There will never be consensus on those questions. You also don't seem to understand that there can be facts about notational conveniences, and that notational conveniences are part of reality. AxelBoldt
- no, there can't be facts about notation conveniences in the Popperian sense, as they are only falsifiable w.r.t. the rest of the notation - at best this is internal consistency. And no, notational conveniences are not part of "reality", they are part of colonialism or a certain paradigm of science at best. And no, again, there is no claim that the questions have been "answered by my little pet theory", as the theory that mathematics arises from the mind is very old, and the theory of mind arising in cognitive science is very deep... so it is *your* "little pet theory" that is under discussion, and its irrelevance in the face of cognitive science and philosophy of mathematics combined. As to your prediction that there will "never be consensus", that could be established merely by killing all over-educated people. To disprove this thesis, of course, you must kill them all yourself. Which brings us to the question of reasonable method...
- In other words, you believe that colonialism is not part of reality. Can I quote you on that, 24? AxelBoldt, Sunday, March 31, 2002
other fields, rings and groups are studied which have nothing whatsoever to do with the complex numbers and with Euler's identity. The "divine"
- that's foolish. How can fields, rings, and groups be totally independent of the operations of addition, multiplication, exponentation, and especially equality and equivalence? Euler's identity summarizes exactly these issues, and it is the way complex numbers "disappear" in the identity's resolution that makes it interesting. Also, fields rings and groups were more or less an invention of Galois - prior to that, Euler's identity summarized what was known. Suggestion, read cognitive science of mathematics and the references.
- Euler's indentity summarizes issues about addition, multiplication, exponentiation and equality of complex numbers. Just because we use the word "addition" in every abelian group doesn't mean that those additions share all properties of complex addition. Euler's identity says precisely nothing about the multiplication in the monster group. It cannot even be interpreted in any way in that context, because there's no exponential map and no addition and no zero element in that context. AxelBoldt
- why is *complex addition* the standard meaning? It isn't required for Euler's identity in particular, as the "e to the i pi" isn't a complex value according to Euler's formula but rather is "equal to minus one".
- But i is a complex number, and the exponential function ex is a function defined on the complex plane. Formulas don't just sit there, they are valid in a certain context. The context in which Euler's identity is valid is the complex number field.
- The "monster group" is a post-Eulerism that wouldn't exist if not for Galois's theory, which is not necessarily a guide to mathematics pre-Euler. I think the naive terms "plus" or "times" meant less to Euler than Galois... who may well have overly generalized them.
- So who cares about the subset of mathematics that was known at Euler's times? It has nothing to do with the discussion. You claim that Euler's identity underlies all of algebra, and the Monster group (and countless other examples) disprove that claim. AxelBoldt
connection is completely out of place and does also not relate to what was said earlier: if Euler's identity were just a social consensus, or a property of human cognition, then it would exactly not be divine. AxelBoldt, Sunday, March 31, 2002
- and if it were *neither* of those, it *would* be 'divine' in the same sense as the Planck length, etc,. - something part of the fundamental structure of the universe, unchangeable, etc.
- there is no need to use the loaded term "divine" for "unchangeable". Furthermore, again you are simplifying matters: Euler's identity would not have to be a fundamental structure of the universe; Platonists would argue that it necessarily holds in any possible universe. AxelBoldt
- fair enough... although a god or "divine" concept can be bound by a universe, and in Plato's time, to an even smaller entity. Although you are definitely splitting hairs here, as the difference between "the universe" and "any possible universe" is a distinction that not all theories of note recognize... why should there be more than one universe? There is value in deliberately loading the term, as it makes a connection to theology, where such matters have been more thoroughly discussed...
"The formula is a consequence of (or, viewed alternatively by some theories in the philosophy of mathematics, assumed in) Euler's formula " -- really? -- Tarquin 10:50 Jan 5, 2003 (UTC)
No, not really, but in the wonderful mind of user:24, which you can also see at work on this very talk page. AxelBoldt 02:05 Jan 8, 2003 (UTC)
Am I not correct in saying that "Euler's Identity" is shortform for "Euler is Identity", whilst "Eulers Identity" would be the correct way of putting it? I remember a very good educational video on Channel 4 (UK) back when I lived over there that explained the eccentricities of the apostrophy - it had a very addictive little tune that I haven't been able to get out of my head in the 15 or so years since I saw it.. but I'm sidetracking: This must be wrong, right?
--Smári 19:01, 1 Mar 2004 (UTC)
- You are incorrect. See: Apostrophe (punctuation) - Bevo 20:23, 1 Mar 2004 (UTC)
- Perfect. Then no need to worry. :) --Smári McCarthy 00:44, 2 Mar 2004 (UTC)
The last revision by 63.189.8.249 states: "It was however known long before to Chinese mathematicians." -- This is highly unlikely, as the concept of imaginary numbers was unknown to Chinese mathematicians at that time. (It may be a case of confusion about Chinese remainder theorem.) I have removed it for now, until a source is cited in favor of it. --Autrijus 18:06, 2004 Aug 15 (UTC)
- I would have to concur with the removal until a valid source/evidence can be found supporting the statement. - Taxman 18:32, Aug 16, 2004 (UTC)
Counter-intuitive?
I may only be speaking for myself, but I would find that is more counter-intuitive than when considering Euler's Identity.
- Erm. Why? As x grows large, you will have the reciprocal of a very large number... why is it a stretch to see this as approaching zero? DocSigma 05:02, 6 Feb 2005 (UTC)
I also consider this remark on the main page (e^\pi vs e^i\pi) quite ... useless, say. The simple insertion of "-" would also change the result, by twice the order of magnitude (speaking of ratios). (Funny... twice the order of magnitude, by putting the square of the exponent....)
I think it would be quite justified to suppress this annotation, — MFH: Talk 19:24, 10 May 2005 (UTC)
- I agree. -- Aleph4 16:47, 13 May 2005 (UTC)
- I was struck by the non sequitur about "counter-intuitive" myself. There is no viewpoint so naive as to make this property remarkable. I support removing the comment. 66.214.64.122 23:23, 29 May 2005 (UTC)
I agree that the paragraph especially as currently worded is not that useful. I will delete it. If someone wants to re-insert it they should look at earlier versions which I think are worded better. Paul August ☎ June 30, 2005 18:52 (UTC)
- I agree current wording is worse, but even the original wording is probably original research. All this "naive" non sense is from people who have a much more advanced understanding of the mathematics than those the comment referred to, and of course to them it is not counter intuitive. However, consistently when teaching students that do not have a firm grasp of imaginary numbers, they express surprise at the result. Since I don't have a published source to draw that from, I do admit I added it when I was not as stringent about the no original research bit. So go ahead and remove it. - Taxman Talk June 30, 2005 19:07 (UTC)
I finally deleted this paragraph. It slipped my mind till now. Paul August ☎ 06:22, July 15, 2005 (UTC)
He was talking about the strangeness regarding how converges to zero, but Euler's equation suggests a cyclical result. This becomes obvious when you see Euler's equation graphed on the axes of Real numbers, Complex numbers, and x. — Preceding unsigned comment added by 72.222.201.45 (talk) 16:20, 9 March 2012 (UTC)
Pi not constant?
The current version of the article says is a constant in a world which is Euclidean, or on small scales of non-Euclidean geometry otherwise, the ratio of the length of the circumference of circle to its diameter would not be a universal constant, i.e. the same for all circumferences).
While the "otherwise" part of the sentence is true, it does not talk about at all. You could as well define to be the infinite string 3.14159..., and then claim that is not constant if you use hexadecimal numbers. The string 3.14... does not define if you interpret it in hexadecimal, and the expression "ratio of circumference..." does not define if you talk about circles in a non-Euclidean plane.
-- Aleph4 16:47, 13 May 2005 (UTC)
Yes. The area or circumfrence of a circle on a minifold, for instance, is independent of pi, and depends if that manifold is a sphere, for isntance, on the magnitude of the curve of the shpere, or more particularly , to the ratio of of the size of the sphere to the size of the circle.He Who Is 22:40, 24 May 2006 (UTC)
true by definition?
Euler's identity is not "true by definition", because e(i pi) is not "defined" to be -1.
it just happens that if you define ez as the sum of the infinite series 1 + z + z2/2! + ... , and if you define sin(x) as the sum of the infinite series x - x3/3! + ... , and similarly cos(x) as 1 - z2/2! + ... (this is only one possibility of defining sin and cos on the complex numbers; other equivalent definitions are possible), then
- ei z = cos(z) + i sin(z)
is a consequence of that definition, and Euler's identity is again a corollary to that formula.
- Some analysis courses essentially introduce π as half the absolute of the period of the exponential function, which makes Euler's identity something that's easy to prove, if not immediately obvious. You could say it is by that definition of π that e2πi = 1. Prumpf 05:14, 4 August 2005 (UTC)
perceptions...
The first comment on perceptions, referring to 0, is currently not justified, as the formula is written as ...=-1 and not ...+1=0. (Only quite implicitely the 0 is present through the definition of "-".) — MFH: Talk 19:30, 10 May 2005 (UTC)
External links
On the page of Ian Henderson's "proof for the Layman", someone "complains" that this page is not even cited in the discussion. Let's fix this, by remarking that with the power series "formula" for e^x, sin x, cos x, Euler's formula and thus Euler's identity are trivial. All the geometric preliminaries do not contribute to this, they are only used for sin(pi)=0, but using for this the geometric definition of sin, while equality with the power series is not shown.
The other external link to a proof (.../jos/...) (which in fact concerns rather Euler's formula and thus should be moved there) deserves about the same critics (with algebraic instead of geometric preliminaries), IMHO, the crucial step being the comparision of the derivatives in zero on the last page (implicitely (via Taylor series) admitting that both sides are analytic). — MFH: Talk 21:25, 10 May 2005 (UTC)
- I don't think those PhysicsWeb Links work, at least they didn't for me... Guerberj (talk) 21:03, 7 August 2009 (UTC)
2=0??
theres a worrying result you get with some simple manipulation of this identity:
e^(i.pi) = -1
e^ 2(i.pi) = 1
ln e^2 (i.pi) = ln 1 ln 1 = 0
2.i.pi= 0
i does not equal 0 pi does not equal 0
therefore 2=0??
i cannot argue with the way the identity is reached, but surely we should be explaining why these results can come after. —The preceding unsigned comment was added by Edallen399 (talk • contribs) 18:07, December 21, 2005 (UTC).
- for all real
But
Paul August ☎ 01:35, 22 December 2005 (UTC)
For more information, you might want to review the following articles:
-- 24.218.218.28 01:48, 22 December 2005 (UTC)
I spotted this and it seems quite interesting. I've looked at all those aarticles, and nothing was explained. Is not the very definition of a logarithm of x to base b the exponent that brings b to x? It seems counter-intuitive that the very concept that gave rise to the idea of a logarithm would collapse anywhere, even over the complexes. He Who Is 02:08, 11 June 2006 (UTC)
- Yes, this is the trouble you can get into with the definite article. The very definition of a logarithm of x to base b is an exponent that brings b to x; but there's more than one! Melchoir 02:17, 11 June 2006 (UTC)
You can't take the ln of a complex number like that. You have to use the identity.
So:
Which makes sense because you just rotated -1 by 180 degrees, and is the same as —Preceding unsigned comment added by 99.253.93.99 (talk) 04:49, 11 June 2009 (UTC)
Does this article need an image?
I cooked one up in about 30 seconds. Is it original research, or do I need a source? Is it appropriate at all? Melchoir 02:51, 28 January 2006 (UTC)
- I like the image! Maybe the (black) line should consist of two directed lines? That is, the semi-circle might have an arrow head (at -1, pointing down) and the straight-line segment might also have an arrow head (at 0, pointing right). Not sure--just an idea. —Preceding unsigned comment added by 81.103.219.85 (talk • contribs) 16:12, January 29, 2006 (UTC)
- I like the image too, but it is a little by cryptic for the uninitiated. I think the idea of adding arrows as suggested is good. You might also want to add some context, like coordinates, axes, the unit circle, the origin, etc. -- Metacomet 16:15, 29 January 2006 (UTC)
- Ideally, I'd like to keep the diagram being interpretable by aliens from another galaxy. (Yup, I know that's not really constructive.) —Preceding unsigned comment added by 81.103.219.85 (talk • contribs) 16:33, January 29, 2006 (UTC)
- Another brilliant idea from the community. -- Metacomet 16:38, 29 January 2006 (UTC)
- My point was to keep the diagram language-independent. This would, for example, exclude numerals. Be sarcastic if you want, but I think that art is preferable that way. And greatly more so here, when dealing with an equation that seems to hold some universal transcendent truth. Think about it. —Preceding unsigned comment added by 81.103.219.85 (talk • contribs) 19:00, January 29, 2006 (UTC)
- Actually, one of the reasons I think this article needs an image is to demonstrate that Euler's identity isn't so "transcendent" after all; it's just telling you that the length of the unit semicircle is pi, which really shouldn't come as a surprise. Anyway, I'll try putting in a bit more context. Melchoir 22:33, 29 January 2006 (UTC)
- Woo. Melchoir 22:45, 29 January 2006 (UTC)
- The revised image is a big improvement. Well done. -- Metacomet 15:53, 31 January 2006 (UTC)
I think it is still too cryptic. I would suggest adding a line segment going from the origin to the circle, making an acute angle with the horizontal axis, labled with a , and its point of intersection on the circle labled Paul August ☎ 16:34, 31 January 2006 (UTC)
- I think those are good suggestions. It might also make sense to add either a caption to the image, or some descriptive prose to the article that explains the meaning of the diagram. -- Metacomet 17:38, 31 January 2006 (UTC)
- I much like the image as it is. Adding an angle like that is extra confusion. Keep it simple. Also, the more complicated image that you propose is already in the article for Euler's formula.
- I do think that the identity shows something universal, as explained in the section entitled "Perceptions of the identity". Including Greek letters tends to go against that. —Preceding unsigned comment added by 81.103.219.85 (talk • contribs) 19:41, January 29, 2006 (UTC)
- WP is an encyclopedia, not a book on transcendental philosophy. -- Metacomet 19:52, 31 January 2006 (UTC)
- Getting back on topic, I didn't want to label the general point, e^i phi, because it's already been done at Euler's formula. It might be more appropriate to simply include that image in the Derivation section. Melchoir 20:53, 31 January 2006 (UTC)
- Come to think of it, that's what I've done. Melchoir 20:55, 31 January 2006 (UTC)
- WP is an encyclopedia, not a book on transcendental philosophy. -- Metacomet 19:52, 31 January 2006 (UTC)
- Ok that's fine now. Paul August ☎ 21:28, 31 January 2006 (UTC)
September
As a math novice, the diagram (the first one) needs some explanation. This article needs to discuss what Euler's identity is about in a large context. Does it have applications? Was it just happened upon? What does all that stuff with the circle mean? -- Reid 03:49, 15 September 2006 (UTC)
- I've added a caption. The larger context of the identity is, unfortunately, that it looks pretty. I doubt that Euler ever wrote it in the form that gets so much press. But it's related to the historically important identity
- which is less photogenic but quite significant because before Euler came up with it, no one knew what the logarithms of negative numbers were. Or, to be more precise, different mathematicians had different answers. I don't have a source in front of me, but I know that one exists... somewhere! Melchoir 04:10, 15 September 2006 (UTC)
- The image would perhaps be easier to understand if the axes were labeled. Jclerman 08:08, 15 September 2006 (UTC)
- No problem, but can you be a little more specific? Melchoir 17:04, 15 September 2006 (UTC)
- Without labels on the horizontal and vertical axes, simple (lay)users might think they are x- and y-axis, while more complex users might guess they are real- and imaginary-axis. The caption mentions i and z but doesn't relate them to the image. Jclerman 17:24, 15 September 2006 (UTC)
- Okay, I can put in something to that effect in a day or so. Melchoir 03:39, 16 September 2006 (UTC)
Good summary folks - I think that the relevant parts of this discussion should be worked into the text of the article.Reid 01:54, 19 October 2006 (UTC)
Derivation
The "derivation" given here involves just one logical step, substitution. To expand on the substitution is not only unnecessary, it's a red herring; the real work is being done by Euler's formula. Melchoir 09:13, 30 January 2006 (UTC)
- This is true for people who are comfortable with high school mathematics. The previous derivation, though, could be partially understood even by people who can do little more than arithmetic and syntactic substitution. So I prefer the previous derivation: let everyone feel that they have understood a little bit.
- In the current version, I've kept all the information; if you don't know the values of cos and sin at pi, they're right there. Melchoir 16:57, 30 January 2006 (UTC)
- I'm pretty sure that the earlier version is easier for people without a mathematics background. See too "The Science of Scientific Writing" by Gopen & Swan (it's on the web).
- I still don't understand what was wrong with this version. Why do we have to display every formula? The choice of which equations to display and which to leave in-line is an important writing tool; it helps guide the reader. From this we get this. In the current version, displaying everything breaks the continuity of the argument. And why isn't x = π being displayed? It's an equation, isn't it? Melchoir 21:02, 31 January 2006 (UTC)
- Metacomet, will you explain why you said "equations should not be in-line with prose" and also why you think x = π is an exception? I most prefer this version of the derivation.
Perceptions of the identity, recently
- We can't claim "Many people find..." without a source.
- Crowing the word "fundamental" four times is too much. Even once makes me uneasy.
- Even worse, "arguably the most fundamental"?
- "the study of logarithms"? Who actually studies logarithms?
- "Furthermore, an equation with zero on one side is the most fundamental relation in mathematics"? Please, let's not mistake conventions for truths.
I'm reverting back. Melchoir 09:00, 5 February 2006 (UTC)
- 1. Come on--does "many people find the moon inspiring" also need a source? Go to any pure math department and ask people. In any case, the quote from Benjamin Peirce gives cited support.
- 2. I think think that the statements are justified. If you disagree, will you explain why?
- 3. (Ditto.)
- 4. Agreed. This was copied from an earlier text.
- 5. This was also copied from an earlier text. Surely, though, this is more than convention! Perhaps you might argue that equality is the most fundamental relation. Okay, but if you have a zero, then you can almost always cast the equality as ___ = 0, which seems more fundamental.
Daphne A 14:14, 5 February 2006 (UTC)
- Thanks for responding! I'll be glad to explain further...
- 1. Yes, the moon bit also needs a source, and Pierce is not "many people". I'm personally aware that plenty of people find the identity beautiful, but there ought to be some support for that. If you can find a source, it would really improve the article (hint hint). For now, it's best to avoid unsupported hedging.
- 2,3,4. It used to say "three most fundamental functions in arithmetic". Incrementation is more "fundamental" than any of those, and historically, among the fundamental functions of arithmetic people have listed numeration, squaring, cubing, extracting roots, and halving as examples-- but IIRC, not exponentiation. It used to say "arguably the most fundamental mathematical constants". Why should that list include 1 and i but not −1? Where's infinity? How about the square root of 2? Going to history again, these were important concepts long before e was even discovered. It used to say "fundamental in the study of logarithms"; I think you agree with me here.
- 5. Finally, it used to say "Furthermore, an equation with zero on one side is the most fundamental relation in mathematics". I don't think there's a most fundamental relation, but if there is, it's probably equality, morphism, implication, or set membership. Equations with zero on one side don't even make sense in mathematical contexts without a zero, and that's a huge chunk of mathematics. You can't do it "almost always"; in fact, it's forbidden whenever you don't have a subtraction operation. For examples, multiplicative groups, the natural numbers, the cardinal numbers, the ordinal numbers...
- Okay? Melchoir 19:52, 5 February 2006 (UTC)
- I've made some revisions, but I think that there should be more. The description of e, for example, is almost vacuous. Taking your points in turn....
- 1. I don't agree with you, but I've not changed this in the revision. Peirce would not seem to be speaking for himself alone. And you say that you know people who effectively agree with him (as I do). A quick search turned up a claim by Contance Reid, in From Zero To Infinity, that the identity is "the most famous formula in all mathemetics". (I'm not sure about that; e.g. the formula for the area of a triangle is more widely known.) In any case, I think that the present wording is stronger than (i.e. semantically implies) the "Many people ..." wording; if so, then surely the stronger wording would need a citation! Writing "Many people ..." seems more accurate and, to me, reads better. The only poll that I've seen was done by Physics World, but this only got 120 responses:
- http://physicsweb.org/articles/world/17/10/2/1
- (Euler's identity seems to have tied for first, but the other first, Maxwell's equations, aren't pure math).
- 2,3,4. Okay. Your point about increment is intriguing, since the identity does include that. Should this be mentioned?
- 5. Is there a branch of mathematical analysis that does not have zero?
I like your latest revision a lot better; it retains a certain sense of wonder while keeping its head out of the clouds. Moving to specifics...
- 1. Please add your sources to the article! If you're worried about formatting and presentation, I can help with that. As for the poll, a sample of 120 is better than a sample of 2; you should put it in. You may doubt Reid, and so do I, but his quote is still relevant; that should go in too.
- 2,3,4. No, it shouldn't. The statement "Euler's identity does not include X" is not a challenge.
- 5. You could argue that the Greeks did a kind of analysis without admitting zero. I'm not sure about that, but it sounds right. More importantly, is there any hope of ever producing a reliable source that says "an equation with zero on one side is the most fundamental relation in Y"? I think not. It's your opinion, and that's fine. I don't feel a strong need to convince you otherwise, but it doesn't belong in an encyclopedia article. That sentence is the only bit that still does have its head in the clouds.
I'll edit that last point to make it more realistic. Melchoir 19:46, 6 February 2006 (UTC)
I've removed the explanations of 0 and 1. Everyone knows what these are, and it is incorrect to suggest (as the prior text seemed to do) that 1 is nothing more than a multiplicative unit.
I've also put back in a sentence about equations with zero on one side. I think that there should be something about this, but the wording could likely be improved.
As Melchoir says, the trick is to retain a sense of wonder without getting carried away. I don't find this easy! I'm still not sure that including the Reid quote is a great idea (as per above), but have included it here.
Daphne A 09:37, 9 March 2006 (UTC)
History
The article currently claims that the identity is from the Introductio. I was curious about how Euler wrote it, so I found a translation in the library, and I don't see the identity; Euler seems more interested with the general formula. What's the deal? Melchoir 20:11, 6 February 2006 (UTC)
- I've added a new section that briefly discusses this; see what you think. TheSeven 22:37, 5 March 2007 (UTC)
- Thanks! That's a great column. It's a shame that we don't have more information, but what you wrote is a lot better than the alternative. Melchoir 23:03, 5 March 2007 (UTC)
It would be good to show not only the arguably first instance of the formula, but the actual, documented first published version of it. That's actually why I came looking for.... /user David James, no account — Preceding unsigned comment added by 107.205.74.23 (talk) 13:24, 22 April 2014 (UTC)
We need a proof
I think a good improvement to the page would be to include a calculus based proof for e^(pi*i) + 1 = 0. Unfortunately, I'm not yet skilled enough to do this myself. - Christopher 22:49, 9 February 2006 (UTC)
- I am not sure you can prove this identity with calculus. The identity is in fact a direct consequence of Euler's formula, which states:
- where
- is the imaginary unit.
- So it is really a matter of whether you can prove Euler's formula. It is not too difficult to prove using the Taylor series expansions for the exponential, sine, and cosine functions, and the fact that
- This proof is actually included in the article for Euler's formula – see Proof.
- Once you prove Euler's formula, then Euler's identity follows quite readily. In fact, the derivation is already included in this article.
First, I don't like to see - the expression can maybe stand for any solution to x²=-1 — yet, where to search this solution before already "having" i ? — but anyway, IF this equation has a solution, it is not unique, thus it cannot be used to define what i is.
Second, to the calculus proof: it depends of what you mean by calculus (series should be part of), and what you mean by "ex". I you define the latter by the power series (which is imho the best definition) then writing explicitely the first 5 terms clearly shows you how grouping together real and imaginary terms make up the power series of (-)cos(π) and sin(π), thus (this shows a way to write a proof for) the result. — MFH:Talk 16:47, 27 March 2006 (UTC)
Actually, technically has no solutions, real or complex, since the radical, , points to the principal, or positive squared root of the radicand. does not fit this description, since a positive number is defined as any number x|x>0. i is complex, and thus incomprable to real numbers, making it neither positive nor negative. Therefore, should be written , as it was before. 71.65.9.68 01:53, 18 May 2006 (UTC)He Who Is 01:54, 18 May 2006 (UTC)
- Of course a real definition isn't going to work outside the reals. See Square root#Square roots of negative and complex numbers for the square root of negative one. Melchoir 04:58, 18 May 2006 (UTC)
- I completely share the sentiment of MFH and You Who Are: Only a nonnegative real number belongs under a square root sign if the expression is intended to be single-valued.
- I just checked the Wikipedia article Square roots mentioned by Melchoir (Melchoir?), and the section "Square roots of complex numbers" contains some utter nonsense, especially this passage:
- Similarly to the real numbers, we say the principal square root of −1 is i, or more generally, if x is any positive number, then the principal square root of −x is
- because
- Similarly to the real numbers, we say the principal square root of −1 is i, or more generally, if x is any positive number, then the principal square root of −x is
- Above all, the phrase "the principal square root of -1" is devoid of mathematical meaning. That is because there is no mathematical way whatsoever to distinguish between i and -i.Daqu (talk) 09:39, 30 January 2008 (UTC)
Derbyshire
I was reformatting the footnotes in this article when I noticed that one of the notes reads simply "Derbyshire". Could someone who has a clue please make sense of this? --Slashme 11:45, 23 May 2006 (UTC)
- See the references! But you are right, there should have been a page number; this is now included. —Daphne A 11:02, 1 June 2006 (UTC)
PNG > SVG
I just noticed the replacement of PNGs by SVGs. I was rather surprised that my browser correctly displays these. To me it's a completely unknown format, and I'm not sure if all visitors can visualize this format without problems. Is it a good idea to switch from well established formats by "bleeding edge" latest developments? (Personally, I have nothing against promoting file formats supported by all browsers except for IE... ;-) — MFH:Talk 17:47, 29 May 2006 (UTC)
- The SVG format doesn't actually appear on your browser; it's translated to PNG by MediaWiki first. Try opening one of the thumbnails in a new window and you'll see what I mean. Melchoir 14:37, 1 June 2006 (UTC)
Estimate of e?
Can we give an estimate of e to several significant digits? At page pi we have an estimate of that number to at least 5 digits... can we have the same here? 4.242.108.238 05:24, 19 December 2006 (UTC)
- 2.71828, from the article or "page" e. Jclerman 06:35, 19 December 2006 (UTC)
the diagram
When I look at the first, simpler diagram, I feel I can almost understand the equation, which is wonderful. But then I read "Starting at the multiplicative identity z = 1" I go "Hunh?" The equation doesn't have a z in it (I can see how the point on the diagram is 1 (0i)). And I've never seen the word "multiplicative" in my life before. I think I know what it means, but I can't imagine what a "multiplicative identity" might be in that sense. Can someone please clarify? --Hugh7 00:12, 23 January 2007 (UTC)
- How about this? Melchoir 01:33, 23 January 2007 (UTC)
- Reminder (from earlier comments):
Jclerman 02:05, 23 January 2007 (UTC)
- The image would perhaps be easier to understand if the axes were labeled. Jclerman 08:08, 15 September 2006 (UTC)
- No problem, but can you be a little more specific? Melchoir 17:04, 15 September 2006 (UTC)
- Without labels on the horizontal and vertical axes, simple (lay)users might think they are x- and y-axis, while more complex (pun iuntended)users might guess they are real- and imaginary-axis. The caption mentions i and z but doesn't relate them to the image. Jclerman 17:24, 15 September 2006 (UTC)
- Okay, I can put in something to that effect in a day or so. Melchoir 03:39, 16 September 2006 (UTC)
Whoops! Yeah, I forgot about that entirely. Sorry! Uh… maybe it's no longer necessary? Melchoir 02:16, 23 January 2007 (UTC)
Many thanks. And I take it the more complex ;) users' guess is correct, so that the second diagram is a more general case of the first? -- Hugh7 23:39, 26 January 2007 (UTC)
ubiquitous in Euclidean geometry
The article says that
- The number π, which is ubiquitous in trigonometry, Euclidean geometry, and mathematical analysis.
I think this is not correct. Euclidean geometry only talks about constructible numbers, doesn't it? --Aleph4 11:25, 26 April 2007 (UTC)
Please elaborate. Does Euclidean geometry talk about the length of a circumference of a circle? Certainly Euclid did not talk about it, and neither does the first order theory in which Euclidean geometry can be expressed (according to our article Euclidean geometry). --Aleph4 16:35, 26 April 2007 (UTC)
- It does if you take "Euclidean geometry" to mean "the geometry of Euclidean space", in contrast with "non-Euclidean geometry". On the other hand, is useful in non-Euclidean geometry as well, so "the geometry in Elements" may be a more accurate interpretation... (and in that case, I must apologize for responding too quickly) Fredrik Johansson 23:53, 28 April 2007 (UTC)
I see, "geometry of Euclidean spaces" then. Should trigonometry now be omitted as a subset of geometry? --Aleph4 09:55, 30 April 2007 (UTC)
Practical Applications
Can I ask- what is the point in i? Why did anyone bother to make up any of this? Euler's identity, however 'beautiful' it is, doesn't help anyone do anything. Please give a section on applications. —Preceding unsigned comment added by Reagar (talk • contribs)
- Euler's identity is a basic result for complex numbers. There's a section on applications of complex numbers in the complex number article. --Zundark 21:11, 7 May 2007 (UTC)
Image caption - velocity
The image caption says "Starting at e0 = 1, travelling at the velocity i relative to one's position for the length of time π, and adding 1, one arrives at 0." Is this correct. How can the velocity be imaginary? Vints 06:53, 14 June 2007 (UTC)
- When you live in the complex plane, your position, velocity, acceleration, etc. are all complex numbers. Melchoir 19:48, 14 June 2007 (UTC)
- So if r = eiφ, r' = i*eiφ = i*(cos(φ)+i*sin(φ)), and if you change coordinate system this velocity is i relative to your own position? I mistook "one's" to mean 1's. Vints 06:19, 15 June 2007 (UTC)
- Yeah, I don't know what would be a better wording -- "your" doesn't really work in an encyclopedia. I don't know what you mean by changing coordinate system... Melchoir 18:00, 15 June 2007 (UTC)
- The velocity r' = i*eiφ is only equal to i when φ=0. For example when φ=π/2 the velocity is -1. So what does "relative to one's position" mean? Vints 08:30, 17 June 2007 (UTC)
- It just means "multiplied by", if you want it in algebraic terms rather than geometric. Melchoir 18:11, 18 June 2007 (UTC)
Simplified identity
I was taught Euler's identity as the simpler . Is really more common? And does anyone else think the equation loses some beauty when it so obviously simplifiable? Foobaz·o< 05:55, 12 July 2007 (UTC)
- I agree that the less verbose form is preferable, and probably more common. The article used until an anon user changed it on 26 January 2006 without even an edit summary to explain the reasoning. We should just change it back, but there some adjustments that need to be made to the rest of the text to make it consistent. --Zundark 09:05, 12 July 2007 (UTC)
- I strongly prefer the current form. That is where much of the beauty comes from: this is discussed in the text at fair length. Additionally most (all?) of the references use that form. TheSeven 20:39, 16 July 2007 (UTC)
- You're right, most google hits for the identity use the same form as the article. It appears my perception of beauty is in the minority. Foobaz·o< 14:53, 17 July 2007 (UTC)
Pi is wrong
Yes, this is because pi is wrong. The true Euler's identity is
where τ = 2π. The complex exponential of the circle constant is unity.
Adding 1 to both sides of the pi-based identity to make it "beautiful" is purely arbitrary, and I don't know why mathematicians stand for it. "OMG 0=1+2-3 is so beaautiful! It contains all the first numbers in order and also equals and minus and plus!"
I'm surprised there's no criticism in this article. You mathematicians are ok with this equation being described with mystical BS like "it's absolutely paradoxical", and "we cannot understand it"? You don't think this is a misrepresentation that reflects poorly on your field? — Preceding unsigned comment added by 71.167.69.162 (talk) 15:51, 26 May 2011 (UTC)
- I am a big fan of τ. - 193.84.186.81 (talk) 09:40, 15 February 2012 (UTC)
- Here is wisdom.Mykhal (talk) 12:50, 7 October 2012 (UTC)
Meaning
I'd like to see a sentence or two describing the mathematical meaning (interpretation) of the equation, i.e., something to answer the question "that's nice, but what does it mean?". Something simple would suffice, e.g., The formula represents a rotation in the complex plane of the unit vector through an angle of π radians (180°). — Loadmaster 18:23, 16 August 2007 (UTC)
Does it make 1 = -1 ?
Rearranging gives :
Square both Sides :
Take a natural log of both sides :
Substitude into the original equation :
How can this be?
—Preceding unsigned comment added by Pi (talk • contribs) 20 November 2007
- The problem is that log is multivalued for complex numbers. If you use the principal value, then . --Zundark (talk) 10:12, 20 November 2007 (UTC)
- This may just make me sound stupid, but doesn't squaring something generally affect the domain of the result? 174.18.154.104 (talk) 23:52, 16 February 2010 (UTC)
The formula is wrong
No matter how widely it has been quoted in the form or , the formula is technically incorrect since exponentiation of complex numbers is multi-valued (and that includes real numbers).Daqu (talk) 09:18, 30 January 2008 (UTC)
- I'm not sure what exactly you're trying to say, but the function e^z is not multi-valued. Melchoir (talk) 01:33, 31 January 2008 (UTC)
- What I am saying is that exponentiation of complex numbers is multi-valued, including e raised to the power z, which is what the notation means.
- (In fact, takes all values of the form ·, where k runs through all integers.)
- The single-valued function you are referring to is often, but incorrectly, referred to as . The correct notation for this single-valued function is . That's what I'm saying.Daqu (talk) 17:53, 25 February 2008 (UTC)
- Can you provide a source that agrees that is different than ? I believe they're just alternate notations for the same thing. Foobaz·o< 18:22, 25 February 2008 (UTC)
- The expression you wrote can be simplified. Melchoir (talk) 17:05, 26 April 2008 (UTC)
- ...Actually, no it can't; I must have been thinking of something else. Melchoir (talk) 23:47, 27 April 2008 (UTC)
- "(In fact, takes all values of the form ·, where k runs through all integers.)"
Those are all the same value though (namely, -1). It's the logarithm which is multivalued, not . 92.29.43.11 (talk) 16:50, 4 July 2009 (UTC)
- "(In fact, takes all values of the form ·, where k runs through all integers.)"
- (I'm not advocating Daqu's position, as ez is simply a common notation for the exp(z) function, it's not supposed to be read as binary exponentiation. However: ) No, they are not. Pluging z = iπ into the formula gives you eiπ = −(exp(−2π2))k for any integer k. In general, ab (for complex a ≠ 0 and b) has a unique value if and only if b is an integer. — Emil J. 14:26, 6 July 2009 (UTC)
Peirce's quote
The article says:
After proving the identity in a lecture, Benjamin Peirce, a noted nineteenth century mathematician and Harvard professor, said, "It is absolutely paradoxical; we cannot understand it, and we don't know what it means, but we have proved it, and therefore we know it must be the truth."
I have a complex variables text in front of me and it has exact same quote, but it says it was B.O. Peirce's math teacher who said it and not after proving the Euler's identity, but after finding sqrt(exp(pi)) to be the principal value for i^(1/i) i.e. i-th root of i. It's on p.130 of Complex Variables With Applications by A. David Wunsch, 3rd edition ISBN: 9780201756098 ------Added Tue, Apr 15 2008 —Preceding unsigned comment added by 99.225.240.84 (talk) 00:59, 16 April 2008 (UTC)
Pure nonsense

Look at this diagram (taken from the article). Well, quite a good piece of poetry, but... How can one "travel at the velocity i"? Does anybody understand, what does it mean? A kind of pure nonsense, I'm afraid, although copied to 15 wikis... Olaf m (talk) 14:56, 26 April 2008 (UTC)
- The function e^it is the solution to the differential equation
- Melchoir (talk) 17:04, 26 April 2008 (UTC)
- I see, by "i relative to one's position" you mean "i multiplied by one's position". IMHO the story is rather hard to understand in the current form. Maybe a kind of animation would be more understandable.
- Yet, I cannot see any reason for inclusion of this story in the article. The beauty of Euler's identity is not linked to being solution of a differential equation, or every differential equation would generate a piece of art. The Euler's identity is the unique relation linking five most important mathematical constants, not just a tale of a point traveling and jumping.
- May I ask of sources for this story? Is it your OR? Olaf m (talk) 20:59, 26 April 2008 (UTC)
- I have no sources, but it's a completely straightforward reading of the sentence "e^i pi + 1 = 0". If you think the exponential function is more about manipulating the number e than it is about solving the differential equation x' = x, that's... unfortunate.
- I will reword the relative part though. Melchoir (talk) 21:26, 26 April 2008 (UTC)
- It makes sense to me as well, and should be kept. It is worded confusingly, but I can't think of a better way to word it so concisely. The key here is the differential properties of exponential functions, as Melchior says. The derivative of is equal to everywhere, which makes it increase exponentially. The derivative of is , which means the derivative is now at right angles to the value, because multiplication by is a 90° rotation in the complex plane. Something traveling at right angles to its position forms a circle around the origin. If you have a nice calculator, you can check for yourself that for all , as you would expect from a circle in the complex plane. I hope this helps explain. Foobaz·o< 22:40, 26 April 2008 (UTC)
- "The Euler's identity is the unique relation linking five most important mathematical constants" - this interpretation is, at best, superficial. Putting aside the question of what "most important" means, the formula is not unique. Just to throw together one random example, contains the same five constants. This formula is unremarkable because of the trivial nature of raising to the zeroth power. Euler's identity is remarkable/beautiful because all the actions are nontrivial. When you interpret the actions geometrically (using the differential property of the exponential function), you understand what Euler's identity really says. I second Melchoir's sentence "If you think...". Fredrik Johansson 00:06, 27 April 2008 (UTC)
- IMHO, the picture and the explanation may be misleading, the phrase traveling at velocity relative to one's position is more than confusing and ASAIK there are no printed sources using explanations of that sort (OR....) But the fundamental problem here is that the identity is more about rotation than "a rotation followed by translation". Stotr (talk) 14:47, 27 April 2008 (UTC)
- Note that the text has now been changed: ...traveling at velocity i multiplied by one's position for the length of time π, which is an improvement over the text we have been discussing so far. (An improvement in mathematical correctness, not neceessarily in readability.)
- For me, the intuitive notion of velocity is real-valued (or vector-valued for a real vector space), but not complex-valued. For real numbers, the equation can be interpreted as "if you start at F(0) at time 0, and travel with velocity F' until time a, then you reach the point F(a)".
- If you plug in the complex function , you get the statement in the caption of our diagram. However, I think that the notion of a complex velocity does not have the same intuitive appeal, and the translation from 1-dimensional complex geometry to 2-dimensional real geometry is completely left to the reader.
- Furthermore, the formulation "and adding 1, one arrives at 0" makes matters even less intuitive. "one arrives at -1" would be clearer. (Or should I say "would subtract some unclarity"?:-) I think we agree that the formulations e^i.pi+1=0 and e^i.pi=-1 are obviously equivalent; if we want to render this equality into text, we should choose the version that gives, among all the possible texts, the most readable one.
- Aleph4 (talk) 16:50, 27 April 2008 (UTC)
- That's not quite the thrust of the caption. Your formula is integrating a function of t, when it should be "integrating" a function of z. The caption is attempting to start from the base meaning of the exponential function: here is this vector field, now follow it! Whereas your interpretation already knows what e^it does and works backwards.
- In principle I'm not opposed to ending the picture at -1, since I prefer that form of the identity anyway. However, I think many people would disagree. And since the statement of the identity in the text of the intro section isn't likely to change, it's better if the diagram is in sync with the text. Melchoir (talk) 20:01, 27 April 2008 (UTC)
- I agree with you that the constant e is far less important than the function e^x (or e^ix), both in this formula and in the rest of mathematics.
- I don't see your point about the integration variable, t vs z. How we call this variable is irrelevant, at least mathematically. (Psychologically there is a difference that I was talking about a real variable first, then generalizing.)
- I don't think that there is a "base meaning" of the exponential function. Solution of y'=y, inverse of log = integral 1/x, series expansion, (1+1/n)^nx, and probably many more equivalent definitions can all claim to be the "real" definition of the exponential function.
- As I understand you, you are considering the real vector field (-y,x) and use the (obvious, I agree) fact that the only curves (x,y) with (x',y')=(-y,x) are circles. (Plus, the curve through (1,0) is parametrized by arclength.) Again, this is a 2-dimensional real intuition, but the equation really (i.e., read in the most natural way) expresses a 1-dimensional complex fact.
- --Aleph4 (talk) 22:30, 27 April 2008 (UTC)
- I think we're mostly on the same page, except with integration variables. I didn't mean just replacing t with z, I meant reparameterizing the integral. Consider your formula:
- This hasn't simplified anything, since there's still a complex exponential on the right-hand side. By "integrating" a function of z, I meant something like this:
- This is getting closer conceptually, but mathematically it's high on crack, hence the quote marks around "integrating". (Please don't take the above formula too seriously.)
- Vector fields vs. first-order ODEs, R^2 vs. C^1... we can gloss over these distinctions for a simple diagram. However, I will dispute that I am "using" the fact that solutions lie on circles. Of course this observation is invaluable in a proof, and it's strongly suggested by the diagram. But we don't have to prove it in order to state the fact that following a certain vector field for a certain length of time lands you at -1. Melchoir (talk) 23:26, 27 April 2008 (UTC)
- I think we're mostly on the same page, except with integration variables. I didn't mean just replacing t with z, I meant reparameterizing the integral. Consider your formula:
- I am not sure if anybody noticed, but there is some discussion here about interpretation and correctness of a small graph that should be used only if it helps the reader to grasp the concept. The graph is not relevant for the formula (though otherwise may be a subject to the interpretation) and if it does not clearly help the reader then it should not be here... Also, can you cite any published work with a picture like this? (I have looked through several Complex Analysis texts and I do not see anything even remotely resembling this picture.) There should be no place for OR in wikipedia, and as the matters stand it at the moment is an OR of a sort. Stotr (talk) 13:25, 28 April 2008 (UTC)
- I agree with Stotr that our discussion really shows that the picture is not appropriate for the article, as it does not clarify the concept at all; as I mentioned above, the concept of complex velocity is so far removed from a non-mathematician's intuition that it is not helpful. (As for mathematicians who read this page, they will anyway know what e^ix is.)
- Concerning OR: The truth is that many of our mathematical articles contain unsourced claims; but I think this is usually supported by a tacit agreement between the editors that a source could easily be found, so (unlike our case) it is not challenged as OR.
- --Aleph4 (talk) 14:06, 28 April 2008 (UTC)
- The graph is relevant to the identity, and it does help some readers. This article is visited some 500 times per day, whereas we have three people on the talk page who don't like it and three who do. Hardly proof either way.
- Also concerning OR: There is another tacit agreement that images require extra leeway. I would be surprised if the diagram were found in a textbook aimed at an expert audience, even more than I'd be surprised if a randomly chosen sentence from the article turned out to be a quotation. Now think about the content: it's half a unit circle with an arrow on the end for crying out loud! We're not talking about a conceptual revolution over here. Melchoir (talk) 16:52, 28 April 2008 (UTC)
- Apparently you do not want to understand what the other side is saying. Fine. I can only hope that my students will not use this partiucular page as they will end up only more confused. Stotr (talk) 17:09, 28 April 2008 (UTC)
- I've identified three constructive comments in this whole thread, one of which I acted on. Beyond that, "the other side" has spent a lot of energy yelling about how they think the diagram is unhelpful. I disagree and have said as much. If I've missed a more subtle point you wanted to make, you can certainly help me out with a less strident and more focused delivery. Melchoir (talk) 18:19, 28 April 2008 (UTC)
- Apparently you do not want to understand what the other side is saying. Fine. I can only hope that my students will not use this partiucular page as they will end up only more confused. Stotr (talk) 17:09, 28 April 2008 (UTC)
- If we dumb down the article, it will become less useful to people who have some mathematical knowledge. I bet a lot of people visiting this article are dealing with the identity at work or school. These people can use a high-level conceptual explanation. Foobaz·o< 17:33, 28 April 2008 (UTC)
RfC: Diagram ("velocity i") helpful or confusing?
Is the diagram ("traveling at velocity i multiplied by one's position") at the beginning of the article confusing or helpful? (This continues the discussion of the previous section; the previous section also includes a version of the diagram. I opened a new section following the recommendation at WP:RFC.)
Aleph4 (talk) 20:12, 28 April 2008 (UTC)
- I'm sure you can come up with a much better explanation for that image that doesn't involve imaginary velocities. I doubt anyone who didn't already know what this page is about would actually glean anything from it as it stands. Someguy1221 (talk) 23:11, 28 April 2008 (UTC)
- I agree, there are better captions that would convey just as much or more information in a way that everyone can understand (even those who aren't comfortable translating complex numbers to vectors and vectors to velocities). I propose something like, "As θ increases from 0 through the positive real numbers, the complex number eiθ moves counterclockwise around the unit circle in the complex plane, starting at the right side of the circle, ei0=e0=1. At θ=π, eiθ has reached the left side of the circle, eiπ=-1." Eh? I guess this caption doesn't make good use of the arrow from -1 to 0 in the diagram, but in a pinch the diagram could be edited... :-) --Steve (talk) 00:04, 29 April 2008 (UTC)
- That's a good explanation, and it avoids trigonometry, which is even better. I just feel like such a caption doesn't analyze the identity; it doesn't provide a step-by-step translation into English. Shouldn't this be a higher priority in the intro, to answer the what before the why, for those who have no idea what the identity is even saying? Melchoir (talk) 01:22, 29 April 2008 (UTC)
- Hmm, I'm not 100% sure what you're getting at. I guess I'm just thinking that the original caption was too ambitious in that it essentially tries to summarize in a sentence a proof/derivation, which would really take a paragraph or two to make clear. Btw, I certainly think that the box is getting at a particularly nice, "geometrical" proof of Euler's formula, and this proof would be a very nice inclusion in the article Euler's formula, to complement the other, less-visual, proofs already in that article. Also, the caption I wrote above should have another sentence at the end: "See Euler's formula.". Certainly, someone who doesn't know what the heck a complex exponential means, will be equally mystified after reading the caption. But I don't think a picture caption is the right forum to start explaining this. Maybe in the text of the introduction, it could say something like "For information on the definition and interpretation of a imaginary-number exponential, see the article: Euler's formula." The picture-caption can have the less-ambitious goal of saying "complex exponentials have this funny-looking behavior in the complex plane, and this formula is one example of that"...and then readers who want to know why complex numbers have that funny-looking behavior can click the link to Euler's formula. This article already explicitly takes the point of view (in the "Derivation" section) that the "explanation" of Euler's identity is that it's a special case of Euler's formula (and if you want to know why that holds, read the article on Euler's formula). If even the "derivation" section doesn't attempt to derive or explain Euler's formula, I think it's too much to expect of a picture caption. :-) --Steve (talk) 16:05, 30 April 2008 (UTC)
- Actually, it sounds like you do know what I'm getting at. :-) I would just make a stronger distinction between a proof/derivation and an interpretation/translation. The current caption is the latter. It certainly isn't a proof, nor does it really suggest a method of proof. Perhaps an interpretation is too ambitious for the caption; that seems to be the question.
- And yes, the relationship with the general Euler's formula is one source of the trouble. I can't think of a proof of the special case that doesn't pass through a proof of the general case. But if Wikipedia is going to have two articles, it seems wasteful for one article to refer the reader back to the other in so many places. I'm actually not too happy about the non-explanation in the "Derivation" section. Perhaps -- dare I say the M word -- a merge would help? Melchoir (talk) 21:08, 30 April 2008 (UTC)
- I posted a potential replacement below. As for the merge idea, I've always thought it was a really great thing to have many referrals to different pages, in lieu of redundancy or merging. To me, the ease of hyperlinking (and "main article:" links in particular) is at the heart of how Wikipedia can work well. Maybe it's an aesthetic difference though. :-) --Steve (talk) 17:58, 5 May 2008 (UTC)
- Sure, and often I'm the one who advocates separate articles for special cases, with the right links and so forth. For example, 1 − 2 + 4 − 8 + · · · is independent of Divergent geometric series. But I seem to recall, back when I first became involved with this article, that I looked for references, and I couldn't find any that treat the "identity" independently of the "formula". The only unique material in the current article is "Perceptions" and "Generalization". These aren't nothing, but if nothing else is forthcoming, are they enough? Melchoir (talk) 18:26, 5 May 2008 (UTC)
Proposed replacement diagram

Better? Worse? Are there improvements that could be made to the image? To the caption? This is my first Wiki image, so I may have messed up some technical aspects :-) --Steve (talk) 17:14, 5 May 2008 (UTC)
- It's a promising idea; I think there's a potential for beauty that isn't quite met. Have you considered not animating it? Melchoir (talk) 18:31, 5 May 2008 (UTC)
- Cool image. Foobaz·o< 21:52, 5 May 2008 (UTC)
- I like it - it does explain/show something and one can actually learn from it. Stotr (talk) 15:48, 6 May 2008 (UTC)
- OK, I've tentatively replaced it. Melchoir, I can't think of how to make it more beautiful and/or clear, but then again, my background is science, not graphic design. If you have more specific advice, I'd be happy to try it that way. Or, I can post the Mathematica code (it's just a couple lines) and you can tinker yourself, if you'd prefer. :-P --Steve (talk) 16:03, 6 May 2008 (UTC)
- Sure, I'll give it a shot some time. I'm thinking the animation can be avoided by showing many iterations at once, and a still image could be an svg, which looks smoother. The axes could be less busy, the "N =" could be better incorporated spatially, and color could be exploited a little more. Melchoir (talk) 16:34, 6 May 2008 (UTC)
- Wow, those are all really good ideas. I don't know how to do many of them in Mathematica though. (You're welcome to use a different program, or maybe you're better at Mathematica then me.) In case it helps, here's the Mathematica program I used: [1]. I'll leave it posted there for the foreseeable future. You (or anyone else) are welcome to try it later, or never. Good luck! :-) --Steve (talk) 17:20, 6 May 2008 (UTC)
- Thanks! I haven't ever played with Mathematica, no. The SVGs I've created were all cooked up in Adobe Illustrator, although any decent vector editor would do. For the diagrams with any nontrivial geometry, such as yours here, I usually write a C program to output Postscript, which is then imported into Illustrator for finishing touches. This workflow is probably heresy to people who actually understand Postscript, and it's not terribly efficient, but it works! Melchoir (talk) 10:19, 10 May 2008 (UTC)
- I don't know the animation, but trying it for a full 2 pi turn is kinda beautiful. Drf5n (talk) 21:59, 8 August 2011 (UTC) In R:
eulerlimplot<-function(n,z){N<-seq(0,n);plot((1+z/n)^N,type='l')}
z<-pi*2i;
eulerlimplot(1,z)
eulerlimplot(2,z)
eulerlimplot(5,z)
eulerlimplot(10,z)
eulerlimplot(20,z)
eulerlimplot(50,z)
eulerlimplot(100,z)
eulerlimplot(200,z)
eulerlimplot(500,z)
eulerlimplot(1000,z)
eulerlimplot(2000,z)
eulerlimplot(5000,z)
...
Section on Taylor Series Expansion
Is this section really worth having? TheSeven (talk) 09:10, 21 June 2008 (UTC)
- Yes, of course. The expansion is used to derive the identity. --AB (talk) 22:28, 29 June 2008 (UTC)
- Not really; the expansion is used to derive Euler's formula, and Euler's formula is then used to derive the identity (as shown in the article). My preference is to delete the section. TheSeven (talk) 14:25, 13 July 2008 (UTC)
- Yes, all right. --AB (talk) 15:41, 13 July 2008 (UTC)
- Okay! No one else commented; so I have deleted the section. TheSeven (talk) 22:00, 24 July 2008 (UTC)
- Yes, all right. --AB (talk) 15:41, 13 July 2008 (UTC)
Section on Nature of the identity
The phrase 'Three basic arithmetic operations occur exactly once each: addition, multiplication, and exponentiation' seems to leave out the square-root operation performed upon the constant negative 1: EricHerman (talk) 11:32, 13 September 2008 (UTC)
- True, but the whole idea of cataloguing which constants and operations are implicit in a given equation is imprecise to begin with. I don't think there's any mathematical value there, although someone familiar with algebraic geometry is probably going to come in here and prove me wrong.
- Anyway, for this article's purposes, it's all a matter of aesthetics. I think the current text is fairly representative of the usual treatment in popular mathematics, although I don't have a source for that. If you find a source that does point out how the square root appears in the identity, please feel free to add it in! Melchoir (talk) 04:02, 14 September 2008 (UTC)
- realistically, if we're going to replace i with we might as well replace e with . But then we'd have something crazy like:
- And noone wants that :). Chris M. (talk) 09:38, 15 December 2008 (UTC)
- Indeed, there's a glaring flaw. Allow me...
- Much better. ;) Melchoir (talk) 10:11, 15 December 2008 (UTC)
- Indeed, there's a glaring flaw. Allow me...
- LOL ... that's very cute. Actually, you could have done even better. Ramanujan came up with some infinite series for that are even longer and more astonishingly twisted and complex than the one you used, which is the most famous one (which is my favorite).Worldrimroamer (talk) 18:52, 5 December 2009 (UTC)
- Doesn't that improve a little more? Lanthanum-138 (talk) 03:52, 9 June 2011 (UTC)
Illustration of Euler's Identity
Sbyrnes321's animated graphic at the top of the page shows (1 + i*pi / n ) ^ n converging to -1 as n grows large. However, the graphic moves too quickly to read the actual real and imaginary values at each iteration. The attached pdf: <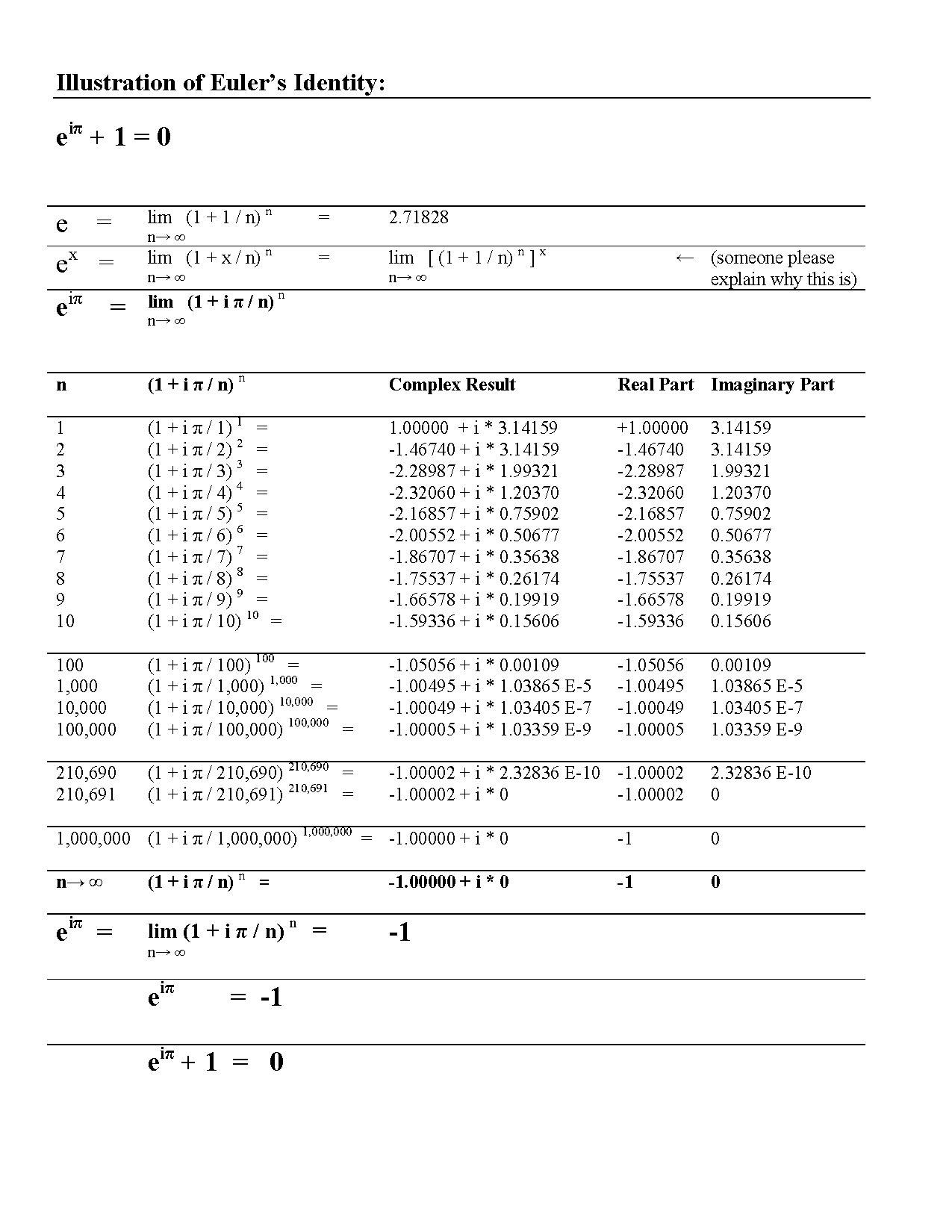 > shows the numeric value of the Complex Result for several increasing values of n. As n approaches infinity, you can see that the Real Part approaches -1 while the Imaginary Part approches 0, illustrating that e^i*pi = -1. DAnderson (talk) 06:01, 22 September 2008 (UTC)
> shows the numeric value of the Complex Result for several increasing values of n. As n approaches infinity, you can see that the Real Part approaches -1 while the Imaginary Part approches 0, illustrating that e^i*pi = -1. DAnderson (talk) 06:01, 22 September 2008 (UTC)
-1 + 1 = 0 ?
I tried to see what this formula would do and got something like this:
eπi+1=0
eπi+e0=0
eπi=-e0
πi=0
π angle 90°=0
0=0
But I wonder if eπi at the top should have been written as -eπi
because e0=0 but not e0=-1 ("or" for that matter eπi=e0
but if we could say -eπi=-1
then this statement would be legal -eπi=-e0
in other words we could start with saying this: -1 + 1 = 0
I am probably doing something terrible wrong here, but I think this correct (but maybe not correct if you look for more dimensions than RE(real)).
Anyway I find the start of the formula a little funky, could exi ever go negative therefor I suspect that for imaginary it should have
as follow: -exi
or even perhaps (1 angle 180°)exi
extra stuff:
e0=1
i=1 angle 90°=0 (in RE)
Could be interesting to hear what you people would say about this :D —Preceding unsigned comment added by 158.39.125.196 (talk) 19:58, 16 November 2008 (UTC)
- Lots of good questions here! I don't think I can do justice to them all... I advise you to ask Wikipedia:Reference desk/Mathematics. You'll probably get a good response there. Melchoir (talk) 23:07, 17 November 2008 (UTC)
- Your transition from eπi=-e0to πi=0seems unwarranted to me. With complex numbers, exp(z) is not a bijection, and exp(z1) = exp(z2) does not imply z1=z2. --Thomas Arelatensis (talk) 15:18, 10 June 2009 (UTC)
You are making it too difficult. Here is how it works. general formula is that eyi = cos(y)+i*sin(y)
If y=π, eπi = cos(π)+i*sin(π)
cos(π)=-1, and sin(π)=0, so eπi = -1+i*0 = -1 174.18.154.104 (talk) 23:44, 16 February 2010 (UTC)
More history, please
Could we please have some more on the history of this equation? Article currently says:
"the formula was likely known before Euler.[8] ... Thus, the question of whether or not the identity should be attributed to Euler is unanswered."
I presume that we could go into more detail on this. What is the evidence that this equation "was likely" known before Euler? (The cite presumably has info on this - can we add to article?) Also, obviously many people throughout history have found this equation very interesting, and we could amplify on that.
I will not be working in this article myself. Thanks for your attention. --201.53.7.16 (talk) 15:26, 26 December 2008 (UTC)
- Sandifer's article alludes to Roger Cotes's prior knowledge of Euler's identity. Cotes published a statement of the more general Euler's formula in 1714, when Euler was about seven. Euler did mention the identity eiπ + 1 = 0, in the form
- in a 1729 letter to Christian Goldbach. Sandifer comments that "it's hard to believe [Euler] figured it out for himself" and suggests that he learned the identity from Johann Bernoulli. Michael Slone (talk) 19:19, 27 December 2008 (UTC)
Is this an identity, equation, formula, or something else?
The article title refers to as an identity. Is that correct? According to the Wikipedia article on identity (mathematics), an identity is an "equality that remains true regardless of the values of any variables that appear within it". And according to the Shorter Oxford English Dictionary [2007], an identity is an "equation which holds for all values of its variables". Those two definitions are effectively the same. And since has no variables, it would seem to be stretching things to call it an identity.
Nahin's book is entitled "Dr. Euler's Fabulous Formula". I.e. Nahin refers to as a formula. Here is a quote from p.3.
… is actually not an equation. An equation (in a single variable) is a mathematical expression of the form , for example, , which is true only for certain values of the variable, that is, for the solutions of the equation. For the just cited quadratic equation, for example, equals zero for the two values of and , only. There is no x, however, to solve for in . So, it isn't an equation. It isn't an identity either, like Euler's identity , where is any angle, not just radians. That's what an identity (in a single variable) is, of course, a statement that is identitically true for any value of the variable. There isn't any variable at all, anywhere, in : just five constants. … So, isn't an equation and it isn't an identity. Well, then, what is it? It is a formula or a theorem.
I think that Nahin is correct in saying that is not an identity, but incorrect in saying that it is not an equation. Certainly, fits the Wikipedia definition of equation (mathematics). It also fits the defintion in the Shorter OED: "a mathematical formula affirming the equivalence of two symbolic or numerical expressions (indicated by the sign =)".
Could Wikipedia call it a formula, as Nahin does? There already is an Euler's formula; in fact, there are many (List_of_topics_named_after_Leonhard_Euler#Euler.E2.80.94formulas). Additionally, I think of a formula as connoting a description of something in terms of other things; for example, describes the volume of a sphere in terms of the radius: this is consistent with the discussion in the article on formula.
So, I suggest that the present article be renamed "Euler's equation". Indeed, I had always called it an equation, before seeing it called an identity in WP. I know of no other source that refers to it as an identity. And some of the references refer to it as an equation, including Keith Devlin.
TheSeven (talk) 12:26, 14 August 2009 (UTC)
- It's an identity, an equation, and a formula. Nahin's claim about what is an equation is definitely bogus. As far as I'm aware, "Euler's formula", "Euler's identity" or "Euler's equation" have long been used to refer to either of what Wikipedia calls Euler's formula and Euler's identity; exclusively calling the former "Euler's formula" and the latter "Euler's identity" is a convention that originates on Wikipedia. Fredrik Johansson 15:09, 14 August 2009 (UTC)
Quaternions
Which identity is considered more mathematically beautiful?
- (this one doesn't even work, does it?)
Leethacker1347 (talk) 17:09, 9 December 2009 (UTC)
This is interesting, as extending Euler's Identity to dimensions higher than the complex plane. (None of it is very beautiful to me, though.) I suppose it's possible to extend it to all (reasonable) dimensions, or is there a proof to the contrary? 24.27.25.87 (talk) 20:17, 25 December 2010 (UTC) Eric
What happens if you use τ = 2π as the circle constant instead? It relates circumference to radius instead of diameter. The special case of Euler's equation where x = τ produces the identity:
Is this easier to extend to quaternions? — Preceding unsigned comment added by 71.167.69.162 (talk) 16:29, 26 May 2011 (UTC)
Why Phi?
Why is phi used in e^iphi = cos(phi) + isin(phi)? When I first read this, I though it meant the golden ratio (1.618...). Why not just use x, instead of a Greek letter? Wouldn't that just be easier, and simpler to understand? Or is there some significance to phi? My 2 Cents' Worth (talk) 15:57, 19 May 2010 (UTC)
- In some contexts it's the usual convention to write angles using Greek letters, and phi is among the popular choices. It has no special significance.—Emil J. 16:52, 19 May 2010 (UTC)
Yeah, but isn't theta generally the preferred Greek variable for angles?My 2 Cents' Worth (talk) 18:51, 19 May 2010 (UTC)
- No. Actually I'm rather surprised our article on polar coordinate systems uses theta and not phi - the article on cylindrical coordinates uses phi. Maybe the preference for either is regional. Huon (talk) 20:27, 19 May 2010 (UTC)
- It is still true for , isn't it? It's just a preference, nothing to worry about. Lanthanum-138 (talk) 03:56, 9 June 2011 (UTC)
Made an appearance in a crop circle?
Of note - a crop circle appearing 22 May 2010 appears to contain an ascii coded reference to Euler's Identity - see http://img64.imageshack.us/img64/3570/img3736o.jpg
Starting radially from center along line marked with arrow, each anticlockwise "branch" is a 0 and each clockwise "branch" is a 1
Read in order they become 01100101 01011110 00101000 01101000 01101001 00101001 01110000 01101001 00101001 00110001 00111101 00110000
and converted in ASCII e^(hi)pi)1=0
Note: this makes no suppositions or assumptions about the origins of the circles themselves and is not intended to be a discussion about who's making them - only the factual statement that Euler's Identity appears to have been encoded in one of them. —Preceding unsigned comment added by 68.98.122.107 (talk) 02:52, 25 May 2010 (UTC)
Dashed clever of those aliens to know the ASCII code! 86.177.19.206 (talk) 21:51, 23 September 2010 (UTC)
Order of the topics in the article
The current order of topics is as follows: Intro, 1 Mathematical beauty, 2 Explanation, 3 Generalizations, 4 Attribution, other... I suggest a new order of these topics to make it similar to other mathematics articles, i.e: Intro, 1 Explanation, 2 Generalizations, 3 Attribution, 4 Mathematical beauty, other... This is still a mathematics article and as such, it must be prioritized for comprehension of the meaning and not the popular culture behind it. Hamsterlopithecus (talk) 15:06, 25 September 2012 (UTC)
Euler's identity expressed with Tau
I think a section about Tau could be included. Here is a very cool thread, explaining why: http://spikedmath.com/forum/viewtopic.php?f=30&t=375&sid=b7b3c2c3800ba9a38c5b62a8f583b5d5
I find especially this image very elegant, showing the "exponentiation as rotation in the complex plane": http://i1123.photobucket.com/albums/l558/Kodegadulo/Euler/Roots4.jpg
Bonus points for the second post: http://spikedmath.com/cgi-bin/mimetex.cgi?0\,%20=\,%201\,%20+\,%20e^{-i\cdot{\frac\tau{2}}} All four basic arithmetic operations appear exactly once, and in their standard order (+,−,*,/) 0,1,2 appear in numerical order e,i,tau appear in alphabetical order
I think both are very cool arguments for mentioning the Tau-version. — Preceding unsigned comment added by RicardAnufriev (talk • contribs) 01:33, 26 July 2013 (UTC)
Multiple identities?
On consulting Euler identity at the Encyclopedia of Mathematics I was surprised to find an entry which, to my untutored eyes at least, seems to correspond to the Euler product formula... Which says "The connection between the zeta function and prime numbers was discovered by Euler, who proved the identity"... In turn linking to a page which doesn't explicitly mention the term "identity". Maybe some disambiguation is needed?
Please forgive my ignorance (and my inability to grasp complex explanations!)
81.147.165.192 (talk) 20:09, 22 January 2014 (UTC)
- William Dunham's Euler: The Master of Us All also uses the expression "Euler's identity" in different ways. Imo, we need to disambiguate with Euler's formula, Euler's product]], etc. 81.147.165.192 (talk) 19:28, 23 January 2014 (UTC)
- This is why we have the page List of topics named after Leonhard Euler, helpfully linked at the top of the article. --JBL (talk) 15:05, 18 February 2014 (UTC)
- I think that that dab link is less than ideal: List of things named after Leonhard Euler#Euler's identities doesn't actually mention either [Euler's formula]] [[2] or Euler product formula. [3] (of course they are listed elsewhere on the page, but without any mention of "identity"). 86.173.146.3 (talk) 21:44, 18 February 2014 (UTC)
- This is why we have the page List of topics named after Leonhard Euler, helpfully linked at the top of the article. --JBL (talk) 15:05, 18 February 2014 (UTC)
Identity crisis?
According to Nahin, the subject of this page is not, technically speaking, an identity (nor an "equation"), but a formula or a theorem [4]. OK, so I realize I'm an annoying general reader (and that the editorial issue may not be an entirely easy one to address), but as a layperson I do tend to feel a bit let down by the terminological inconsistencies here. Especially in an article on such a key... erm, topic. 109.158.185.136 (talk) 20:32, 5 February 2014 (UTC)
- In the relevant passage, Nahin is just wrong about usage of mathematical terminology in both the formal and informal settings. Also, the idea that this point (as opposed to something substantive) has any been a confusion for any significant number of lay readers is incredible. --JBL (talk) 14:59, 18 February 2014 (UTC)
- Also, Nahin's book (while possibly interesting) should probably not be used as a reliable source for mathematical statements. --JBL (talk) 15:02, 18 February 2014 (UTC)
- Nahin is just wrong about usage of mathematical terminology in both the formal and informal settings. Please provide a relaible source to support your statement. The present wording seems (to my lay eyes at least) to conflict with Identity (mathematics).
- Also, the idea that this point (as opposed to something substantive) has any been a confusion for any significant number of lay readers is incredible. Why "incredible"? (It has certainly been a source of confusion to this lay reader, and after seeking help both here and at WT:MATH I have spent time and effort trying to address the issue on strictly editorial grounds.)
- Also, Nahin's book (while possibly interesting) should probably not be used as a reliable source for mathematical statements. This assertion seems to conflict with the current text supported by a secondary source (as restored [5] by another editor after my copyedit to tone down possible puffery).
86.173.146.3 (talk) 21:30, 18 February 2014 (UTC)
- Every single citation that refers to the given identity as "Euler's identity" is a reliable source supporting the view that this is correct usage. But, also, the article identity (mathematics) is perfectly consistent with my position; annotated for emphasis, the definition there (which, incidentally, is also not cited) reads
- "An identity is an equality relation A = B, such that A and B contain some variables [possibly none] and give the same result when the variables are substituted by any values. In other words, A = B is an identity if A and B define the same functions [possibly constant]."
- I don't know why Nahin is trying to draw a pedantic distinction here, but his distinctions don't actually make sense and certainly don't reflect a distinction in usage among practicing mathematicians.
- On the second point, the reason is that I have had hundreds of students at various levels over the past 10 or so years, and this is simply a non-issue. Evidently, you have been confused by Nahin's nonstandard usage; that's fine, because his usage is, in fact, confusing. But no one who has not been exposed to someone making strange claims about the meaning of the words "equation" and "identity" would be in a position to be confused by the choice among synonyms. This confusion is very rare because it is not naturally occurring but needs to be introduced by someone (e.g., Nahin).
- Finally, there is a big difference between using Nahin as a reference for a claim about mathematical beauty and using Nahin for a mathematical claim. --JBL (talk) 21:53, 18 February 2014 (UTC)
- Every single citation that refers to the given identity as "Euler's identity" is a reliable source supporting the view that this is correct usage. But, also, the article identity (mathematics) is perfectly consistent with my position; annotated for emphasis, the definition there (which, incidentally, is also not cited) reads
- Ok, but if it confused this educated lay reader, then I do feel that there are editorial inconsistencies that need to be addressed. That's all I was trying to communicate above (and elsewhere). 86.173.146.3 (talk) 22:01, 18 February 2014 (UTC)
"Attribution" vs. "History"
I feel "History" would be a more helpful and appropriate section title. 86.173.146.3 (talk) 15:56, 19 February 2014 (UTC)
Explanation section
I've deleted some text. There wasn't a really explanation in it. It didn't really say why
--Ssola (talk) 02:57, 16 March 2014 (UTC)
- Copied from User talk:Ssola:
- Hi there, why'd you remove material from Euler's identity? Thanks, Newyorkadam (talk) 19:38, 27 March 2014 (UTC)Newyorkadam
- @Newyorkadam: Hey, I didn't understand the section. I don't know wether it was trying to explain or illustrate the identity. It seemed to be using the fact that the rotates to discretizise the problem and show the Euler identity, but the identity wold be trivial if we knew the rotation property. I don't understand the reference of the text to group theory. In particular, if the text was trying to say something like
- I think the first equality is not justified (and I can accept the others assuming things that are not said).
- In fact, this problem arised in the Catalan wikipedia when the text was translated. I'm not an expert so I'm not sure if I'm missing something. The text was written by an anonimous IP time ago and I think I couldn't contact he/she. I hoped to atract the atention of more editors removing the text here, so I would apreciate your opinion. Sorry for my bad English. --Ssola (talk) 23:55, 27 March 2014 (UTC)
- @Ssola: I don't really understand Euler's identity, but I'm trying to get into more advanced math so I'm scanning articles and noticed the content removal. I personally think that you should've gotten a second opinion before you removed the content, but I think some would disagree on the need for a second opinion. Either way, I'd suggest putting in the edit summary to check this section on the talk page. Have a nice day
 -Newyorkadam (talk) 00:16, 28 March 2014 (UTC)Newyorkadam
-Newyorkadam (talk) 00:16, 28 March 2014 (UTC)Newyorkadam
- @Ssola: I don't really understand Euler's identity, but I'm trying to get into more advanced math so I'm scanning articles and noticed the content removal. I personally think that you should've gotten a second opinion before you removed the content, but I think some would disagree on the need for a second opinion. Either way, I'd suggest putting in the edit summary to check this section on the talk page. Have a nice day
- @Newyorkadam: Hey, I didn't understand the section. I don't know wether it was trying to explain or illustrate the identity. It seemed to be using the fact that the rotates to discretizise the problem and show the Euler identity, but the identity wold be trivial if we knew the rotation property. I don't understand the reference of the text to group theory. In particular, if the text was trying to say something like
x is a number
- — Preceding unsigned comment added by Becker-Sievert (talk • contribs) 07:13, 14 July 2014 (UTC)

































































![{\displaystyle ({e^{i}e^{j}e^{k}})^{\pi {\sqrt[{2}]{3}}^{-1}}+1=0,\,\!}](https://wikimedia.org/enwiki/api/rest_v1/media/math/render/svg/ba5823fa730d21d1d62748865d078aef8c33c1c4)
![{\displaystyle e^{\pi (i+j+k){\sqrt[{2}]{3}}^{-1}}+1=0,\,\!}](https://wikimedia.org/enwiki/api/rest_v1/media/math/render/svg/dfcee5b06df5bb5a3ca6312206d67b7eb36e1484)
![{\displaystyle e^{{\sqrt[{2}]{3}}^{-1}(i+j+k)\pi }+1=0,\,\!}](https://wikimedia.org/enwiki/api/rest_v1/media/math/render/svg/b3c6d56d1e491b79af9a0fecf5f55397d49bc743)
![{\displaystyle ({e^{i}e^{j}e^{k}})^{\tau {\sqrt[{2}]{3}}^{-1}}=1\,\!}](https://wikimedia.org/enwiki/api/rest_v1/media/math/render/svg/5a9574a4a4c7e611b80819aa74992bee34f01376)




