Concentration

For other uses, see Concentration (disambiguation).

In chemistry, concentration is the measure of how much of a given substance there is mixed with another substance. This can apply to any sort of chemical mixture, but most frequently is used in relation to solutions, where it refers to the amount of solute dissolved in a solvent.

To concentrate a solution, one must add more solute, or reduce the amount of solvent (for instance, by selective evaporation). By contrast, to dilute a solution, one must add more solvent, or reduce the amount of solute.

Unless two substances are fully miscible there exists a concentration at which no further solute will dissolve in a solution. At this point, the solution is said to be saturated. If additional solute is added to a saturated solution, it will not dissolve. Instead, phase separation will occur, leading to either coexisting phases or a suspension. The point of saturation depends on many variables such as ambient temperature and the precise chemical nature of the solvent and solute.

Qualitative description

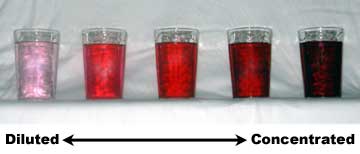
Often in informal, non-technical language, concentration is described in a qualitative way, through the use of adjectives such as "dilute" or "weak" for solutions of relatively low concentration and of others like "concentrated" or "strong" for solutions of relatively high concentration. Those terms relate the amount of a substance in a mixture to the observable intensity of effects or properties caused by that substance. For example, a practical rule is that that the more concentrated a chromatic solution is, the more intensely coloured it is.

These glasses containing red dye demonstrate qualitative changes in concentration. The solutions on the left are more dilute, compared to the more concentrated solutions on the right.

Quantitative notation

For scientifical or technical applications, a qualitative account of concentration is almost never sufficient, therefore quantitative measures are needed to describe concentration. There are a number of different ways to quantitatively express concentration; the most common are listed below. They are based on mass or volume or both. Depending on what they are based on it is not always trivial to convert one measure to the other, because knowledge of the density might be needed to do so. At times this information may not available, particularly if the temperature varies.

Mass versus volume

Some units of concentration -particularly the most popular one (molarity)- require knowledge of a substance's volume, which -in contrast to mass- is variable depending on ambient temperature and pressure. In fact (partial) molar volume can even be a function of concentration itself. This is why volumes are not necessarily completely additive when two liquids are added and mixed. Volume-based measures for concentration are therefore not to be recommended for non-dilute solutions or problems where relatively large differences in temperature are encountered (e.g. for phase diagrams).

Unless otherwise stated, all the following measurements of volume are assumed to be at a standard state temperature and pressure (for example 25 degrees Celsius at 1 atmosphere or 101.325 kPa). The measurement of mass does not require such restrictions

Mass can be determined at a precision of < 0.2 mg on a routine basis with an analytical balance and more precise instruments exist. Both solids and liquids are easily quantified by weighing.

The volume of a liquid is usually determined by calibrated glassware such as burettes and volumetric flasks. For very small volumes precision syringes are available. The use of graduated beakers and cylinders is not recommended as their indication of volume is mostly for decorative rather than quantitative purposes. The volume of solids, particularly of powders, is often difficult to measure, which is why mass is the more usual measure . For gases the opposite is true, the volume of a gas can be measured in a gas burette, if care is taken to control the pressure. The mass is not so easy to measure due to buoyancy effects.

Molarity

Molarity (M) denotes the number of moles of a given substance per litre of solution. For instance: 4.0 litres of liquid, containing 2.0 moles of dissolved particles, constitutes a solution of 0.5 M. Such a solution may be described as "0.5 molar." (Working with moles can be highly advantageous, as they enable measurement of the absolute number of particles in a solution, irrespective of their weight and volume. This is often more useful when performing stoichiometric calculations.). See molar solution for further information.

Although molarity is by far the most commonly used measure for concentration, particularly for dilute aqueous solutions, it does suffer from a number of disadvantages. Masses can be determined with great precision as balances are often very precise. Determining volume is often not as precise. In addition, a volume of a liquid changes with temperature so that the molarity also changes without adding or removing any mass. For non-dilute solutions another problem is that the molar volume of a substance is itself a function of concentration so that volume is not strictly additive.

The National Institute of Standards and Technology, the United States authority on measurement, considers the term molarity and the unit symbol M to be obsolete, and suggests instead the 'amount-of-substance concentration' (c) with units mol/m³ or other units used alongside the SI such as mol/L [1]. This recommendation has not been generally accepted in academia.

Molality

Molality (m) denotes the number of moles of a given substance per kilogram of solvent (not: solution). For instance: 2.0 kilograms of solvent, to which are added 1.0 moles of dissolved particles, constitutes a molality of 0.5 mol/kg. Such a solution may be described as "0.5 molal". The term molal solution is used as a shorthand for a "one molal solution", i.e. a solution which contains one mole of the solute per 1000 grams of the solvent.

As for water a mass of 1000 grams corresponds roughly to a volume 1 liter at room temperature, molalities and molarities converge in value for dilute aqueous solutions at ambient conditions. For different solvents and different conditions this is not the case.

NIST considers the symbol m to be obsolete, and instead suggests the notation m_B (and the use SI units such as mol/kg). This recommendation has not been generally accepted in science.

The determination of molality only requires a good balance, because the masses of both solvent and solute can be obtained by weighing. Using a balance is often more precise than working with volumetric flasks burettes and pipettes. Another advantage of molality is that it does not change with the temperature as it deals with the mass of solvent, rather than the volume of solution. Volume typically increases with increase in temperature resulting in decrease in molarity. Molality of a solution is always constant irrespective of the physical conditions like temperature and pressure.

Mole fraction

The mole fraction χ, chi (or x) (also called molar fraction) denotes the number of moles of solute as a proportion of the total number of moles in a solution. For instance: 1 mole of solute dissolved in 9 moles of solvent would have a mole fraction of 1/10 or 0.1. Mole fractions are dimensionless quantities.

This measure is used very frequently in the construction of phase diagrams. It has a number of advantages:

• the measure is not temperature dependent (such as molarity) and does not require knowledge of the densities of the phase(s) involved
• a mixture of known mole fraction can be prepared by weighing off the appropriate masses of the consituents
• the measure is symmetrical: in the mole fractions x=0.1 and x=0.9 the roles of 'solvent' and 'solute' are reversed.

As both mole fractions and molality are only based on the masses of the components it is easy to convert between these measures. This is not true for molarity, because there a knowledge of the density is required.

Mass percentage

Mass percentage denotes the mass of a substance in a mixture as a percentage of the mass of the entire mixture. For instance: if a bottle contains 40 grams of ethanol and 60 grams of water, then it contains 40% ethanol by mass. Commercial concentrated aqueous reagents such as acids and bases are often labeled in concentrations of weight percentage with the specific gravity also listed. In older texts and references this is sometimes referred to as weight-weight percentage (abbreviated as w/w). In water pollution chemistry, a common term of measuring total mass percentage of dissolved solids in an aqueous medium is total dissolved solids.

Mass-volume percentage

Mass-volume percentage, (sometimes referred to as weight-volume percentage and often abbreviated as % m/v or % w/v) denotes the mass of the substance in a mixture as a percentage of the volume of the entire mixture. Mass-volume percentage is often used for solutions made from solid reagents. It is the mass of the solute in grams divided by the volume of solution in millilitres and multiplied by one hundred.

Volume-volume percentage

Volume-volume percentage or % (v/v) describes the volume of the solute in mL per 100 mL of the resulting solution. This is most useful when a liquid - liquid solution is being prepared. For example, beer is about 5% ethanol by volume. This means every 100 mL beer contains 5 mL ethanol and 95 mL of water.

Molinity

Molinity is a rarely-used term that denotes the number of moles of a given substance per kilogram of solution. For instance: imagine 2.0 kg of solvent, plus 1.0 mol of dissolved particles, weighs a total of 2.5 kg. The molinity of the solution is therefore 1 mol / 2.5 kg = 0.4 mol/kg.

Note: molarity and molinity are calculated using the volume of the entire solution, but molality is calculated using the mass of solvent only.

Normality

This type of concentration highlights the chemical nature of salts: in solution, salts break apart into distinct reactive species (ions such as H⁺, Fe³⁺, or Ag⁺), but those distinct species can never be isolated - they only come as part of a charge-balanced salt. The concept normality, which is a measure of reactive species in a solution was developed as a way to deal with this issue.

Definition:

A normal is one gram equivalent of a solute per liter of solution. The definition of a gram equivalent varies depending on the type of chemical reaction that is discussed - it can refer to acids, bases, redox species, and ions that will precipitate.

Usage:

It is critical to note that normality measures a single ion which takes part in an overall solute. For example, one could determine the normality of hydroxide or sodium in an aqueous solution of sodium hydroxide, but the normality of sodium hydroxide itself has no meaning. Nevertheless it is often used to describe solutions of acids or bases, in those cases it is implied that the normality refers to the H+ or OH- ion. For example, 2 Normal Sulfuric Acid (H₂SO₄), means that the normality of H+ ions is 4, or that the Molarity of the Sulfuric Acid is 1.Similarly for 1 moral H₃PO₄ the normality is 3 as it contains 3 H+.

Specific Cases:

As ions in solution can react through different pathways, there are three common definitions for normality as a measure of reactive species in solution:

• In acid-base chemistry, Normality is used to express the concentration protons or hydroxide ions in the solution. Here, the normality differs from the molarity by an integer value - each solute can produce n equivalents of reactive species when dissolved. For example: 1 M aqueous Ca(OH)₂ is 2 N (normal) in hydroxide.

• In redox reactions, normality measures the quantity of oxidizing or reducing agent that can accept or furnish one mole of electrons. Here, the normality scales from the molarity, most commonly, by a fractional value. Calculating the normality of redox species in solution can be challenging.

• In precipitation reactions, normality measures the concentration of ions which will precipitate in a given reaction. Here, the normality scales from the molarity again by an integer value.

Note: The same ion at the same concentration can possess a different normality, depending on which kind of reaction it participates in

Practical Uses:

The measure of normality is extremely useful for titrations - given two species that are known to react with a known ratio, one simply needs to scale the volumes of solutions with known normalities to get a complete reaction with the following equation:

N_aV_a=N_bV_b

Because this concentration measure depends on the very chemistry of the reaction the solute participates in, its use has been largely abandoned. NIST has also stipulated that this unit is obsolete and recommends discontinuing its use. This recommendation is likely to find widespread acceptance in academia.

Formal

The formal (F) is yet another measure of concentration similar to molarity. It is rarely used. It is calculated based on the formula weights of chemicals per litre of solution. The difference between formal and molar concentrations is that the formal concentration indicates moles of the original chemical formula in solution, without regard for the species that actually exist in solution. Molar concentration, on the other hand, is the concentration of species in solution.

For example: if one dissolves sodium carbonate (Na₂CO₃) in a litre of water, the compound dissociates into the Na⁺ and CO₃^2- ions. Some of the CO₃^2- reacts with the water to form HCO₃^- and H₂CO₃. If the pH of the solution is low, there is practically no Na₂CO₃ left in the solution. So, although we have added 1 mol of Na₂CO₃ to the solution, it does not contain 1 M of that substance. (Rather, it contains a molarity based on the other constituents of the solution.) However, one can still say that the solution contains 1 F of Na₂CO₃.

"Parts-per" notation

The parts-per notation is used in some areas of science and engineering because it does not require conversion from weights or volumes to more chemically relevant units such as normality or molarity. It describes the amount of one substance in another. It is the ratio of the amount of the substance of interest to the amount of that substance plus the amount of the substance it is in. e.g. 10 parts per million (ppm) sugar in water means that there are 10 mg of sugar in 999,990 mg of water.

• Parts per hundred (denoted by '%' and very rarely 'pph') - denotes the amount of a given substance in a total amount of 100 regardless of the units of measure as long as they are the same. e.g. 1 gm in a total weight of 100 gm. This is the common percent. 1 part in 10².

• Parts per thousand (denoted by '‰‰' [the per mil symbol], and occasionally 'ppt') denotes denotes the amount of a given substance in a total amount of 1000 regardless of the units of measure as long as they are the same. e.g. 1 liter in a total volume of 1000 liters. 1 part in 10³.

• Parts per million ('ppm') denotes denotes the amount of a given substance in a total amount of 1,000,000 regardless of the units of measure used as long as they are the same. e.g. 1 mg in a total weight of 1,000,000 mg. 1 part in 10⁶.

• Parts per billion ('ppb') denotes denotes the amount of a given substance in a total amount of 1,000,000,000 regardless of the units of measure as long as they are the same. e.g. 1 gallon in a total volume of 1 billion gallons. 1 part in 10⁹.

• Parts per trillion ('ppt') denotes denotes the amount of a given substance in a total amount of 1,000,000,000,000 regardless of the units of measure as long as they are the same. e.g. 1 pound in a total weight of 1 trillion pounds. 1 part in 10¹².

• Parts per quadrillion ('ppq') denotes denotes the amount of a given substance in a total amount of 1,000,000,000,000,000 regardless of the units of measure as long as they are the same. There are currently no analytical techniques that can measure ppq concentrations. 1 part in 10¹⁵.

Warning: although 'ppt' is usually used to denote 'parts per trillion', it is also on occasion used to denote 'parts per thousand'. If there is any chance of ambiguity, one should describe the abbreviation in full.

According to the U.S. National Institute of Standards and Technology (NIST) Guide for the Use of the International System of Units (SI), "the language-dependent terms part per million, part per billion, and part per trillion ... are not acceptable for use with the SI to express the values of quantities." [2] which lists examples of alternative expressions.

Notes for clarity: The notation is used for convenience and the units of measure must be denoted for clarity though this is frequently not the case even in technical publications.

In atmospheric chemistry and in air pollution regulations, the parts per notation is commonly expressed with a v following, such as ppmv, to indicate parts per million by volume. This works fine for gas concentrations (e.g., ppmv of carbon dioxide in the ambient air) but, for concentrations of non-gaseous substances such as aerosols, cloud droplets, and particulate matter in the ambient air, the concentrations are commonly expressed as μg/m³ or mg/m³ (e.g., μg or mg of particulates per cubic metre of ambient air). This expression eliminates the need to take into account the impact of temperature and pressure on the density and hence weight of the gas.

The usage is generally quite fixed inside most specific branches of science, leading some researchers to believe that their own usage (mass/mass, volume/volume or others) is the only correct one. This, in turn, leads them not to specify their usage in their research, and others may therefore misinterpret their results. For example, electrochemists often use volume/volume, while chemical engineers may use mass/mass as well as volume/volume. Many academic papers of otherwise excellent level fail to specify their usage of the part-per notation. The difference between expressing concentrations as mass/mass or volume/volume is quite significant when dealing with gases and it is very important to specify which is being used. It is quite simple, for example, to distinguish ppm by volume from ppm by mass or weight by using ppmv or ppmw.

Techniques used to determine concentration

• Preparation of known mixtures
  • Mass - Weighing (balances)
  • Volume of liquids (gases) - calibrated glassware, syringes
• Measurement of unknown mixtures
  • Spectroscopic methods
    • Spectrophotometry (UV/VIS)
    • Atomic Absorption Spectroscopy (AAS)
    • X-ray fluorescence
    • Neutron activation
  • Chromatography
  • Various titration methods
  • Electrochemical methods

Table of concentration measures

Frequently used standards of concentration

Measurement	Notation	Generic formula	Typical units
Mass percentage	-	$\left({\frac {\mathrm {grams} \ \mathrm {solute} \times 100}{\mathrm {grams} \ \mathrm {solution} }}\right)$	%
Mass-volume percentage	-	$\left({\frac {\mathrm {grams} \ \mathrm {solute} \times 100}{\mathrm {millilitres} \ \mathrm {solution} }}\right)$	% though strictly %kg/L
Volume-volume percentage	-	$\left({\frac {\mathrm {millilitres} \ \mathrm {solute} \times 100}{\mathrm {millilitres} \ \mathrm {solution} }}\right)$	%
Molarity	M	$\left({\frac {\mathrm {moles} \ \mathrm {solute} }{\mathrm {litres} \ \mathrm {solution} }}\right)$	mol/L (or M)
Molinity	-	$\left({\frac {\mathrm {moles} \ \mathrm {solute} }{\mathrm {kilograms} \ \mathrm {solution} }}\right)$	mol/kg
Molality	m	$\left({\frac {moles\ solute}{kilograms\ solvent}}\right)$	mol/kg (or m)
Molar fraction	χ (chi)	$\left({\frac {moles\ solute}{moles\ solution}}\right)$	(fraction)
Formal	F	$\left({\frac {moles\ undissolved\ solute}{litres\ solution}}\right)$	mol/L (or F)
Normality	N	$\left({\frac {gram\ equivalents}{litres\ solution}}\right)$	N
Parts per hundred	% (or pph)	$\left({\frac {dekagrams\ solute}{kilograms\ solution}}\right)$	da.g/kg
Parts per thousand	‰‰ (or ppt*)	$\left({\frac {grams\ solute}{kilograms\ solution}}\right)$	g/kg
Parts per million	ppm	$\left({\frac {milligrams\ solute}{kilograms\ solution}}\right)$	mg/kg
Parts per billion	ppb	$\left({\frac {micrograms\ solute}{kilograms\ solution}}\right)$	μg/kg
Parts per trillion	ppt*	$\left({\frac {nanograms\ solute}{kilograms\ solution}}\right)$	ng/kg
Parts per quadrillion	ppq	$\left({\frac {picograms\ solute}{kilograms\ solution}}\right)$	pg/kg

* Although 'ppt' is usually used to denote 'parts per trillion', it is on occasion used for 'parts per thousand'. Sometimes 'ppt' is also used as an abbreviation for precipitate.

Note (1) : The table above is described in terms of solvents and solutes; however the units given often also apply to other types of mixture.

Note (2) : The use of billion, trillion, quadrillion above follows the short scale usage of these words.