Lurie–Houghton telescope: Difference between revisions
citations formatted as templates; math formatting |
copy edit |
||
| Line 1: | Line 1: | ||
[[Image:lh scope front closeup 600x430.jpg|right|thumb|250px|A Lurie–Houghton telescope]] |
[[Image:lh scope front closeup 600x430.jpg|right|thumb|250px|A Lurie–Houghton telescope]] |
||
The '''Lurie–Houghton telescope''' is a [[catadioptric]] [[telescope]]. |
The '''Houghton telescope''' or '''Lurie–Houghton telescope''' is a [[catadioptric]] [[telescope]]. Houghton's original design was patented<ref>{{cite patent |inventor=Houghton, James Leonard |assign1=Eastman Kodak Co., Rochester, NY |country=US |number=2350112 |gdate=30 May 1944 |fdate=2 March 1942}}</ref> in 1944. Instead of the fairly hard to make [[Schmidt corrector plate|Schimdt]] and heavy [[meniscus corrector|heavy meniscus (Maksutov)]] corrector [[lens (optics)|lenses]], the corrector for the Houghton is relatively easy to make. It consists of two lenses: a positive and a negative, set at the front of the telescope which fixes the telescope's aperture. All lens and mirror surfaces are [[spherical mirror|spheroidal]], which eases construction. These lenses are relatively thin, though not as thin as the [[Schmidt corrector plate|Schmidt]] corrector. With a good [[anti-reflective coating]], light loss and "ghost" reflections are minimal. |
||
Lurie slightly modified Houghton's original design by adding a diagonal mirror to direct the focused light outside the telescope tube in the same way as a [[Newtonian telescope]].<ref>{{cite book |last1=Rutten |first1=Harrie |last2=van Venrooij |first2=Martin |year=1988 |title=Telescope Optics - Evaluation and Design |page=127 |publisher=Willmann-Bell, Inc. |location=Richmond, VA |ISBN=0-943396-18-2}}</ref> |
|||
== The corrector == |
== The corrector == |
||
Each surface of the lenses in the corrector creates a degree of freedom to correct optical aberrations. There are enough degrees of freedom to correct for [[spherical aberration]], [[Coma (optics)|coma]], and field curvature |
Each surface of the lenses in the corrector<ref group=lower-alpha>The total power of a "corrector lens" is 1, by definition.</ref> creates a degree of freedom to correct optical aberrations. There are enough degrees of freedom to correct for [[spherical aberration]], [[Coma (optics)|coma]], and field curvature. It is placed in the path of the incoming light rays, which are parallel, so the residual [[chromatic aberration]] is very nearly zero. The Houghton corrector can be made of the same type of glass (usually BK7) which reduces cost. The design tolerances are very modest compared to the similar [[Maksutov telescope]]s. |
||
There are two types of correctors |
There are two types of correctors: symmetric and asymmetric. In the symmetric version ''R''<sub>1</sub> = −''R''<sub>3</sub> and ''R''<sub>2</sub> = −''R''<sub>4</sub> which allows for [[interferometry]] testing of the surfaces against each other. However, the correction is slightly worse than the asymmetric corrector with four different radii. The following figure presents the equations necessary to design the corrector.<ref group=lower-alpha>Note that in these equations ''D'' is not the diameter of the primary mirror; rather, it is the distance from the mirror to the corrector as a fraction of the focal length of the mirror.</ref> |
||
[[Image:lh design equations s.gif|center|Houghton Doublet Corrector Design Equations]] |
[[Image:lh design equations s.gif|center|Houghton Doublet Corrector Design Equations]] |
||
== The mirrors == |
== The mirrors == |
||
The |
The Houghton uses a spheroidal primary mirror unlike the paraboidal mirror used in the Newtonian telescope. A spheroidal mirror is much easier to make because the entire surface appears to evenly black out when tested with a Foucault tester. In the Houghton and the Lurie–Houghton, the radius of curvature of the primary mirror is slightly less than that of the total system. The diameter of the primary mirror should be larger than the aperture set by the corrector, to avoid vignetting. |
||
The secondary mirror in the Lurie–Houghton is |
The secondary mirror in the Lurie–Houghton is identical to the secondary mirror in a Newtonian telescope. An advantage of the Lurie–Houghton over the Newtonian is that the secondary mirror can be mounted to one of the corrector lenses, thus eliminating the spider mount. This eliminates diffraction of the incoming light that is caused by the vanes of the spider mount. |
||
==Notes== |
==Notes== |
||
Revision as of 02:03, 22 June 2018

The Houghton telescope or Lurie–Houghton telescope is a catadioptric telescope. Houghton's original design was patented[1] in 1944. Instead of the fairly hard to make Schimdt and heavy heavy meniscus (Maksutov) corrector lenses, the corrector for the Houghton is relatively easy to make. It consists of two lenses: a positive and a negative, set at the front of the telescope which fixes the telescope's aperture. All lens and mirror surfaces are spheroidal, which eases construction. These lenses are relatively thin, though not as thin as the Schmidt corrector. With a good anti-reflective coating, light loss and "ghost" reflections are minimal.
Lurie slightly modified Houghton's original design by adding a diagonal mirror to direct the focused light outside the telescope tube in the same way as a Newtonian telescope.[2]
The corrector
Each surface of the lenses in the corrector[a] creates a degree of freedom to correct optical aberrations. There are enough degrees of freedom to correct for spherical aberration, coma, and field curvature. It is placed in the path of the incoming light rays, which are parallel, so the residual chromatic aberration is very nearly zero. The Houghton corrector can be made of the same type of glass (usually BK7) which reduces cost. The design tolerances are very modest compared to the similar Maksutov telescopes.
There are two types of correctors: symmetric and asymmetric. In the symmetric version R1 = −R3 and R2 = −R4 which allows for interferometry testing of the surfaces against each other. However, the correction is slightly worse than the asymmetric corrector with four different radii. The following figure presents the equations necessary to design the corrector.[b]
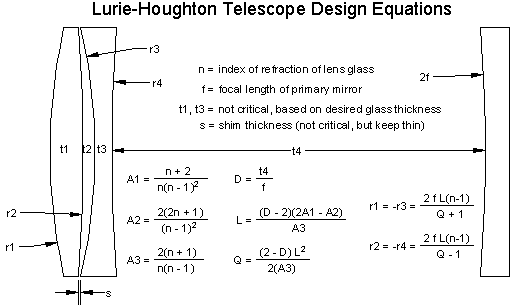
The mirrors
The Houghton uses a spheroidal primary mirror unlike the paraboidal mirror used in the Newtonian telescope. A spheroidal mirror is much easier to make because the entire surface appears to evenly black out when tested with a Foucault tester. In the Houghton and the Lurie–Houghton, the radius of curvature of the primary mirror is slightly less than that of the total system. The diameter of the primary mirror should be larger than the aperture set by the corrector, to avoid vignetting.
The secondary mirror in the Lurie–Houghton is identical to the secondary mirror in a Newtonian telescope. An advantage of the Lurie–Houghton over the Newtonian is that the secondary mirror can be mounted to one of the corrector lenses, thus eliminating the spider mount. This eliminates diffraction of the incoming light that is caused by the vanes of the spider mount.
Notes
References
- ^ US 2350112, Houghton, James Leonard, issued 30 May 1944, assigned to Eastman Kodak Co., Rochester, NY
- ^ Rutten, Harrie; van Venrooij, Martin (1988). Telescope Optics - Evaluation and Design. Richmond, VA: Willmann-Bell, Inc. p. 127. ISBN 0-943396-18-2.
