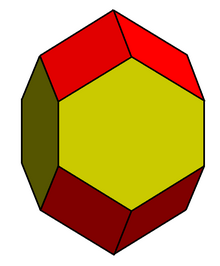
Elongated dodecahedron
| Elongated dodecahedron | |
|---|---|
 | |
| Type | Dodecahedron |
| Faces | 8 rhombi 4 hexagons |
| Edges | 28 |
| Vertices | 18 |
| Vertex configuration | (8) 4.6.6 (8) 4.4.6 (2) 4.4.4.4 |
| Symmetry group | D4h, [4,2], (*422), order 16 |
| Rotation group | D4, [4,2]+, (422), order 8 |
| Dual polyhedron | - |
| Properties | convex, parallelohedron |
| Net | |
 | |
In geometry, the elongated dodecahedron,[1] extended rhombic dodecahedron, rhombo-hexagonal dodecahedron[2] or hexarhombic dodecahedron[3] is a convex polyhedron with 8 rhombic and 4 hexagonal faces. The hexagons can be made equilateral, or regular depending on the shape of the rhombi. It can be seen as constructed from a rhombic dodecahedron elongated by a square prism. Along with the rhombic dodecahedron, it is a space-filling polyhedron.
Variations
The elongated dodecahedron can be constructed as a contraction of a uniform truncated octahedron, where square faces are reduced to single edges and regular hexagonal faces are reduced to 60 degree rhombic faces. This construction has half the symmetry, D2h symmetry, order 8.
Tessellation
- It can tesselate all space by translations.
- It is the Wigner-Seitz cell for certain body-centered tetragonal lattices.

|

|
This is related to the rhombic dodecahedral honeycomb with an elongation of zero. Projected normal to the elongation direction, the honeycomb looks like a square tiling with the rhombi projected into squares.
See also
References
- ^ Coxeter (1973) p.257
- ^ Williamson (1979) p169
- ^ http://www.matha.mathematik.uni-dortmund.de/~thilo/contents/fedorov.htm
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. rhombo-hexagonal dodecahedron, p169
- H.S.M. Coxeter, Regular Polytopes, Third edition, (1973), Dover edition, ISBN 0-486-61480-8 p. 257

