Triangle
A triangle is one of the basic shapes of geometry: a polygon with three vertices and three sides which are straight line segments.
Any three non-collinear points determine a triangle and a unique plane, i.e. two dimensional Cartesian space in Euclidean geometry .
Types of triangles
Triangles can be classified according to the relative lengths of their sides:
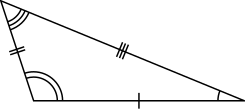
- In an equilateral triangle, all sides are of equal length. An equilateral triangle is also equiangular, i.e. all its internal angles are equal—namely, 60°; it is a regular polygon[1]
- In an isosceles triangle, at least two sides are of equal length. An isosceles triangle also has two congruent angles (namely, the angles opposite the congruent sides). An equilateral triangle is also an isosceles triangle, but not all isosceles triangles are equilateral triangles.[2]
- In a scalene triangle, all sides have different lengths. The internal angles in a scalene triangle are all different.[3]
 |
 |
 |
| Equilateral | Isosceles | Scalene |
Triangles can also be classified according to the size of their largest internal angle, described below using degrees of arc.
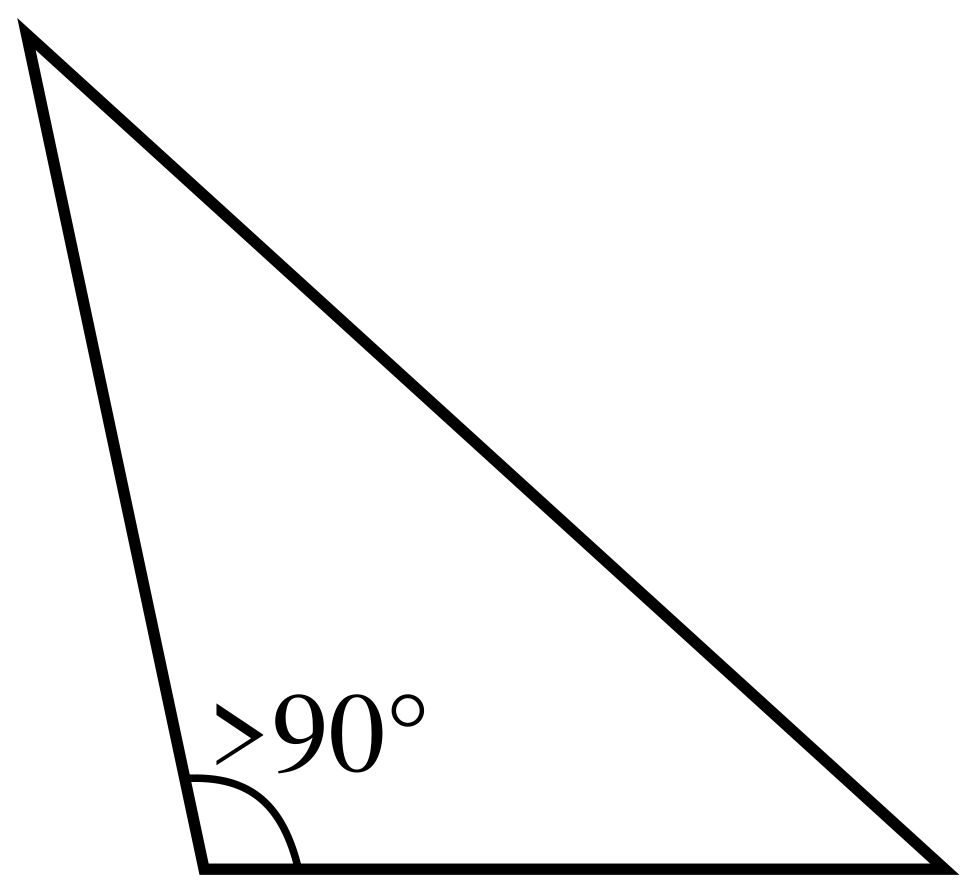
- A right triangle (or right-angled triangle, formerly called a rectangled triangle) has one 90° internal angle (a right angle). The side opposite to the right angle is the hypotenuse; it is the longest side in the right triangle. The other two sides are the legs or catheti (singular: cathetus) of the triangle.
- An obtuse triangle has one internal angle larger than 90° (an obtuse angle).
- An acute triangle has internal angles that are all smaller than 90° (three acute angles). An equilateral triangle is an acute triangle, but not all acute triangles are equilateral triangles.
 |
 |
 |
| Right | Obtuse | Acute |
Basic facts
Elementary facts about triangles were presented by Euclid in books 1-4 of his Elements around 300 BCE.
A triangle is a polygon and a 2-simplex (see polytope). All triangles are two-dimensional.
An exterior angle of a triangle (an angle that is adjacent and supplementary to an internal angle) is always equal to the two angles of a triangle that it is not adjacent/supplementary to.
Also, the exterior angles (3 total) of a triangle measure up to 360 degrees.
Two triangles are said to be similar if and only if the angles of one are equal to the corresponding angles of the other. In this case, the lengths of their corresponding sides are proportional. This occurs for example when two triangles share an angle and the sides opposite to that angle are parallel.
A few basic postulates and theorems about similar triangles: Two triangles are similar if at least 2 corresponding angles are congruent. If two corresponding sides of two triangles are in proportion, and their included angles are congruent, the triangles are similar. If three sides of two triangles are in proportion, the triangles are similar.
For two triangles to be congruent, each of their corresponding angles and sides must be congruent (6 total).
STEVEN IS A FET HOMO
A few basic postulates and theorems about congruent triangles:
SAS Postulate: If two sides and the included angles of two triangles are correspondingly congruent, the two triangles are congruent.
SSS Postulate: If every side of two triangles are correspondingly congruent, the triangles are congruent.
ASA Postulate: If two angles and the included sides of two triangles are correspondingly congruent, the two triangles are congruent.
AAS Theorem: If two angles and any side of two triangles are correspondingly congruent, the two triangles are congruent.
Hypotenuse-Leg Theorem: If the hypotenuses and 1 pair of legs of two right triangles are correspondingly congruent, the triangles are congruent.
Using right triangles and the concept of similarity, the trigonometric functions sine and cosine can be defined. These are functions of an angle which are investigated in trigonometry.
In Euclidean geometry, the sum of the internal angles of a triangle is equal to 180°. This allows determination of the third angle of any triangle as soon as two angles are known.

A central theorem is the Pythagorean theorem stating that in any right triangle, the area of the square on the hypotenuse is equal to the sum of the areas of the squares on the other two sides. If side C is the hypotenuse, we can write this as
The converse is true; If, in a triangle, a squared plus b squared equals c squared, the triangle is a right triangle.
A few basic theorems about right triangles: The acute angles of a right triangle are complementary. If the legs of a right triangle are congruent, then.... the angles opposite the legs are congruent, which are also acute and complementary, and thus those opposite angles are both 45 degrees. The triangle is then called a 45-45 Right Triangle, and so, the leg of a 45-45 Right Triangle is equal to the hypotenuse divided by half times the square root of 2. Also, the hypotenuse is the leg times the square root of 2. If the acute angles of a Right Triangle measure 30 and 60 degrees (a 30-60 right triangle), the opposite side (and the shortest side) of the 30 degree angle times two equals the hypotenuse. The side opposite the 60 degree angle equals the side opposite the 30 degree angle time the square root of 3.
Last, four definitions and two more right triangle theorems. Median: The 3 segments from each vertex of a triangle that go to the opposite side of the triangle in such a way that they bisect the opposite sides. Altitude: The 3 segments from each vertex of a triangle that go to the opposite side of the triangle in such a way that they are perpendicular to the opposite side. Note that in obtuse triangles, the altitudes drawn using the vertices of the acute angles do not hit the opposite sides, they hit the extended line of the opposite sides. Also, two altitudes of a right triangle are the legs. Therefore, a right triangle has only 1 drawable altitude.
Theorem: The median to the hypotenuse of a right triangle.... bisects it, however, the two congruent segments it cuts off are also congruent to the median itself, so the median actually creates three congruent segments.
Inscribed Triangle: A triangle that is completely inside a circle in such a way that the vertices of the triangle each are ON the circle. Circumscribed triangle: A triangle that is completely outside of a circle in such a way that each side of the triangle hits ONLY 1 point on the circle each.
Theorem: In an right triangle that is inscribed in a circle, the hypotenuse is a diameter of the circle (this theorem is VERY easily noticeable if you use the last theorem, where the median hits the center of the circle, the median is a radius, and the two segments that it congruently cuts off are also radii.
Points, lines and circles associated with a triangle
There are hundreds of different constructions that find a special point inside a triangle, satisfying some unique property: see the references section for a catalogue of them. Often they are constructed by finding three lines associated in a symmetrical way with the three sides (or vertices) and then proving that the three lines meet in a single point: an important tool for proving the existence of these is Ceva's theorem, which gives a criterion for determining when three such lines are concurrent. Similarly, lines associated with a triangle are often constructed by proving that three symmetrically constructed points are collinear: here Menelaus' theorem gives a useful general criterion. In this section just a few of the most commonly-encountered constructions are explained.

STEVEN IS A FAT HOMO A perpendicular bisector of a triangle is a straight line passing through the midpoint of a side and being perpendicular to it, i.e. forming a right angle with it. The three perpendicular bisectors meet in a single point, the triangle's circumcenter; this point is the center of the circumcircle, the circle passing through all three vertices. The diameter of this circle can be found from the law of sines stated above.
Thales' theorem states that if the circumcenter is located on one side of the triangle, then the opposite angle is a right one. More is true: if the circumcenter is located inside the triangle, then the triangle is acute; if the circumcenter is located outside the triangle, then the triangle is obtuse.

An altitude of a triangle is a straight line through a vertex and perpendicular to (i.e. forming a right angle with) the opposite side. This opposite side is called the base of the altitude, and the point where the altitude intersects the base (or its extension) is called the foot of the altitude. The length of the altitude is the distance between the base and the vertex. The three altitudes intersect in a single point, called the orthocenter of the triangle. The orthocenter lies inside the triangle if and only if the triangle is acute. The three vertices together with the orthocenter are said to form an orthocentric system.

An angle bisector of a triangle is a straight line through a vertex which cuts the corresponding angle in half. The three angle bisectors intersect in a single point, the incenter, the center of the triangle's incircle. The incircle is the circle which lies inside the triangle and touches all three sides. There are three other important circles, the excircles; they lie outside the triangle and touch one side as well as the extensions of the other two. The centers of the in- and excircles form an orthocentric system.

A median of a triangle is a straight line through a vertex and the midpoint of the opposite side, and divides the triangle into two equal areas. The three medians intersect in a single point, the triangle's centroid. This is also the triangle's center of gravity: if the triangle were made out of wood, say, you could balance it on its centroid, or on any line through the centroid. The centroid cuts every median in the ratio 2:1, i.e. the distance between a vertex and the centroid is twice as large as the distance between the centroid and the midpoint of the opposite side.

The midpoints of the three sides and the feet of the three altitudes all lie on a single circle, the triangle's nine-point circle. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the orthocenter. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the Feuerbach point) and the three excircles.

The centroid (yellow), orthocenter (blue), circumcenter (green) and barycenter of the nine-point circle (red point) all lie on a single line, known as Euler's line (red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter.
The center of the incircle is not in general located on Euler's line.
If one reflects a median at the angle bisector that passes through the same vertex, one obtains a symmedian. The three symmedians intersect in a single point, the symmedian point of the triangle.
Computing the area of a triangle
Calculating the area of a triangle is an elementary problem encountered often in many different situations. Various approaches exist, depending on what is known about the triangle. What follows is a selection of frequently used formulae for the area of a triangle.[4]
Using vectors
The area of a parallelogram can also be calculated by the use of vectors. If AB and AC are vectors pointing from A to B and from A to C, respectively, the area of parallelogram ABDC is |AB × AC|, the magnitude of the cross product of vectors AB and AC. |AB × AC| is also equal to |h × AC|, where h represents the altitude h as a vector.
The area of triangle ABC is half of this, or S = ½|AB × AC|.
The area of triangle ABC can also be expressed in term of dot products as follows:

Using trigonometry
The altitude of a triangle can be found through an application of trigonometry. Using the labelling as in the image on the left, the altitude is h = a sin γ. Substituting this in the formula S = ½bh derived above, the area of the triangle can be expressed as:
Furthermore, since sin α = sin (π - α) = sin (β + γ), and similarly for the other two angles:
Using coordinates
If vertex A is located at the origin (0, 0) of a Cartesian coordinate system and the coordinates of the other two vertices are given by B = (xB, yB) and C = (xC, yC), then the area S can be computed as ½ times the absolute value of the determinant
For three general vertices, the equation is:
In three dimensions, the area of a general triangle {A = (xA, yA, zA), B = (xB, yB, zB) and C = (xC, yC, zC)} is the 'Pythagorean' sum of the areas of the respective projections on the three principal planes (i.e. x=0, y=0 and z=0):
Using Heron's formula
The shape of the triangle is determined by the lengths of the sides alone. Therefore the area S also can be derived from the lengths of the sides. By Heron's formula:
where s = ½ (a + b + c) is the semiperimeter, or half of the triangle's perimeter.
An equivalent way of writing Heron's formula is
Non-planar triangles
A non-planar triangle is a triangle which is not contained in a (flat) plane. Examples of non-planar triangles in noneuclidean geometries are spherical triangles in spherical geometry and hyperbolic triangles in hyperbolic geometry.
While all regular, planar (two dimensional) triangles contain angles that add up to 180°, there are cases in which the angles of a triangle can be greater than or less than 180°. In curved figures, a triangle on a negatively curved figure ("saddle") will have its angles add up to less than 180° while a triangle on a positively curved figure ("sphere") will have its angles add up to more than 180°. Thus, if one were to draw a giant triangle on the surface of the Earth, one would find that the sum of its angles were greater than 180°.
See also
References
External links
- Triangle Calculator - solves for remaining sides and angles when given three sides or angles, supports degrees and radians.
- Napoleon's theorem A triangle with three equilateral triangles. A purely geometric proof. It uses the Fermat point to prove Napoleon's theorem without transformations by Antonio Gutierrez from "Geometry Step by Step from the Land of the Incas"
- William Kahan: Miscalculating Area and Angles of a Needle-like Triangle.
- Clark Kimberling: Encyclopedia of triangle centers. Lists some 3200 interesting points associated with any triangle.
- Christian Obrecht: Eukleides. Software package for creating illustrations of facts about triangles and other theorems in Euclidean geometry.
- Proof that the sum of the angles in a triangle is 180 degrees
- Triangle constructions, remarkable points and lines, and metric relations in a triangle at cut-the-knot
- Compendium Geometry Analytical Geometry of Triangles
- Area of a triangle - 7 different ways
- Triangle definition pages with interactive applets that are also useful in a classroom setting. Math Open Reference
Animated demonstrations of constructions using compass and straightedge:









