跳转到内容

擬詹森多面體：修订间差异

本页使用了标题或全文手工转换
维基百科，自由的百科全书
以互动方式浏览历史
←上一版本
删除的内容 添加的内容
可视化wikitext
无编辑摘要
 
（未显示5个用户的134个中间版本）
第1行： 第1行：
{{noteTA
{{noteTA
|1=zh:擬Johnson多面體;zh-hans:拟约翰逊多面体; zh-hant:擬詹森多面體;
|1=zh:擬Johnson多面體; zh-hans:拟约翰逊多面体; zh-hant:擬詹森多面體;
|2=zh-hans:台塔; zh-hant:帳塔;
|2=zh-hans:台塔; zh-hant:帳塔;
|3=zh-hans:丸塔; zh-hant:罩帳;
|3=zh-hans:丸塔; zh-hant:罩帳;
第6行： 第6行：
|G1=Math
|G1=Math
}}
}}
{{Geometric Shape Example
在[[幾何學]]中，'''擬詹森多面體'''是嚴格[[凸多面體]]，其面幾乎都是[[正多邊形]]，但其中有部分或全部的面不是正多邊形但很接近正多邊形。這種多面體也包含[[詹森多面體]]，即所有的面都是[[正多邊形]]，而擬詹森多面體經常會有在物理構造沒有注意到的差異在正多邊形與非正多邊形之間<ref>{{citation|contribution=Symmetrohedra: Polyhedra from Symmetric Placement of Regular Polygons|title=Bridges: Mathematical Connections in Art, Music and Science|year=2001|url=http://www.cgl.uwaterloo.ca/~csk/papers/kaplan_hart_bridges2001.pdf|first1=Craig S.|last1=Kaplan|first2=George W.|last2=Hart|author2-link=George W. Hart|accessdate=2014-05-01|archive-date=2015-09-23|archive-url=https://web.archive.org/web/20150923202125/http://www.cgl.uwaterloo.ca/~csk/papers/kaplan_hart_bridges2001.pdf|dead-url=no}}.</ref>。近似的精確值取決於這樣一個多面體的面逼近正多邊形的程度。
|shape class=擬詹森多面體
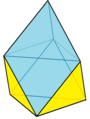
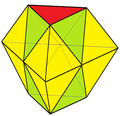
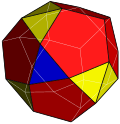
|Tetrated_dodecahedron.svg|name=[[四階十二面體]]
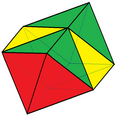
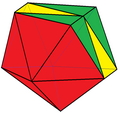
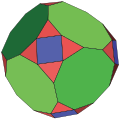
|Uncompleted rectified truncated octahedron.svg|name2=[[部分截半截角八面體]]
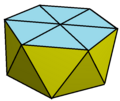
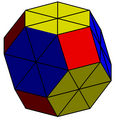
|Pyritohedral_near-miss_johnson.png|name3=[[五邊形六邊形五角十二面七十四面體|五邊形六邊形<br/>五角十二面七十四面體]]
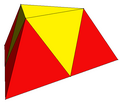
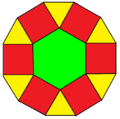
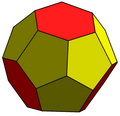
|Truncated_triakis_tetrahedron.png|name4=[[截角三角化四面體]]
}}
在[[幾何學]]中，'''擬詹森多面體'''是[[嚴格凸多面體]]，其[[面 (幾何)|面]]幾乎都是[[正多邊形]]，但其中有部分或全部的[[面 (幾何)|面]]不是正多邊形但很接近正多邊形。
而擬詹森多面體經常會在正多邊形與非正多邊形之間有物理構造上可以忽略的微小差異<ref>{{citation|contribution=Symmetrohedra: Polyhedra from Symmetric Placement of Regular Polygons|title=Bridges: Mathematical Connections in Art, Music and Science|year=2001|url=http://www.cgl.uwaterloo.ca/~csk/papers/kaplan_hart_bridges2001.pdf|first1=Craig S.|last1=Kaplan|first2=George W.|last2=Hart|author2-link=George W. Hart|accessdate=2014-05-01|archive-date=2015-09-23|archive-url=https://web.archive.org/web/20150923202125/http://www.cgl.uwaterloo.ca/~csk/papers/kaplan_hart_bridges2001.pdf|dead-url=no}}.</ref>。近似的精確值取決於這樣一個多面體的面逼近正多邊形的程度。


== 例子 ==
== 例子 ==
{{clear}}
{| class="wikitable sortable"
{| class="wikitable sortable"
! 名稱<br>[[康威多面體表示法]]!! 圖像!!{{link-en|頂點布局|Vertex configuration}}!! 頂點!! 邊!! 面!! F<sub>3</sub>!! F<sub>4</sub>!! F<sub>5</sub>!! F<sub>6</sub>!! F<sub>8</sub>!! F<sub>10</sub>!! F<sub>12</sub>!! {{link-en|球面對稱群列表|List of spherical symmetry groups|對稱性}}
! 名稱<br>[[康威多面體表示法]]!! 圖像!!{{link-en|頂點布局|Vertex configuration}}!! 頂點!! 邊!! 面!! F<sub>3</sub>!! F<sub>4</sub>!! F<sub>5</sub>!! F<sub>6</sub>!! F<sub>8</sub>!! F<sub>10</sub>!! F<sub>12</sub>!! {{link-en|球面對稱群列表|List of spherical symmetry groups|對稱性}}
|- align=center
|- align=center
| [[三側錐三角柱#對偶多面體|底面截角]][[雙三角錐]]<br>[https://levskaya.github.io/polyhedronisme/?recipe=C100A1t4dP3 t4dP3]||[[File:Associahedron.gif|80px]]|| 2 (5.5.5)<br>12 (4.5.5)|| 14|| 21|| 9|| || 3|| 6|||||||||| Dih<sub>3</sub><br>order 12
| [[三側錐三角柱#對偶多面體|底面截角]][[雙三角錐]]<br/>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C100A1t4dP3|2=t4dP3|type=ext}}||[[File:Associahedron.gif|80px]]|| 2 (5.5.5)<br>12 (4.5.5)|| 14|| 21|| 9|| || 3|| 6|||||||||| Dih<sub>3</sub><br/>12階
|- align=center
|- align=center
| [[截角三角化四面體]]<br>[https://levskaya.github.io/polyhedronisme/?recipe=C100A1t6kT t6kT]
| [[截角三角化四面體]]<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C100A1t6kT|2=t6kT|type=ext}}
|[[File:Truncated triakis tetrahedron.png|80px]]
|[[File:Truncated triakis tetrahedron.png|80px]]
|4 (5.5.5)<br>24 (5.5.6)
|4 (5.5.5)<br>24 (5.5.6)
第27行： 第36行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>, [3,3]<br>order 24
| ''T''<sub>d</sub>, [3,3]<br>24階
|- align=center
|- align=center
|[[五邊形六邊形五角十二面七十四面體]]
|[[五邊形六邊形五角十二面七十四面體]]
第42行： 第51行：
|
|
|
|
| ''T''<sub>h</sub>, [3<sup>+</sup>,4]<br>order 24
| ''T''<sub>h</sub>, [3<sup>+</sup>,4]<br>24階
|- align=center
|- align=center
| [[倒角立方體]]<br>[https://levskaya.github.io/polyhedronisme/?recipe=C100A1cC cC]
| [[倒角立方體]]<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C100A1cC|2=cC|type=ext}}
|[[File:Truncated rhombic dodecahedron.png|80px]]
|[[File:Truncated rhombic dodecahedron.png|80px]]
| 24 (4.6.6)<br>8 (6.6.6)
| 24 (4.6.6)<br>8 (6.6.6)
第57行： 第66行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''O''<sub>h</sub>, [4,3]<br>order 48
| ''O''<sub>h</sub>, [4,3]<br>48階
|- align=center
|- align=center
| --
| --
第72行： 第81行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>6h</sub>, [6,2]<br>order 24
| ''D''<sub>6h</sub>, [6,2]<br>24階
|- align=center
|- align=center
| --
| --
第87行： 第96行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>3h</sub>, [3,2]<br>order 12
| ''D''<sub>3h</sub>, [3,2]<br>12階
|- align=center
|- align=center
| [[四階十二面體]]
| [[四階十二面體]]
第102行： 第111行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>, [3,3]<br>order 24
| ''T''<sub>d</sub>, [3,3]<br>24階
|- align=center
|- align=center
| [[部分截半截角八面體]]
| [[部分截半截角八面體]]
第119行： 第128行：
| ''O''<sub>h</sub>, [4,3]
| ''O''<sub>h</sub>, [4,3]
|- align=center
|- align=center
| [[倒角十二面體]]<br>[https://levskaya.github.io/polyhedronisme/?recipe=C100A1cD cD]
| [[倒角十二面體]]<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C100A1cD|2=cD|type=ext}}
|[[File:Truncated rhombic triacontahedron.png|80px]]
|[[File:Truncated rhombic triacontahedron.png|80px]]
| 60 (5.6.6)<br>20 (6.6.6)
| 60 (5.6.6)<br>20 (6.6.6)
第132行： 第141行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''I''<sub>h</sub>, [5,3]<br>order 120
| ''I''<sub>h</sub>, [5,3]<br>120階
|- align=center
|- align=center
| [[截半截角二十面體]]<br>[https://levskaya.github.io/polyhedronisme/?recipe=C400A1atI atI]
| [[截半截角二十面體]]<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C400A1atI|2=atI|type=ext}}
| [[File:Rectified truncated icosahedron.png|80px]]
| [[File:Rectified truncated icosahedron.png|80px]]
| 60 (3.5.3.6)<br>30 (3.6.3.6)
| 60 (3.5.3.6)<br>30 (3.6.3.6)
第147行： 第156行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''I''<sub>h</sub>, [5,3]<br>order 120
| ''I''<sub>h</sub>, [5,3]<br>120階
|- align=center
|- align=center
| 截角截角二十面體<br>[https://levskaya.github.io/polyhedronisme/?recipe=C1000ttI ttI]
| 截角截角二十面體<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C1000ttI|2=ttI|type=ext}}
| [[File:Truncated truncated icosahedron.png|80px]]
| [[File:Truncated truncated icosahedron.png|80px]]
| 120 (3.10.12)<br>60 (3.12.12)
| 120 (3.10.12)<br>60 (3.12.12)
第162行： 第171行：
| 12
| 12
| 20
| 20
| ''I''<sub>h</sub>, [5,3]<br>order 120
| ''I''<sub>h</sub>, [5,3]<br>120階
|- align=center
|- align=center
| 擴展截角二十面體<br>[https://levskaya.github.io/polyhedronisme/?recipe=C1000aatI etI]
| 擴展截角二十面體<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C1000aatI|2=etI|type=ext}}
| [[File:Expanded truncated icosahedron.png|80px]]
| [[File:Expanded truncated icosahedron.png|80px]]
| 60 (3.4.5.4)<br>120 (3.4.6.4)
| 60 (3.4.5.4)<br>120 (3.4.6.4)
第177行： 第186行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''I''<sub>h</sub>, [5,3]<br>order 120
| ''I''<sub>h</sub>, [5,3]<br>120階
|- align=center
|- align=center
| Snub rectified truncated icosahedron<br>[https://levskaya.github.io/polyhedronisme/?recipe=C1000stI stI]
| 扭稜截角二十面體<br>{{AnyLink|1=https://levskaya.github.io/polyhedronisme/?recipe=C1000stI|2=stI|type=ext}}
| [[File:Snub rectified truncated icosahedron.png|80px]]
| [[File:Snub rectified truncated icosahedron.png|80px]]
| 60 (3.3.3.3.5)<br>120 (3.3.3.3.6)
| 60 (3.3.3.3.5)<br>120 (3.3.3.3.6)
第192行： 第201行：
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''I'', [5,3]<sup>+</sup><br>order 60
| ''I'', [5,3]<sup>+</sup><br>60階
|}



{| class="wikitable"
! 名稱
! 圖像
![[頂點圖]]
! V
! E
! F
! F<sub>3</sub>
! F<sub>4</sub>
! F<sub>5</sub>
! F<sub>6</sub>
! F<sub>8</sub>
! F<sub>9</sub>
! F<sub>10</sub>
! [[三維點群|對稱群]]
|-
| [[截角三角化四面體]]
|[[Image:Truncated triakis tetrahedron.png|80px]]
|4 (5.5.5)<BR>24 (5.5.6)
| 28
| 42
| 16
| &nbsp;
| &nbsp;
| 12
| 4
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>
|-
| --
| [[Image:Dh3 symmetry dodecahedral nearmiss johnson.png|80px]]
|6 (5.5.5)<br>9 (3.5.3.5)<br>12 (3.3.5.5)
| 27
| 51
| 26
| 14
| &nbsp;
| 12
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>3h</sub>
|-
| [[四階十二面體]]
|[[Image:Tetrated Dodecahedron.gif|80px]]
|4 (5.5.5)<BR>12 (3.5.3.5)<BR>12 (3.3.5.5)
| 28
| 54
| 28
| 16
| &nbsp;
| 12
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>
|-
| --
| [[Image:Hexpenttri near-miss Johnson solid.png|80px]]
|12 (5.5.6)<BR>6 (3.5.3.5)<BR>12 (3.3.5.5)
| 30
| 54
| 26
| 12
| &nbsp;
| 12
| 2
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>6h</sub>

|}
|}


== 共面擬詹森多面體 ==
== 共面擬詹森多面體 ==
有些未能成為詹森多面體的候選多面體是因為其存在有兩個以上共面的面，其也可以算是全部由正多邊形組成的凸多面體，只是其凸為非嚴格凸。<ref name="Robert R Tupelo-Schneck conditional edges">{{cite web|url= http://tupelo-schneck.org/polyhedra/|title= Convex regular-faced polyhedra with conditional edges|author= Robert R Tupelo-Schneck|access-date= 2023-01-31|archive-date= 2021-08-18|archive-url= https://web.archive.org/web/20210818142541/http://tupelo-schneck.org/polyhedra/|dead-url= no}}</ref>這些多面體可被看做是凸的面且非常接近正多邊形。這些立體通常有無限多種，但若約定所有頂點要位於頂角處，不能位於面（共面的一組面視為同一個面）的內部，則滿足條件的立體只有78個，可以視為詹森多面體的自然推廣<ref name="Robert R Tupelo-Schneck conditional edges"/>（參見[[#條件邊正多邊形凸多面體|條件邊正多邊形凸多面體]]）。
有些未能成為詹森多面體的候選多面體是因為其存在有兩個以上共面的面。這些多面體可被看做是凸的面切非常接近正多邊形。


例如：
例如：
3.3...
3.3...：
{{see also|三角面多面體#非嚴格凸的情況}}
<gallery>
<gallery>
File:Digonal orthobicupola.png|(菱形柱)
File:Digonal orthobicupola.png|同相雙三角柱<br/>（菱形柱）
File:Tet-oct-wedge.png|楔形體
File:Tet-oct-wedge.png|楔形體
File:Gyroelongated triangular bipyramid.png|([[三方偏方面體]])
File:Gyroelongated triangular bipyramid.png|二側錐八面體<br/>（[[三方偏方面體]]）
File:Augmented_octahedron.png|正三角錐反角柱
File:Augmented_octahedron.png|正三角錐反角柱
File:Triangulated monorectified tetrahedron.png|
File:Triangulated monorectified tetrahedron.png|三側錐八面體
File:TetOct2_solid2.png|[[長八面體]]
File:TetOct2_solid2.png|{{link-en|長八面體|Elongated octahedron}}
File:Triangulated_tetrahedron.png|
File:Triangulated_tetrahedron.png|四側錐八面體
File:Augmented hexagonal antiprism flat.png|[[正六角錐反角柱]]<BR>([[正六角反柱體]])
File:Augmented hexagonal antiprism flat.png|[[正六角錐反角柱]]<BR>（[[正六角反棱柱]]）
File:Augmented triangular cupola.png|
File:Augmented triangular cupola.png|
File:Triangulated_truncated_triangular_bipyramid.png|
File:Triangulated_truncated_triangular_bipyramid.png|
File:Double_diminished_icosahedron.png|[[十八面體]]
File:Double_diminished_icosahedron.png|[[邊收縮二十面體]]<br/>[[十八面體]]
File:Triangulated truncated tetrahedron.png|([[截角四面體]])
File:Triangulated truncated tetrahedron.png|四側錐截角四面體<br/>（[[截角四面體]]）
File:Triangulated truncated octahedron.png|([[截角八面體]])
File:Triangulated truncated octahedron.png|八側錐截角八面體<br/>（[[截角八面體]]）
File:Triangulated hexagonal prism.png|([[六角柱]])
File:Triangulated hexagonal prism.png|雙六角錐柱<br/>（[[六角柱]]）
File:Augmented triangular cupula.png|([[正三角帳塔]])
File:Augmented triangular cupula.png|正三角帳塔錐<br/>（[[正三角帳塔]]）
</gallery>
</gallery>
4.4.4.4
4.4.4.4：
<gallery>
<gallery>
File:Partial cubic honeycomb.png|[[正方形二十四面體]]<BR>([[正方體]])
File:Partial cubic honeycomb.png|[[正方形二十四面體]]<BR>（[[正方體]]）
</gallery>
</gallery>
3.4.6.4:
3.4.6.4：
<gallery>
<gallery>
File:Hexagonal cupola flat.png|正六角帳塔<BR>(退化)
File:Hexagonal cupola flat.png|正六角帳塔<BR>（退化）
</gallery>
</gallery>

=== 條件邊[[正多邊形多面體|正多邊形凸多面體]] ===
{{main|條件邊正多邊形凸多面體}}
{{Geometric Shape Example
|shape class=條件邊[[正多邊形凸多面體]]
|Green_augmented_bilunabirotunda.svg|name=[[側錐雙新月雙罩帳]]|size=121
|Gyroelongated_triangular_bipyramid.png|name2=二側錐八面體
|Augmented octahedron.png|name3=正三角錐反角柱
|Elongated_digonal_gyrobicupola.png|name4=[[柱化異相雙三角柱]]|size4=90
}}
若將[[詹森多面體]]的條件放寬成允許面兩兩共面，且所有[[頂點 (幾何)|頂點]]都要嚴格位於頂角上，不能有邊兩兩共線的情況（若允許邊兩兩共線，則結果會有無窮多種情況），也不能夠有頂點位於共面區域內部的情況，則能夠再列出有限個有此特性的立體。條件邊（{{lang|en|conditional edges}}）指的是對應棱的二面角為平角的邊。<ref name="Robert R Tupelo-Schneck conditional edges"/>在這條件下，能允許互相共面的面有正三角形與正三角形（3+3）、正三角形與正方形（3+4）、正三角形與正五邊形（3+5）、正方形和兩個位於對側的正三角形（3+4+3）、正五邊形和兩個不相鄰的正三角形（3+5+3），也就是說，這些立體除了有正多邊形面外，也會存在上述組合之形狀的面。<ref name="Robert R Tupelo-Schneck Regular-faced Polyhedra">{{cite web | url= http://tupelo-schneck.org/polyhedra/background.html | title= Regular-faced Polyhedra | author= Robert R Tupelo-Schneck | access-date= 2023-02-01 | archive-date= 2022-11-14 | archive-url= https://web.archive.org/web/20221114153406/http://tupelo-schneck.org/polyhedra/background.html | dead-url= no }}</ref>這類立體一共有78個。<ref name="Robert R Tupelo-Schneck conditional edges"/>和詹森多面體一樣，這些立體除了一些基本立體外，都能夠用柱體、錐體和28種立體互相組合而成。<ref name="Robert R Tupelo-Schneck Regular-faced Polyhedra"/>


== 參見 ==
== 參見 ==

2024年1月15日 (一) 03:12的最新版本

擬詹森多面體
部分的擬詹森多面體
四階十二面體
四階十二面體 		部分截半截角八面體
部分截半截角八面體
五邊形六邊形 五角十二面七十四面體
五邊形六邊形
五角十二面七十四面體 		截角三角化四面體
截角三角化四面體

在幾何學中，擬詹森多面體是嚴格凸多面體，其面幾乎都是正多邊形，但其中有部分或全部的面不是正多邊形但很接近正多邊形。 而擬詹森多面體經常會在正多邊形與非正多邊形之間有物理構造上可以忽略的微小差異[1]。近似的精確值取決於這樣一個多面體的面逼近正多邊形的程度。

例子

[编辑]
名稱
康威多面體表示法		 圖像 頂點布局（英语：Vertex configuration） 頂點 邊 面 F3 F4 F5 F6 F8 F10 F12 對稱性（英语：List of spherical symmetry groups）
底面截角雙三角錐
t4dP3		 2 (5.5.5)
12 (4.5.5)		 14 21 9 3 6 Dih3
12階
截角三角化四面體
t6kT 		4 (5.5.5)
24 (5.5.6) 		28 42 16     12 4       Td, [3,3]
24階
五邊形六邊形五角十二面七十四面體 12 (3.5.3.6)
24 (3.3.5.6)
24 (3.3.3.3.5) 		60 132 74 56 12 6 Th, [3+,4]
24階
倒角立方體
cC 		24 (4.6.6)
8 (6.6.6) 		32 48 18   6   12       Oh, [4,3]
48階
-- 12 (5.5.6)
6 (3.5.3.5)
12 (3.3.5.5) 		30 54 26 12   12 2       D6h, [6,2]
24階
-- 6 (5.5.5)
9 (3.5.3.5)
12 (3.3.5.5) 		27 51 26 14   12         D3h, [3,2]
12階
四階十二面體 4 (5.5.5)
12 (3.5.3.5)
12 (3.3.5.5) 		28 54 28 16   12         Td, [3,3]
24階
部分截半截角八面體 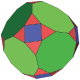24 (3.4.3.9)
24 (3.9.9) 		38 84 48 24 6           Oh, [4,3]
倒角十二面體
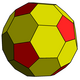
cD 		60 (5.6.6)
20 (6.6.6) 		80 120 42     12 30       Ih, [5,3]
120階
截半截角二十面體
atI 		60 (3.5.3.6)
30 (3.6.3.6) 		90 180 92 60   12 20       Ih, [5,3]
120階
截角截角二十面體
ttI 		120 (3.10.12)
60 (3.12.12) 		180 270 92 60         12 20 Ih, [5,3]
120階
擴展截角二十面體
etI 		60 (3.4.5.4)
120 (3.4.6.4) 		180 360 182 60 90 12 20       Ih, [5,3]
120階
扭稜截角二十面體
stI 		60 (3.3.3.3.5)
120 (3.3.3.3.6) 		180 450 272 240   12 20       I, [5,3]+
60階

共面擬詹森多面體

[编辑]

有些未能成為詹森多面體的候選多面體是因為其存在有兩個以上共面的面，其也可以算是全部由正多邊形組成的凸多面體，只是其凸為非嚴格凸。[2]這些多面體可被看做是凸的面且非常接近正多邊形。這些立體通常有無限多種，但若約定所有頂點要位於頂角處，不能位於面（共面的一組面視為同一個面）的內部，則滿足條件的立體只有78個，可以視為詹森多面體的自然推廣[2]（參見條件邊正多邊形凸多面體）。

例如： 3.3...：

参见：三角面多面體 § 非嚴格凸的情況

4.4.4.4：

3.4.6.4：

  • 正六角帳塔 （退化）
    正六角帳塔
    （退化）

條件邊正多邊形凸多面體

[编辑]
主条目：條件邊正多邊形凸多面體
條件邊正多邊形凸多面體
部分的條件邊正多邊形凸多面體
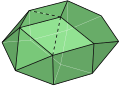側錐雙新月雙罩帳
側錐雙新月雙罩帳 		二側錐八面體
二側錐八面體
正三角錐反角柱
正三角錐反角柱 		柱化異相雙三角柱
柱化異相雙三角柱

若將詹森多面體的條件放寬成允許面兩兩共面，且所有頂點都要嚴格位於頂角上，不能有邊兩兩共線的情況（若允許邊兩兩共線，則結果會有無窮多種情況），也不能夠有頂點位於共面區域內部的情況，則能夠再列出有限個有此特性的立體。條件邊（conditional edges）指的是對應棱的二面角為平角的邊。[2]在這條件下，能允許互相共面的面有正三角形與正三角形（3+3）、正三角形與正方形（3+4）、正三角形與正五邊形（3+5）、正方形和兩個位於對側的正三角形（3+4+3）、正五邊形和兩個不相鄰的正三角形（3+5+3），也就是說，這些立體除了有正多邊形面外，也會存在上述組合之形狀的面。[3]這類立體一共有78個。[2]和詹森多面體一樣，這些立體除了一些基本立體外，都能夠用柱體、錐體和28種立體互相組合而成。[3]

參見

[编辑]

參考文獻

[编辑]
  1. ^ Kaplan, Craig S.; Hart, George W., Symmetrohedra: Polyhedra from Symmetric Placement of Regular Polygons, Bridges: Mathematical Connections in Art, Music and Science (PDF), 2001 [2014-05-01], （原始内容存档 (PDF)于2015-09-23） .
  2. ^ 2.0 2.1 2.2 2.3 Robert R Tupelo-Schneck. Convex regular-faced polyhedra with conditional edges. [2023-01-31]. （原始内容存档于2021-08-18）. 
  3. ^ 3.0 3.1 Robert R Tupelo-Schneck. Regular-faced Polyhedra. [2023-02-01]. （原始内容存档于2022-11-14）. 
检索自“https://zh.wikipedia.org/zhwiki/w/index.php?title=擬詹森多面體&oldid=80498770”