截角二十面體：修订间差异

截角二十面體圖
截角二十面體圖
	6邊形置中心的施萊格爾圖（英语：Schlegel_diagram）
顶点	60
边	90
自同构群	120
色数	3
属性	立方體（英语：Cubic graph）、哈密顿、正則、零對稱性（英语：Zero-symmetric graph）
	查; 论; 编;

截角二十面體
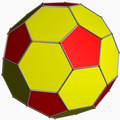
	; （点此查看旋转模型）
類別	阿基米德立體; 半正多面體; 戈德堡多面體
對偶多面體	五角化十二面體
識別
名稱	截角二十面體
參考索引	U25, C27, W9
鮑爾斯縮寫; （verse-and-dimensions的wikia：Bowers acronym）	ti
數學表示法
考克斯特符號; （英语：Coxeter-Dynkin diagram）
施萊夫利符號	t{3,5}; t0,1{3,5}
威佐夫符號; （英语：Wythoff symbol）	2 5 \| 3
康威表示法	tI
性質
面	32
邊	90
頂點	60
歐拉特徵數	F=32, E=90, V=60 （χ=2）
二面角	6-6: 138.189685°; 6-5: 142.62085°
組成與佈局
面的種類	正五邊形 ; 正六邊形
面的佈局; （英语：Face configuration）	12{5}+20{6}
頂點圖	5.6.6
對稱性
對稱群	Ih（英语：Icosahedral symmetry）, H3, [5,3], (*532), order 120
旋轉對稱群; （英語：Rotation_groups）	I（英语：Icosahedral symmetry）, [5,3]+, (532), order 60
特性
	半正、凸
圖像
	; 5.6.6; （頂點圖）
; 五角化十二面體; （對偶多面體）	; （展開圖）
	查; 论; 编;

以互动方式浏览历史

←上一版本

删除的内容添加的内容

可视化wikitext

行内

2024年5月26日 (日) 01:06的最新版本

在幾何學中，截角二十面體是一種由12個正五邊形和20個正六邊形所組成的凸半正多面體，同時具有每個三面角等角和每條邊等長的性質，因此屬於阿基米德立體^[1]，但由於其並非所有面全等因此不能算是正多面體。由於其包含了正五邊形和六邊形面，因此也是一種戈德堡多面体^[2]，其對偶多面體為五角化十二面體。這種結構最早由列奥纳多·达·芬奇給予描述，後來出現於許多藝術創作和學術研究中。自1970年墨西哥足球世界杯之後，這種形狀成為了足球的代表性形狀^[3]^[4]，並且會在六邊形塗上白色、五邊形塗上黑色。在科學領域中，這種形狀亦有許多用途，例如建築學家巴克明斯特·富勒提出的網格球頂（英语：Geodesic dome）結構，甚至在核子武器的引爆技術上也有使用這種形狀的設計。巴克明斯特富勒烯分子（C₆₀）也是這種形狀。

歷史

這種形狀的骨架結構最早由列奥纳多·达·芬奇給予描述^[5]，雖然阿基米德被認為是最早想出十三種阿基米德立體的學者，這十三種立體中也包括了截角二十面體^[6]，但並未留下明確直接提及此形狀的文獻記錄。阿尔布雷希特·丢勒也重現了一個類似截角二十面體的多面體，包含12個五角形和20個六角形面，但是沒有明確的文獻記錄^[7]^[8]，不過部分文獻認為這是最早的截角二十面體圖像^[9]，不過這個時候還沒有「截角」的概念。直到了16、17世紀時，约翰内斯·开普勒才引入了「截角」的概念^[9]，才得以將這個形狀與正二十面體聯繫起來。自1970年墨西哥世界杯之後，截角二十面體成為足球的代表性形狀。^[3]^[4]

性質

截角二十面體是一種半正多面體，由五邊形和六邊形組成^[10]，每個頂點都是兩個六邊形和一個五邊形的公共頂點^[11]，在頂點圖中可計為5.6.6，因此具有點可遞的性質。由於其可以藉由正二十面體透過截角變換，變換而成，因此稱為截角二十面體^[12]。由於此原因，截角二十面體在施萊夫利符號中可以用t{3,5}來表示，其中，t表示截角變換，{3,5}表示正二十面體（每個頂點都是五個三角形的公共頂點）。^[13]

分類

在幾何學中，截角二十面體是一種半正多面體，由於其具有點可遞的性質，因此屬於阿基米德立體^[1]。它由12個正五邊形面、20個正六邊形面、60個頂點和90個邊構成。由於包含了正五邊形和六邊形面因此也是一種戈德堡多面体，在戈德堡符號中可用GP_V(1,1) 或 {5+,3}_1,1表示^[2]。而1983年時，溫尼爾在他的書《多面體模型》中列出許多多面體模型，其中也收錄了此種形狀，並給予編號W₉^[13]。

組成

截角二十面體共有90條棱和60個頂點^[14]，正二十面體是由20個正三角形組成。把正二十面體的棱做三等分，則20個正三角形的面就得到了20個正六邊形；同時把正二十面體的所有12個頂點削去^[15]，則每個頂點由上述三等分點形成的正五邊形代替^[12]^[16]。這就形成了截角二十面體^[17]。由於正二十面體有20個正三角形的面，30條棱。每條棱做三等分則有2個分割點，由此削去正二十面體所有12個頂點後得到的截角二十面體有60個頂點。^[12]

面的組成

截角二十面體由32個面組成，在這32個面中，共包含了12個正五邊形面和20個正六邊形面，其頂角皆為三面角^[18]，由2個六邊形和一個五邊形組成，換句話說，即每個頂點都是2個六邊形和一個五邊形的公共頂點，其頂點圖可以計為5.6.6^[19]。另外，在這個結構中，五邊形面彼此不相鄰。^[20]

尺寸

若以a表示棱長，則截角二十面體的外接球半徑為^[21]：

{a \over 2}{\sqrt {9\phi +10}}\approx 2.4780~a

關於截角二十面體的體積，由於截角二十面體已知可以透過切去正二十面體的十二個頂點來構造，並且已知目標立體為等邊多面體，因此可以知道被切下來的12個錐體是等邊正五角錐。所以截角二十面體的體積可以透過正二十面體的體積減去12個錐體是等邊正五角錐來計算^[21]，其結果為^[22]：

體積：

V={1 \over 4}(125+43{\sqrt {5}})~a^{3}\approx 55.2877~a^{3}

而關於截角二十面體的表面積，已知截角二十面體由12個正五邊形和20正六邊形所組成，因此截角二十面體的表面積可以透過12倍正五邊形面積與20倍正六邊形面積的和來計算^[21]，其結果為^[22]：

表面積：

{\begin{aligned}A&=\left(20\cdot {\frac {3}{2}}{\sqrt {3}}+12\cdot {\frac {5}{4}}{\sqrt {1+{\frac {2}{\sqrt {5}}}}}\right)a^{2}\\&=3(10{\sqrt {3}}+{\sqrt {5}}{\sqrt {5+2{\sqrt {5}}}})~a^{2}\approx 72.6073~a^{2}\end{aligned}}

頂點坐標

邊長為2，幾何中心位於原點的截角二十面體，其頂點的坐標為：^[23]

(0, ±1, ±3φ)

(±1, ±(2 + φ), ±2φ)

(±2, ±(1 + 2φ), ±φ)

其中φ = (1+√5)/2，黃金分割數；此時稜長為 2，外接球半徑為 ${\sqrt {9\phi +10}}$ 。^[24]

二面角

截角二十面體有兩種二面角，一個為兩個六邊形的交角，另一個為五邊形與六邊形的交角^[22]：

其中，兩個六邊形的交角為:

\arccos {\frac {-{\sqrt {5}}}{3}}\approx 138.18968510^{\circ }

^[22]

其中，五邊形與六邊形的交角為:

\arccos {\left(-{\sqrt {\frac {5+2{\sqrt {5}}}{15}}}\right)}\approx 142.62263185^{\circ }

^[22]

另外，其兩個六邊形的共線與五邊形的交角為:

\arccos {\left(-{\sqrt {\frac {5+{\sqrt {5}}}{10}}}\right)}\approx 148.2825255^{\circ }

以及五邊形和六邊形的共線與鄰近的六邊形的交角為144°。

正交投影

截角二十面體有五種具有特殊對稱性的正交投影，分別是以頂點為中心、以邊為中心（兩種）、以六邊形面為中心以及以五邊形面為中心的正交投影。所述後者兩種正交投影，其對稱性對應於A₂ 和 H₂的考克斯特平面^[25]^[26]。

正交投影
投影位置	頂點	五邊形-六邊形稜	六邊形-六邊形稜	六邊形面	五邊形面
立體
圖像
投影對稱性	[2]	[2]	[2]	[6]	[10]
對偶

球面鑲嵌

截角二十面體也可以表示為球面鑲嵌，並通過球極投影，投影到平面上。這個投影是一個等角投影，雖然長度發生改變，但保留了角度資訊。球面鑲嵌上的直線投影到了平面後成為了弧線。其結果與足球十分相似。^[27]^[16]

正交投影	球極平面投影
	以五邊形為中心	以六邊形為中心

應用

截角二十面體在日常生活^[3]、藝術、自然科學和技術等領域中皆有用途。

在科學中

在化學中也有一些分子的形狀為截角二十面體，如富勒烯C₆₀^[8]^[28]，這種分子於1985年被發現，其直徑約為0.71奈米^[29]，由60個碳原子組成，且每個碳原子正好位於截角二十面體的頂點上^[30]。這種形狀也用於核子武器中，聚焦雷管的爆炸衝擊波用的爆縮透鏡（日语：爆縮レンズ），並配置於三位一體和胖子等原子彈中^[31]^[32]。截角二十面體的一種變體也曾用於由聚合材料製成的窩狀車輪胎框的基礎，其使用了富勒的網格球頂的部分幾何結構（截角二十面體的局部），其被龐蒂克汽車部門在1971年至1976年間用於Trans Am和Grand Prix中^[33]。此外，由於這個幾何結構與建築學家巴克明斯特·富勒設計的1967年世界博覽會美國館網格球頂（英语：Geodesic dome）時分相似，為了表達對他的敬意，有時會將其稱為「巴克球」（buckyball）^[34]。

在文化中

截角二十面體的球面鑲嵌被運用在足球和手球的形狀上^[16]，大致上使得截角二十面體被認為是在日常生活中最著名的球面多面體之例子^[3]。這些球類運動所使用的球體皆包含了相同的正五邊形和正六邊形圖案，但由於運動用球內部通常會充氣，使得空氣的壓力令球具有彈性、更加接近球體。足球的模樣自1970年墨西哥世界杯之后为截角二十面體。^[3]^[35]

在藝術中

截角二十面體出現於列奥纳多·达·芬奇繪製於卢卡·帕西奥利的著作《Divina proportione》中的插圖。^[36]

截角二十面體（左）與足球
富勒烯 C₆₀ 分子模型
外型為截角二十面體的氣象雷達
用6061-T6鋁加工而成的截角二十面體
喬治·W·哈特（英语：George W. Hart）的截角二十面體木頭模型。
與截角二十面體對應的球面鑲嵌（截角五階三角形鑲嵌）設計的閃光球（英语：Sparkleball）

截角二十面體圖

在圖論的數學領域中，截角二十面體圖是阿基米得立體中截角二十面體之邊與頂點的圖（英语：n-skeleton）^[43]，其有時被稱為巴克明斯特富勒圖^[44]。共有60個頂點和90條稜，且是立方體（英语：Cubic graph）的阿基米德圖（英语：Archimedean graph）^[45]^[46]^[44]^[43]。

正射投影
五摺對稱性	5邊形置中心的施萊格爾圖（英语：Schlegel_diagram）

參見

正十二面體

參考文獻

^ ^1.0 ^1.1 Cromwell, P. Polyhedra. United Kingdom: CUP hbk (1997), pbk. (1999), Cambridge. 1997: 79–86 Archimedean solids. ISBN 0-521-55432-2 （英语）.
^ ^2.0 ^2.1 Magnus Wenninger（英语：Magnus J. Wenninger）, Spherical Models, Cambridge University Press, 1979, ISBN 978-0-521-29432-4, MR 0552023, （原始内容存档于2008-07-04）（英语） Reprinted by Dover 1999 ISBN 978-0-486-40921-4
^ ^3.0 ^3.1 ^3.2 ^3.3 ^3.4 ^3.5 Kotschick, Dieter. The Topology and Combinatorics of Soccer Balls. American Scientist. 2006, 94 (4): 350–357. doi:10.1511/2006.60.350 （英语）.
^ ^4.0 ^4.1 Swart, David; et al, Soccer Ball Symmetry, Proceedings of Bridges 2015: Mathematics, Music, Art, Architecture, Culture (Tessellations Publishing), 2015: 151–158 （英语）
^ Saffaro, L. Cosmoids, Fullerenes and continuous polygons. Taliani, C.; Ruani, G.; Zamboni, R. (编). Proceedings of the First Italian Workshop on Fullerenes: States and Perspectives 2. Singapore: World Scientific. 1992: 55. ISBN 9810210825 （英语）.
^ Grünbaum, Branko, An enduring error, Elemente der Mathematik, 2009, 64 (3): 89–101, MR 2520469, doi:10.4171/EM/120 （英语）
^ Durer, A. German artist who made an early model of a regular truncated icosahedron. 1471–1528 （英语）.
^ ^8.0 ^8.1 Dresselhaus, M. S.; Dresselhaus, G.; Eklund, P. C. Science of fullerenes and carbon nanotubes. San Diego, CA: Academic Press. 1996. ISBN 012-221820-5 （英语）.
^ ^9.0 ^9.1 History of the truncated icosahedron. www2.fkf.mpg.de. [2019-09-10]. （原始内容存档于2017-12-19）（英语）.
^ Martin Henk. Truncated Icosahedron, Electronic Geometry Model No. 2001.02.065. eg-models.de. 2001-02-01 [2019-09-11]. （原始内容存档于2021-06-22）（英语）.
^ Truncated Icosahedron. coolmath.com. [2019-09-10]. （原始内容存档于2019-03-11）（英语）.
^ ^12.0 ^12.1 ^12.2 Murakami, Hidenori and Nishimura, Yoshitaka. Static and dynamic characterization of regular truncated icosahedral and dodecahedral tensegrity modules. International Journal of Solids and Structures (Elsevier). 2001, 38 (50-51): 9359––9381 （英语）.
^ ^13.0 ^13.1 Wenninger, Magnus（英语：Magnus J. Wenninger）. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9 （英语）.
^ Roman E. Maeder. 25: truncated icosahedron. MathConsult AG. [2019-09-10]. （原始内容存档于2019-01-16）（英语）.
^ Kostant, Bertram. Structure of the truncated icosahedron (such as Fullerene or viral coatings) and a 60-element conjugacy class in PSl (2, 11). Proceedings of the National Academy of Sciences (National Acad Sciences). 1994, 91 (24): 11714––11717 （英语）.
^ ^16.0 ^16.1 ^16.2 Paul Bourke, § Soccer ball (Hand ball), Geometry of sports balls, Paul Bourke, January 2017 [2019-09-11], （原始内容存档于2018-07-27）（英语）
^ The Icosahedron and the Truncated Icosahedron. geom.uiuc.edu. [2019-09-10]. （原始内容存档于2019-03-09）（英语）.
^ Rene K. Mueller. Geodesic Polyhedra: Geodesic Truncated Icosahedron. simply differently. 2012-10-28 [2019-09-11]. （原始内容存档于2014-10-22）（英语）.
^ Truncated icosahedron, tI. polyhedra.tessera.li. [2019-09-11]. （原始内容存档于2022-03-28）（英语）.
^ A. Ramachandran, The Truncated Icosahedron –An Iconic 3-D Shape, Azim Premji University At Right Angles, March 2019: 69–71 [2019-09-11], （原始内容存档于2021-06-22）（英语）
^ ^21.0 ^21.1 ^21.2 Harish Chandra Rajpoot Rajpoot, HCR, Mathematical analysis of truncated icosahedron & identical football, Applications of HCR's Theory of Polygon & HCR's formula for platonic solids, 2014 （英语）
^ ^22.0 ^22.1 ^22.2 ^22.3 ^22.4 Archimedean Solids: Truncated Icosahedron. dmccooey.com. [2018-01-06]. （原始内容存档于2017-12-31）（英语）.
^ ^23.0 ^23.1 The Truncated Icosahedron. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-02-14）（英语）.
^ ^24.0 ^24.1 Weisstein, Eric W. (编). Icosahedral group. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2019-09-06]. （原始内容存档于2019-05-14）（英语）.
^ 約翰·史坦布里奇（英语：John Stembridge）. Coxeter Planes. math.lsa.umich.edu. （原始内容存档于2018-02-10）（英语）.
^ 約翰·史坦布里奇（英语：John Stembridge）. More Coxeter Planes. math.lsa.umich.edu. （原始内容存档于2017-08-21）（英语）.
^ Huybers, P, In search of the roundest soccer ball, Int. Conference on Adaptability in Design and Construction, Eindhoven, 2006: 3––5 （英语）
^ Kroto, H. W.; Health, J. R.; O'Brien, S. C.; Curl, R. F.; Smalley, R. E. C₆₀: Buckminsterfullerene. Nature. 1985, 318 (6042): 162–163. Bibcode:1985Natur.318..162K. doi:10.1038/318162a0 （英语）.
^ Katz, E. A. Fullerene Thin Films as Photovoltaic Material. Sōga, Tetsuo (编). Nanostructured materials for solar energy conversion. Elsevier. 2006: 364 [2019-09-10]. ISBN 978-0-444-52844-5. （原始内容存档于2021-03-18）（英语）.
^ Ozaki, Masa-aki and Taahashi, Akira. On electronic states and bond lengths of the truncated icosahedral C₆₀ molecule. Chemical physics letters (Elsevier). 1986, 127 (3): 242––244 （英语）.
^ Rhodes, Richard. Dark Sun: The Making of the Hydrogen Bomb. Touchstone Books. 1996: 195. ISBN 0-684-82414-0 （英语）.
^ 山田克哉, 原子爆弾 : その理論と歴史, ブルーバックス（日语：ブルーバックス）, 講談社, 1996年7月, ISBN 4-06-257128-5, ISBN ISBN 978-4-06-257128-9 （日语）
^ Rotella, R. The Definitive Firebird & Trans Am Guide: 1970 1/2 - 1981: Detailed Facts, Figures & Features of Pontiac's Legendary Firebirds & Trans Ams. CarTech. 2018: 64. ISBN 9781613253212. LCCN 2017014572 （英语）.
^ Richard E. Smalley, Robert F. Curl, Jr., and Harold W. Kroto. Chemical Heritage Foundation. [2016-02-03]. （原始内容存档于2016-01-19）（英语）.
^ Tarnai, Tibor and Lengyel, András. The Truncated Icosahedron as an Inflatable Ball. Periodica Polytechnica Architecture. 2018, 49 (2): 99––108 （英语）.
^ Luca Pacioli. Divina proportione. Republic of Venice: Paganini (Venice). 1509 （意大利语）.
^ Weisstein, Eric W. Echidnahedron. MathWorld--A Wolfram Web Resource. [2019-09-06]. （原始内容存档于2018-10-03）（英语）.
^ Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294-296) （英文）
^ Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999 ISBN 0-486-40919-8 (Chapter 10: Regular honeycombs in hyperbolic space, Summary tables II,III,IV,V, p212-213) （英文）
^ Klitzing, Richard. 4D uniform polytopes (polychora). bendwavy.org. , (x3x3x5o - grix) （英文）
^ The Cantitruncated 600-cell. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-05-03）（英语）.
^ The Bitruncated 120-cell. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-02-05）（英语）.
^ ^43.0 ^43.1 Kostant, Bertram. "The graph of the truncated icosahedron and the last letter of Galois" (PDF). Notices of the AMS. 1995, 42 (9): 959––968 [2018-02-01]. （原始内容存档 (PDF)于2017-01-15）（英语）.
^ ^44.0 ^44.1 Chris Godsil, Gordon F. Royle. "Algebraic Graph Theory". Graduate Texts in Mathematics. New York: Springer-Verlag. 2001: 211. ISBN 978-0387952208 （英语）.
^ Read, R. C.; Wilson, R. J., An Atlas of Graphs, Oxford University Press: 268, 1998 （英语）
^ Weisstein, Eric W. TruncatedIcosahedralGraph. MathWorld--A Wolfram Web Resource. [2019-09-06]. （原始内容存档于2019-05-18）（英语）.

參考書目

Williams, Robert. The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. 1979. ISBN 0-486-23729-X. (Section 3-9)
Cromwell, P. Polyhedra. United Kingdom: Cambridge. 1997: 79–86 Archimedean solids. ISBN 0-521-55432-2.

外部連結

埃里克·韦斯坦因, 截角二十面體（參閱阿基米德立體）於MathWorld（英文）
- 埃里克·韦斯坦因. Truncated icosahedral graph. MathWorld.
Klitzing, Richard. 3D convex uniform polyhedra x3x5o - ti. bendwavy.org.
Editable printable net of a truncated icosahedron with interactive 3D view （页面存档备份，存于互联网档案馆）
The Uniform Polyhedra （页面存档备份，存于互联网档案馆）
Virtual Reality Polyhedra （页面存档备份，存于互联网档案馆） The Encyclopedia of Polyhedra
3D paper data visualization World Cup ball （页面存档备份，存于互联网档案馆）

[Cromwell_1997-3] 1.0 ^1.1 Cromwell, P. Polyhedra. United Kingdom: CUP hbk (1997), pbk. (1999), Cambridge. 1997: 79–86 Archimedean solids. ISBN 0-521-55432-2 （英语）.

[Wenninger_1979-4] 2.0 ^2.1 Magnus Wenninger（英语：Magnus J. Wenninger）, Spherical Models, Cambridge University Press, 1979, ISBN 978-0-521-29432-4, MR 0552023, （原始内容存档于2008-07-04）（英语） Reprinted by Dover 1999 ISBN 978-0-486-40921-4

[The_Topology_and_Combinatorics_of_Soccer_Balls-5] 3.0 ^3.1 ^3.2 ^3.3 ^3.4 ^3.5 Kotschick, Dieter. The Topology and Combinatorics of Soccer Balls. American Scientist. 2006, 94 (4): 350–357. doi:10.1511/2006.60.350 （英语）.

[inproceedings_swart2015soccer-6] 4.0 ^4.1 Swart, David; et al, Soccer Ball Symmetry, Proceedings of Bridges 2015: Mathematics, Music, Art, Architecture, Culture (Tessellations Publishing), 2015: 151–158 （英语）

[Saffaro-7] Saffaro, L. Cosmoids, Fullerenes and continuous polygons. Taliani, C.; Ruani, G.; Zamboni, R. (编). Proceedings of the First Italian Workshop on Fullerenes: States and Perspectives 2. Singapore: World Scientific. 1992: 55. ISBN 9810210825 （英语）.

[8] Grünbaum, Branko, An enduring error, Elemente der Mathematik, 2009, 64 (3): 89–101, MR 2520469, doi:10.4171/EM/120 （英语）

[Durer-9] Durer, A. German artist who made an early model of a regular truncated icosahedron. 1471–1528 （英语）.

[Dresselhaus-10] 8.0 ^8.1 Dresselhaus, M. S.; Dresselhaus, G.; Eklund, P. C. Science of fullerenes and carbon nanotubes. San Diego, CA: Academic Press. 1996. ISBN 012-221820-5 （英语）.

[fullerene/vatican-11] 9.0 ^9.1 History of the truncated icosahedron. www2.fkf.mpg.de. [2019-09-10]. （原始内容存档于2017-12-19）（英语）.

[12] Martin Henk. Truncated Icosahedron, Electronic Geometry Model No. 2001.02.065. eg-models.de. 2001-02-01 [2019-09-11]. （原始内容存档于2021-06-22）（英语）.

[13] Truncated Icosahedron. coolmath.com. [2019-09-10]. （原始内容存档于2019-03-11）（英语）.

[article_murakami2001static-14] 12.0 ^12.1 ^12.2 Murakami, Hidenori and Nishimura, Yoshitaka. Static and dynamic characterization of regular truncated icosahedral and dodecahedral tensegrity modules. International Journal of Solids and Structures (Elsevier). 2001, 38 (50-51): 9359––9381 （英语）.

[Wenninger_1974-15] 13.0 ^13.1 Wenninger, Magnus（英语：Magnus J. Wenninger）. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9 （英语）.

[16] Roman E. Maeder. 25: truncated icosahedron. MathConsult AG. [2019-09-10]. （原始内容存档于2019-01-16）（英语）.

[article_kostant1994structure-17] Kostant, Bertram. Structure of the truncated icosahedron (such as Fullerene or viral coatings) and a 60-element conjugacy class in PSl (2, 11). Proceedings of the National Academy of Sciences (National Acad Sciences). 1994, 91 (24): 11714––11717 （英语）.

[Paul_Bourke_2017-18] 16.0 ^16.1 ^16.2 Paul Bourke, § Soccer ball (Hand ball), Geometry of sports balls, Paul Bourke, January 2017 [2019-09-11], （原始内容存档于2018-07-27）（英语）

[19] The Icosahedron and the Truncated Icosahedron. geom.uiuc.edu. [2019-09-10]. （原始内容存档于2019-03-09）（英语）.

[20] Rene K. Mueller. Geodesic Polyhedra: Geodesic Truncated Icosahedron. simply differently. 2012-10-28 [2019-09-11]. （原始内容存档于2014-10-22）（英语）.

[21] Truncated icosahedron, tI. polyhedra.tessera.li. [2019-09-11]. （原始内容存档于2022-03-28）（英语）.

[22] A. Ramachandran, The Truncated Icosahedron –An Iconic 3-D Shape, Azim Premji University At Right Angles, March 2019: 69–71 [2019-09-11], （原始内容存档于2021-06-22）（英语）

[Harish_2014-23] 21.0 ^21.1 ^21.2 Harish Chandra Rajpoot Rajpoot, HCR, Mathematical analysis of truncated icosahedron & identical football, Applications of HCR's Theory of Polygon & HCR's formula for platonic solids, 2014 （英语）

[dmccooey-24] 22.0 ^22.1 ^22.2 ^22.3 ^22.4 Archimedean Solids: Truncated Icosahedron. dmccooey.com. [2018-01-06]. （原始内容存档于2017-12-31）（英语）.

[buckyball_eusebeia-25] 23.0 ^23.1 The Truncated Icosahedron. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-02-14）（英语）.

[IcosahedralGroup,Mathworld-26] 24.0 ^24.1 Weisstein, Eric W. (编). Icosahedral group. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2019-09-06]. （原始内容存档于2019-05-14）（英语）.

[27] 約翰·史坦布里奇（英语：John Stembridge）. Coxeter Planes. math.lsa.umich.edu. （原始内容存档于2018-02-10）（英语）.

[28] 約翰·史坦布里奇（英语：John Stembridge）. More Coxeter Planes. math.lsa.umich.edu. （原始内容存档于2017-08-21）（英语）.

[inproceedings_huybers2006search-29] Huybers, P, In search of the roundest soccer ball, Int. Conference on Adaptability in Design and Construction, Eindhoven, 2006: 3––5 （英语）

[Kroto-30] Kroto, H. W.; Health, J. R.; O'Brien, S. C.; Curl, R. F.; Smalley, R. E. C₆₀: Buckminsterfullerene. Nature. 1985, 318 (6042): 162–163. Bibcode:1985Natur.318..162K. doi:10.1038/318162a0 （英语）.

[31] Katz, E. A. Fullerene Thin Films as Photovoltaic Material. Sōga, Tetsuo (编). Nanostructured materials for solar energy conversion. Elsevier. 2006: 364 [2019-09-10]. ISBN 978-0-444-52844-5. （原始内容存档于2021-03-18）（英语）.

[article_ozaki1986electronic-32] Ozaki, Masa-aki and Taahashi, Akira. On electronic states and bond lengths of the truncated icosahedral C₆₀ molecule. Chemical physics letters (Elsevier). 1986, 127 (3): 242––244 （英语）.

[33] Rhodes, Richard. Dark Sun: The Making of the Hydrogen Bomb. Touchstone Books. 1996: 195. ISBN 0-684-82414-0 （英语）.

[34] 山田克哉, 原子爆弾 : その理論と歴史, ブルーバックス（日语：ブルーバックス）, 講談社, 1996年7月, ISBN 4-06-257128-5, ISBN ISBN 978-4-06-257128-9 （日语）

[book_rotella2018definitive-35] Rotella, R. The Definitive Firebird & Trans Am Guide: 1970 1/2 - 1981: Detailed Facts, Figures & Features of Pontiac's Legendary Firebirds & Trans Ams. CarTech. 2018: 64. ISBN 9781613253212. LCCN 2017014572 （英语）.

[chemheritage-smalley-36] Richard E. Smalley, Robert F. Curl, Jr., and Harold W. Kroto. Chemical Heritage Foundation. [2016-02-03]. （原始内容存档于2016-01-19）（英语）.

[article_tarnai2018truncated-37] Tarnai, Tibor and Lengyel, András. The Truncated Icosahedron as an Inflatable Ball. Periodica Polytechnica Architecture. 2018, 49 (2): 99––108 （英语）.

[38] Luca Pacioli. Divina proportione. Republic of Venice: Paganini (Venice). 1509 （意大利语）.

[Echidnahedron,Mathworld-39] Weisstein, Eric W. Echidnahedron. MathWorld--A Wolfram Web Resource. [2019-09-06]. （原始内容存档于2018-10-03）（英语）.

[40] Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294-296) （英文）

[41] Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999 ISBN 0-486-40919-8 (Chapter 10: Regular honeycombs in hyperbolic space, Summary tables II,III,IV,V, p212-213) （英文）

[42] Klitzing, Richard. 4D uniform polytopes (polychora). bendwavy.org. , (x3x3x5o - grix) （英文）

[cantitrunc600cell_eusebeia-43] The Cantitruncated 600-cell. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-05-03）（英语）.

[bitrunc120cell_eusebeia-44] The Bitruncated 120-cell. eusebeia.dyndns.org. [2019-09-10]. （原始内容存档于2019-02-05）（英语）.

[article_kostant1995graph-45] 43.0 ^43.1 Kostant, Bertram. "The graph of the truncated icosahedron and the last letter of Galois" (PDF). Notices of the AMS. 1995, 42 (9): 959––968 [2018-02-01]. （原始内容存档 (PDF)于2017-01-15）（英语）.

[Chris_Godsil_2001-46] 44.0 ^44.1 Chris Godsil, Gordon F. Royle. "Algebraic Graph Theory". Graduate Texts in Mathematics. New York: Springer-Verlag. 2001: 211. ISBN 978-0387952208 （英语）.

[47] Read, R. C.; Wilson, R. J., An Atlas of Graphs, Oxford University Press: 268, 1998 （英语）

[TruncatedIcosahedralGraph,Mathworld-48] Weisstein, Eric W. TruncatedIcosahedralGraph. MathWorld--A Wolfram Web Resource. [2019-09-06]. （原始内容存档于2019-05-18）（英语）.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

[16]

[17]

[18]

[19]

[20]

[21]

[22]

[23]

[24]

[25]

[26]

[27]

[28]

[29]

[30]

[31]

[32]

[33]

[34]

[35]

[36]

[37]

[38]

[39]

[40]

[41]

[42]

[43]

[44]

[45]

[46]

@@ 第1行： / 第1行： @@
 {{GA}}{{noteTA
-|G1=Science
+|G1=Math
+|G2=Science
 |1 = zh-cn:列奥纳多; zh-tw:李奧納多; zh-hk:李安納度;
 |2 = zh-cn:达·芬奇; zh-tw:達文西; zh-hk:達文西;
 |3 = zh-cn:芬奇; zh-tw:文西; zh-hk:文西;
 }}
+{{Infobox polyhedron
-{{Polyhedronbox
 | name = 截角二十面體
 | polyhedron = 截角二十面體
 | imagename = Truncatedicosahedron.jpg
 | rotating = Truncatedicosahedron.gif
-| rinfo = (點選檢視旋轉模型)
+| rinfo = （-{zh-hant:點選檢視旋轉模型;zh-hans:点此查看旋转模型;}-）
 | Type = [[阿基米德立體]]<br/>[[半正多面體]]<br/>[[戈德堡多面體]]
 | Coxeter_diagram = {{CDD|node|5|node_1|3|node_1}}
@@ 第16行： / 第17行： @@
 | Edge = 90
 | Vertice = 60
-| Face_type = [[正五邊形]] [[File:Simple_pentagon.svg|20px]]<br/>
+| Face_type = [[正五邊形]] [[File:Simple_pentagon.svg|20px]]<br/>[[正六邊形]] [[File:Orange hexagon.png|25px]]
-[[正六邊形]] [[File:Orange hexagon.png|25px]]
 | Vertice_type = 5.6.6
 | Schläfli = t{3,5}<br/>t<sub>0,1</sub>{3,5}
@@ 第25行： / 第25行： @@
 | Symmetry_group = {{link-en|二十面體群對稱性|Icosahedral symmetry|I<sub>h</sub>}}, H<sub>3</sub>, [5,3], (*532), order 120
 | Index_references = [[均勻多面體|U]]<sub>25</sub>, [[哈罗德·斯科特·麦克唐纳·考克斯特|C]]<sub>27</sub>, [[溫尼爾多面體模型列表|W]]<sub>9</sub>
-| dual = [[五角化十二面體]]
 | Rotation_group = {{link-en|二十面體群對稱性|Icosahedral symmetry|I}}, [5,3]<sup>+</sup>, (532), order 60
-| Dihedral_angle = 6-6: 138.189685°<BR>6-5: 142.62°
+| Dihedral_angle = 6-6: 138.189685°<BR>6-5: 142.62085°
 | Properties = [[半正多面體|半正]]、[[凹凸性 (幾何)|凸]]
 | 3d_image = Truncated_icosahedron.png
@@ 第38行： / 第37行： @@
 == 歷史 ==
 {| align=right
-| [[Image:De divina proportione - Illustration 17, crop.jpg|thumb|1509年繪製於《De divina proportione》的截角二十面體圖形。]]
+| [[Image:De divina proportione - Illustration 17, crop.jpg|thumb|1509年繪製於《De divina proportione》的截角二十面體插圖。]]
 |}
 這種形狀的骨架結構最早由[[列奥纳多·达·芬奇]]給予描述<ref name=Saffaro>{{cite book |last=Saffaro|first=L.| chapter=Cosmoids, Fullerenes and continuous polygons | editor-last1=Taliani | editor-first1=C. | editor-last2=Ruani | editor-first2=G. | editor-last3=Zamboni | editor-first3=R. | script-title = en:Proceedings of the First Italian Workshop on Fullerenes: States and Perspectives| year=1992|volume=2|pages=55|publisher=World Scientific|location=Singapore | isbn=9810210825 |language=en}}</ref>，雖然[[阿基米德]]被認為是最早想出十三種[[阿基米德立體]]的學者，這十三種立體中也包括了截角二十面體<ref>{{citation
@@ 第49行： / 第48行： @@
  | title = An enduring error
  | volume = 64
- | year = 2009 | language=en}}</ref>，但並未留下明確直接提及此形狀的文獻記錄。[[阿尔布雷希特·丢勒]]也重現了一個類似截角二十面體的多面體，包含12個五角形和20個六角形面，但是沒有明確的文獻記錄<ref name=Durer>{{cite news|last=Durer|first=A.|title=German artist who made an early model of a regular truncated icosahedron|date=1471–1528 | language=en}}</ref><ref name=Dresselhaus>{{cite book |last1=Dresselhaus |first1=M. S. |last2=Dresselhaus |first2=G. |last3=Eklund |first3=P. C. |title=Science of fullerenes and carbon nanotubes |date=1996 |publisher=Academic Press |location=San Diego, CA |isbn=012-221820-5 | language=en}}</ref>，不過部分文獻認為這是最早的截角二十面體圖像<ref name="fullerene/vatican">{{Cite web | url = https://www2.fkf.mpg.de/andersen/fullerene/vatican.html | title = History of the truncated icosahedron | publisher = www2.fkf.mpg.de | access-date = 2019-09-10 | archive-url = https://web.archive.org/web/20171219031302/http://www2.fkf.mpg.de/andersen/fullerene/vatican.html | archive-date = 2017-12-19 | dead-url = no  | language=en}}</ref>，不過這個時候還沒有「[[截角 (幾何)|截角]]」的概念。直到了16、17世紀時，[[约翰内斯·开普勒]]才引入了「[[截角 (幾何)|截角]]」的概念<ref name="fullerene/vatican"/>，才得以將這個形狀與[[正二十面體]]聯繫起來。自1970年墨西哥世界杯之後，截角二十面體成為[[足球]]的代表性形狀。<ref name="The Topology and Combinatorics of Soccer Balls"/><ref name="inproceedings swart2015soccer">{{Citation
+ | year = 2009 | language=en}}</ref>，但並未留下明確直接提及此形狀的文獻記錄。[[阿尔布雷希特·丢勒]]也重現了一個類似截角二十面體的多面體，包含12個五角形和20個六角形面，但是沒有明確的文獻記錄<ref name=Durer>{{cite news|last=Durer|first=A.|title=German artist who made an early model of a regular truncated icosahedron|date=1471–1528 | language=en}}</ref><ref name=Dresselhaus>{{cite book |last1=Dresselhaus |first1=M. S. |last2=Dresselhaus |first2=G. |last3=Eklund |first3=P. C. |title=Science of fullerenes and carbon nanotubes |url=https://archive.org/details/scienceoffullere0000dres |date=1996 |publisher=Academic Press |location=San Diego, CA |isbn=012-221820-5 | language=en}}</ref>，不過部分文獻認為這是最早的截角二十面體圖像<ref name="fullerene/vatican">{{Cite web | url = https://www2.fkf.mpg.de/andersen/fullerene/vatican.html | title = History of the truncated icosahedron | publisher = www2.fkf.mpg.de | access-date = 2019-09-10 | archive-url = https://web.archive.org/web/20171219031302/http://www2.fkf.mpg.de/andersen/fullerene/vatican.html | archive-date = 2017-12-19 | dead-url = no  | language=en}}</ref>，不過這個時候還沒有「[[截角 (幾何)|截角]]」的概念。直到了16、17世紀時，[[约翰内斯·开普勒]]才引入了「[[截角 (幾何)|截角]]」的概念<ref name="fullerene/vatican"/>，才得以將這個形狀與[[正二十面體]]聯繫起來。自1970年墨西哥世界杯之後，截角二十面體成為[[足球]]的代表性形狀。<ref name="The Topology and Combinatorics of Soccer Balls"/><ref name="inproceedings swart2015soccer">{{Citation
   |title=Soccer Ball Symmetry
   |author1=Swart, David
@@ 第59行： / 第58行： @@
 == 性質 ==
-截角二十面體是一種[[半正多面體]]，由[[五邊形]]和[[六邊形]]組成<ref>{{Cite web | url = http://www.eg-models.de/models/Lattices_and_Packings/Densest_Polytopes/2001.02.065/_preview.html | title=Truncated Icosahedron, Electronic Geometry Model No. 2001.02.065|author=Martin Henk|website = eg-models.de | date=2001-02-01 |accessdate=2019-09-11 | language=en}}</ref>，每個頂點都是兩個六邊形和一個五邊形的公共頂點<ref>{{Cite web | url = https://www.coolmath.com/reference/polyhedra-truncated-icosahedron | title = Truncated Icosahedron | publisher = coolmath.com | access-date = 2019-09-10 | archive-url = https://web.archive.org/web/20190311070519/http://www.coolmath.com/reference/polyhedra-truncated-icosahedron | archive-date = 2019-03-11 | dead-url = no  | language=en}}</ref>，在[[頂點圖]]中可計為5.6.6，因此具有點可遞的性質。由於其可以藉由正二十面體透過[[截角 (幾何)|截角]]變換，變換而成，因此稱為截角二十面體<ref name="article murakami2001static">{{cite journal |title=Static and dynamic characterization of regular truncated icosahedral and dodecahedral tensegrity modules
+截角二十面體是一種[[半正多面體]]，由[[五邊形]]和[[六邊形]]組成<ref>{{Cite web| url=http://www.eg-models.de/models/Lattices_and_Packings/Densest_Polytopes/2001.02.065/_preview.html| title=Truncated Icosahedron, Electronic Geometry Model No. 2001.02.065| author=Martin Henk| website=eg-models.de| date=2001-02-01| accessdate=2019-09-11| language=en| archive-date=2021-06-22| archive-url=https://web.archive.org/web/20210622111827/http://www.eg-models.de/models/Lattices_and_Packings/Densest_Polytopes/2001.02.065/_preview.html}}</ref>，每個頂點都是兩個六邊形和一個五邊形的公共頂點<ref>{{Cite web | url = https://www.coolmath.com/reference/polyhedra-truncated-icosahedron | title = Truncated Icosahedron | publisher = coolmath.com | access-date = 2019-09-10 | archive-url = https://web.archive.org/web/20190311070519/http://www.coolmath.com/reference/polyhedra-truncated-icosahedron | archive-date = 2019-03-11 | dead-url = no  | language=en}}</ref>，在[[頂點圖]]中可計為5.6.6，因此具有點可遞的性質。由於其可以藉由正二十面體透過[[截角 (幾何)|截角]]變換，變換而成，因此稱為截角二十面體<ref name="article murakami2001static">{{cite journal |title=Static and dynamic characterization of regular truncated icosahedral and dodecahedral tensegrity modules
+  |url=https://archive.org/details/sim_international-journal-of-solids-and-structures_2001-12_38_50-51/page/9359
   |author=Murakami, Hidenori and Nishimura, Yoshitaka
   |journal=International Journal of Solids and Structures
@@ 第66行： / 第66行： @@
   |pages=9359--9381
   |year=2001
-  |publisher=Elsevier | language=en}}</ref>。由於此原因，截角二十面體在施萊夫利符號中可以用t{3,5}來表示，其中，t表示截角變換，{3,5}表示[[正二十面體]]（每個頂點都是五個三角形的公共頂點）。<ref name="Wenninger 1974"/>
+  |publisher=Elsevier | language=en}}</ref>。由於此原因，截角二十面體在施萊夫利符號中可以用t{{mset|3,5}}來表示，其中，t表示截角變換，{{mset|3,5}}表示[[正二十面體]]（每個頂點都是五個三角形的公共頂點）。<ref name="Wenninger 1974"/>
 === 分類 ===
-在[[幾何學]]中，'''截角二十面體'''是一種[[半正多面體]]，由於其具有點可遞的性質，因此屬於[[阿基米德立體]]<ref name="Cromwell 1997">{{cite book|author=Cromwell, P.|year=1997|title=Polyhedra|location=United Kingdom|publisher=CUP hbk (1997), pbk. (1999), Cambridge|pages=79–86 ''Archimedean solids''|isbn=0-521-55432-2 | language=en}}</ref>。它由12個[[正五邊形]]面、20個[[正六邊形]]面、60個頂點和90個邊構成。由於包含了正五邊形和[[六邊形]]面因此也是一種[[戈德堡多面体]]，在戈德堡符號中可用GP<sub>V</sub>(1,1) 或 {5+,3}<sub>1,1</sub>表示<ref name="Wenninger 1979">{{Citation | author={{link-en|馬格努斯·J·溫尼爾|Magnus J. Wenninger|Magnus Wenninger}} |title=Spherical Models |publisher=[[Cambridge University Press]] |isbn=978-0-521-29432-4 |mr=552023 |year=1979 |url=http://www.employees.csbsju.edu/mwenninger/magnus2000.html |deadurl=yes |archiveurl=https://web.archive.org/web/20080704103400/http://www.employees.csbsju.edu/mwenninger/magnus2000.html |archivedate=2008-07-04 |language=en}} Reprinted by Dover 1999 {{isbn|978-0-486-40921-4}}</ref>。而1983年時，溫尼爾在他的書《[[溫尼爾多面體模型列表|多面體模型]]》中列出許多多面體模型，其中也收錄了此種形狀，並給予編號W<sub>9</sub><ref name="Wenninger 1974">{{cite book | author = {{link-en|馬格努斯·J·溫尼爾|Magnus J. Wenninger|Wenninger, Magnus}} | title = Polyhedron Models | publisher = Cambridge University Press | year = 1974 | isbn = 0-521-09859-9  | language=en}}</ref>。
+在[[幾何學]]中，'''截角二十面體'''是一種[[半正多面體]]，由於其具有點可遞的性質，因此屬於[[阿基米德立體]]<ref name="Cromwell 1997">{{cite book|author=Cromwell, P.|year=1997|title=Polyhedra|url=https://archive.org/details/polyhedra0000crom|location=United Kingdom|publisher=CUP hbk (1997), pbk. (1999), Cambridge|pages=[https://archive.org/details/polyhedra0000crom/page/79 79]–86 ''Archimedean solids''|isbn=0-521-55432-2 | language=en}}</ref>。它由12個[[正五邊形]]面、20個[[正六邊形]]面、60個頂點和90個邊構成。由於包含了正五邊形和[[六邊形]]面因此也是一種[[戈德堡多面体]]，在戈德堡符號中可用GP<sub>V</sub>(1,1) 或 {{mset|5+,3}}<sub>1,1</sub>表示<ref name="Wenninger 1979">{{Citation | author={{link-en|馬格努斯·J·溫尼爾|Magnus J. Wenninger|Magnus Wenninger}} |title=Spherical Models |publisher=[[Cambridge University Press]] |isbn=978-0-521-29432-4 |mr=552023 |year=1979 |url=http://www.employees.csbsju.edu/mwenninger/magnus2000.html |deadurl=yes |archiveurl=https://web.archive.org/web/20080704103400/http://www.employees.csbsju.edu/mwenninger/magnus2000.html |archivedate=2008-07-04 |language=en}} Reprinted by Dover 1999 {{isbn|978-0-486-40921-4}}</ref>。而1983年時，溫尼爾在他的書《[[溫尼爾多面體模型列表|多面體模型]]》中列出許多多面體模型，其中也收錄了此種形狀，並給予編號W<sub>9</sub><ref name="Wenninger 1974">{{cite book | author = {{link-en|馬格努斯·J·溫尼爾|Magnus J. Wenninger|Wenninger, Magnus}} | title = Polyhedron Models | publisher = Cambridge University Press | year = 1974 | isbn = 0-521-09859-9  | language=en}}</ref>。
 === 組成 ===
 {| align=right
@@ 第85行： / 第86行： @@
 === 面的組成 ===
-截角二十面體由32個面組成，在這32個面中，共包含了12個正五邊形面和20個正六邊形面，其頂角皆為三面角<ref>{{Cite web | url=https://simplydifferently.org/Geodesic_Polyhedra?page=13 | title=Geodesic Polyhedra: Geodesic Truncated Icosahedron | author=Rene K. Mueller | publisher=simply differently | date=2012-10-28 | accessdate=2019-09-11 | archive-url=https://web.archive.org/web/20141022121852/http://simplydifferently.org/Geodesic_Polyhedra?page=13 | archive-date=2014-10-22 | dead-url=no  | language=en}}</ref>，由2個六邊形和一個五邊形組成，換句話說，即每個頂點都是2個六邊形和一個五邊形的公共頂點，其[[頂點圖]]可以計為5.6.6<ref>{{Cite web | url = https://polyhedra.tessera.li/truncated-icosahedron/info | title = Truncated icosahedron, tI | publisher=polyhedra.tessera.li | accessdate=2019-09-11 | language=en}}</ref>。另外，在這個結構中，五邊形面彼此不相鄰。<ref>{{Citation|url=http://www.teachersofindia.org/en/download/file/fid/20166 |author=A. Ramachandran | title=The Truncated Icosahedron –An Iconic 3-D Shape | periodical=Azim Premji University At Right Angles | date=March 2019 |pages=69-71 | language=en}}</ref>
+截角二十面體由32個面組成，在這32個面中，共包含了12個正五邊形面和20個正六邊形面，其頂角皆為三面角<ref>{{Cite web | url=https://simplydifferently.org/Geodesic_Polyhedra?page=13 | title=Geodesic Polyhedra: Geodesic Truncated Icosahedron | author=Rene K. Mueller | publisher=simply differently | date=2012-10-28 | accessdate=2019-09-11 | archive-url=https://web.archive.org/web/20141022121852/http://simplydifferently.org/Geodesic_Polyhedra?page=13 | archive-date=2014-10-22 | dead-url=no  | language=en}}</ref>，由2個六邊形和一個五邊形組成，換句話說，即每個頂點都是2個六邊形和一個五邊形的公共頂點，其[[頂點圖]]可以計為5.6.6<ref>{{Cite web | url = https://polyhedra.tessera.li/truncated-icosahedron/info | title = Truncated icosahedron, tI | publisher = polyhedra.tessera.li | accessdate = 2019-09-11 | language = en | archive-date = 2022-03-28 | archive-url = https://web.archive.org/web/20220328043906/https://polyhedra.tessera.li/truncated-icosahedron/info }}</ref>。另外，在這個結構中，五邊形面彼此不相鄰。<ref>{{Citation |url=http://www.teachersofindia.org/en/download/file/fid/20166 |author=A. Ramachandran |title=The Truncated Icosahedron –An Iconic 3-D Shape |periodical=Azim Premji University At Right Angles |date=March 2019 |pages=69-71 |language=en |accessdate=2019-09-11 |archive-date=2021-06-22 |archive-url=https://web.archive.org/web/20210622124229/http://www.teachersofindia.org/en/download/file/fid/20166 }}</ref>
 {|
 | [[Image:Truncated icosahedron vertfig.png|160px|thumb|{{link-en|頂點佈局|Vertex_configuration}}5.6.6]]
@@ 第92行： / 第93行： @@
 === 尺寸 ===
 [[File:Truncated Platonic solid like a ball.svg|thumb|截角二十面體已知可以透過切去正二十面體的十二個頂點來構造，並且確保切出來的立體都是等邊多面體。]]
-[[File:Polyhedron truncated 20 net.svg|thumb|從截角二十面體的[[展開圖]]可以看出構成截角二十面體表面的所有形狀，只要將這些形狀的[[面積]]加總即可求得截角二十面體的[[表面積]]。]]
+[[File:Polyhedron truncated 20 net compact.svg|thumb|從截角二十面體的[[展開圖]]可以看出構成截角二十面體表面的所有形狀，只要將這些形狀的[[面積]]加總即可求得截角二十面體的[[表面積]]。]]
 若以''a''表示棱長，則截角二十面體的[[外接球]]半徑為<ref name="Harish 2014">{{citation
  |title=Mathematical analysis of truncated icosahedron & identical football
@@ 第175行： / 第176行： @@
  |periodical=Int. Conference on Adaptability in Design and Construction, Eindhoven
  |pages=3--5
- |year=2006 | language=en}}</ref><ref name="Paul Bourke 2017">{{Citation | url = http://paulbourke.net/geometry/spherical/ | title = Geometry of sports balls | chapter = § Soccer ball (Hand ball) | publisher =  Paul Bourke |date = January 2017 | author =  Paul Bourke | language=en}}</ref>
+ |year=2006 | language=en}}</ref><ref name="Paul Bourke 2017">{{Citation | url = http://paulbourke.net/geometry/spherical/ | title = Geometry of sports balls | chapter = § Soccer ball (Hand ball) | publisher = Paul Bourke | date = January 2017 | author = Paul Bourke | language = en | accessdate = 2019-09-11 | archive-date = 2018-07-27 | archive-url = https://web.archive.org/web/20180727054109/http://paulbourke.net/geometry/spherical/ | dead-url = no }}</ref>
 {|class=wikitable
 |- align=center valign=top
@@ 第190行： / 第191行： @@
 === 在科學中 ===
 [[Image:Implosion nuclear weapon design3.gif|thumb|right|截角二十面體的{{link-ja|爆縮透鏡|爆縮レンズ}}。]]
-在化學中也有一些分子的形狀為截角二十面體，如[[富勒烯]]C<sub>60</sub><ref name=Dresselhaus/><ref name=Kroto>{{cite journal |last1=Kroto |first1=H. W. |last2=Health |first2=J. R. |last3=O'Brien |first3=S. C. |last4=Curl |first4=R. F. |last5=Smalley |first5=R. E. |title=C<sub>60</sub>: Buckminsterfullerene |journal=Nature |year=1985 |volume=318 |pages=162–163 |doi=10.1038/318162a0 |bibcode=1985Natur.318..162K |issue=6042 | language=en}}</ref>，這種分子於1985年被發現，其直徑約為0.71奈米<ref>{{cite book|title=Nanostructured materials for solar energy conversion|editor=Sōga, Tetsuo |chapter=Fullerene Thin Films as Photovoltaic Material|author=Katz, E. A.|year=2006 |isbn=978-0-444-52844-5|publisher=Elsevier|pages=364|chapter-url=https://books.google.com/books?id=GmQR1tuk5IgC&pg=PA361|ref=Katz | language=en}}</ref>，由60個碳原子組成，且每個碳原子正好位於截角二十面體的頂點上<ref name="article ozaki1986electronic">{{Cite journal
+在化學中也有一些分子的形狀為截角二十面體，如[[富勒烯]]C<sub>60</sub><ref name=Dresselhaus/><ref name=Kroto>{{cite journal |last1=Kroto |first1=H. W. |last2=Health |first2=J. R. |last3=O'Brien |first3=S. C. |last4=Curl |first4=R. F. |last5=Smalley |first5=R. E. |title=C<sub>60</sub>: Buckminsterfullerene |journal=Nature |year=1985 |volume=318 |pages=162–163 |doi=10.1038/318162a0 |bibcode=1985Natur.318..162K |issue=6042 | language=en}}</ref>，這種分子於1985年被發現，其直徑約為0.71奈米<ref>{{cite book|title=Nanostructured materials for solar energy conversion|editor=Sōga, Tetsuo|chapter=Fullerene Thin Films as Photovoltaic Material|author=Katz, E. A.|year=2006|isbn=978-0-444-52844-5|publisher=Elsevier|pages=364|chapter-url=https://books.google.com/books?id=GmQR1tuk5IgC&pg=PA361|ref=Katz|language=en|access-date=2019-09-10|archive-date=2021-03-18|archive-url=https://web.archive.org/web/20210318034815/https://books.google.com/books?id=GmQR1tuk5IgC&pg=PA361|dead-url=no}}</ref>，由60個碳原子組成，且每個碳原子正好位於截角二十面體的頂點上<ref name="article ozaki1986electronic">{{Cite journal
   |title=On electronic states and bond lengths of the truncated icosahedral C<sub>60</sub> molecule
   |author=Ozaki, Masa-aki and Taahashi, Akira
@@ 第198行： / 第199行： @@
   |pages=242--244
   |year=1986
-  |publisher=Elsevier | language=en}}</ref>。這種形狀也用於核子武器中，聚焦雷管的爆炸衝擊波用的{{link-ja|爆縮透鏡|爆縮レンズ}}，並配置於[[三位一體_(核試驗)|小工具]]和[[胖子原子彈|胖子]]等[[原子彈]]中<ref>{{cite book
+  |publisher=Elsevier | language=en}}</ref>。這種形狀也用於核子武器中，聚焦雷管的爆炸衝擊波用的{{link-ja|爆縮透鏡|爆縮レンズ}}，並配置於[[三位一體_(核試驗)|三位一體]]和[[胖子原子彈|胖子]]等[[原子彈]]中<ref>{{cite book
 |author=Rhodes, Richard
 |title=Dark Sun: The Making of the Hydrogen Bomb
@@ 第321行： / 第322行： @@
 ![[File:Nonuniform truncated icosahedron.png|64px]]<br/>非半正截角二十面體<br/>2 5 | 3
 |[[Image:Great truncated dodecahedron.png|64px]]<br/>[[截角大十二面體|U37]]<br/> 2 {{sfrac|5|2}} | 5
-|[[Image:Great dodecicosidodecahedron.png|64px]]<br/>{{link-en|大十二面二十面十二面體|Great dodecicosidodecahedron|U61}}<br> {{sfrac|5|2}} 3 | {{sfrac|5|3}}
+|[[Image:Great dodecicosidodecahedron.png|64px]]<br/>[[大十二面截半二十面體|U61]]<br> {{sfrac|5|2}} 3 | {{sfrac|5|3}}
 |[[Image:Uniform great rhombicosidodecahedron.png|64px]]<br/>{{link-en|均勻星形斜方截半二十面體|Nonconvex great rhombicosidodecahedron|U67}}<br> {{sfrac|5|3}} 3 | 2
-|[[Image:Great rhombidodecahedron.png|64px]]<br/>{{link-en|大斜方十二面體|Great rhombidodecahedron|U73}}<br/>2 {{sfrac|5|3}} ({{sfrac|3|2}} {{sfrac|5|4}})
+|[[Image:Great rhombidodecahedron.png|64px]]<br/>[[大斜方十二面體|U73]]<br/>2 {{sfrac|5|3}} ({{sfrac|3|2}} {{sfrac|5|4}})
 |[[File:Complete icosahedron ortho stella.png|64px]]<br/>[[完全星形二十面體]]
 |-
 ![[File:Rhombidodecadodecahedron convex hull.png|64px]]<br/>非半正截角二十面體<br/>2 5 | 3
-|[[Image:Rhombidodecadodecahedron.png|64px]]<br/>{{link-en|斜方截半大十二面體|Rhombidodecadodecahedron|U38}}<br/>{{sfrac|5|2}} 5 | 2
+|[[Image:Rhombidodecadodecahedron.png|64px]]<br/>[[斜方截半大十二面體|U38]]<br/>{{sfrac|5|2}} 5 | 2
 |[[Image:Icosidodecadodecahedron.png|64px]]<br/>{{link-en|二十面十二面十二面體|Icosidodecadodecahedron|U44}}<br/>{{sfrac|5|3}} 5 | 3
-|[[Image:Rhombicosahedron.png|64px]]<br/>{{link-en|斜方二十面体|Rhombicosahedron|U56}}<br/>2 3 (5/4 {{sfrac|5|2}}) |
+|[[Image:Rhombicosahedron.png|64px]]<br/>[[斜方二十面体|U56]]<br/>2 3 (5/4 {{sfrac|5|2}}) |
 |-
 ![[File:Small snub icosicosidodecahedron convex hull.png|64px]]<br/>非半正截角二十面體<br>2 5 | 3
-|[[Image:Small snub icosicosidodecahedron.png|64px]]<br/>{{link-en|小扭稜二十面二十面十二面體|Small snub icosicosidodecahedron|U32}}<br/> | {{sfrac|5|2}} 3 3
+|[[Image:Small snub icosicosidodecahedron.png|64px]]<br/>[[完全扭稜二十面體|U32]]<br/> | {{sfrac|5|2}} 3 3
 |}
 |}
@@ 第364行： / 第365行： @@
  | properties = {{link-en|立方體圖|Cubic graph|立方體}}、[[哈密顿图|哈密顿]]、[[正則圖|正則]]、{{link-en|零對稱性圖|Zero-symmetric graph|零對稱性}}
 }}
-在圖論的數學領域中，截角二十面體圖是[[阿基米得立體]]中截角二十面體之{{link-en|n-骨架|n-skeleton|邊與頂點的圖}}<ref name="article kostant1995graph">{{Cite journal  |title=''"The graph of the truncated icosahedron and the last letter of Galois"''  |author=Kostant, Bertram  |journal=Notices of the AMS  |volume=42  |url=http://www.ams.org/notices/199509/kostant.pdf  |number=9  |pages=959--968  |year=1995  |access-date=2018-02-01  |archive-url=https://web.archive.org/web/20170115165248/http://www.ams.org/notices/199509/kostant.pdf  |archive-date=2017-01-15  |dead-url=no  | language=en }}</ref>，其有時被稱為'''巴克明斯特富勒圖'''<ref name="Chris Godsil 2001">{{Cite book | author= Chris Godsil, Gordon F. Royle | title= "Algebraic Graph Theory"|isbn=978-0387952208 |series=Graduate Texts in Mathematics|location=New York|publisher=Springer-Verlag|page=211|year=2001 | language=en}} </ref>。共有60個頂點和90條稜，且是{{link-en|立方體圖|Cubic graph|立方體}}的{{link-en|阿基米德圖|Archimedean graph}}<ref>{{citation|last1=Read|first1=R. C.|last2=Wilson|first2=R. J.|title=An Atlas of Graphs|publisher=[[Oxford University Press]]|year= 1998|page=268 | language=en}}</ref><ref name="TruncatedIcosahedralGraph,Mathworld">{{Cite web | url = http://mathworld.wolfram.com/TruncatedIcosahedralGraph.html | title = TruncatedIcosahedralGraph | author = [[埃里克·韦斯坦因|Weisstein, Eric W]] | publisher = [[MathWorld]]--A Wolfram Web Resource. | access-date = 2019-09-06 | archive-url = https://web.archive.org/web/20190518211933/http://mathworld.wolfram.com/TruncatedIcosahedralGraph.html | archive-date = 2019-05-18 | dead-url = no  | language=en}}</ref><ref name="Chris Godsil 2001"/><ref name="article kostant1995graph"/>。
+在圖論的數學領域中，截角二十面體圖是[[阿基米得立體]]中截角二十面體之{{link-en|n-骨架|n-skeleton|邊與頂點的圖}}<ref name="article kostant1995graph">{{Cite journal  |title=''"The graph of the truncated icosahedron and the last letter of Galois"''  |author=Kostant, Bertram  |journal=Notices of the AMS  |volume=42  |url=http://www.ams.org/notices/199509/kostant.pdf  |number=9  |pages=959--968  |year=1995  |access-date=2018-02-01  |archive-url=https://web.archive.org/web/20170115165248/http://www.ams.org/notices/199509/kostant.pdf  |archive-date=2017-01-15  |dead-url=no  | language=en }}</ref>，其有時被稱為'''巴克明斯特富勒圖'''<ref name="Chris Godsil 2001">{{Cite book | author= Chris Godsil, Gordon F. Royle | title= "Algebraic Graph Theory"| url= https://archive.org/details/algebraicgraphth00gods |isbn=978-0387952208 |series=Graduate Texts in Mathematics|location=New York|publisher=Springer-Verlag|page=[https://archive.org/details/algebraicgraphth00gods/page/n231 211]|year=2001 | language=en}} </ref>。共有60個頂點和90條稜，且是{{link-en|立方體圖|Cubic graph|立方體}}的{{link-en|阿基米德圖|Archimedean graph}}<ref>{{citation|last1=Read|first1=R. C.|last2=Wilson|first2=R. J.|title=An Atlas of Graphs|publisher=[[Oxford University Press]]|year= 1998|page=268 | language=en}}</ref><ref name="TruncatedIcosahedralGraph,Mathworld">{{Cite web | url = http://mathworld.wolfram.com/TruncatedIcosahedralGraph.html | title = TruncatedIcosahedralGraph | author = [[埃里克·韦斯坦因|Weisstein, Eric W]] | publisher = [[MathWorld]]--A Wolfram Web Resource. | access-date = 2019-09-06 | archive-url = https://web.archive.org/web/20190518211933/http://mathworld.wolfram.com/TruncatedIcosahedralGraph.html | archive-date = 2019-05-18 | dead-url = no  | language=en}}</ref><ref name="Chris Godsil 2001"/><ref name="article kostant1995graph"/>。
 {| class=wikitable
 |+ 正射投影
@@ 第382行： / 第383行： @@
 {{refbegin|2}}
 # {{The Geometrical Foundation of Natural Structure (book)}} (Section 3-9)
-# {{cite book|author=Cromwell, P.|year=1997|title=Polyhedra|location=United Kingdom|publisher=Cambridge|pages=79–86 ''Archimedean solids''|isbn=0-521-55432-2}}
+# {{cite book|author=Cromwell, P.|year=1997|title=Polyhedra|url=https://archive.org/details/polyhedra0000crom|location=United Kingdom|publisher=Cambridge|pages=[https://archive.org/details/polyhedra0000crom/page/79 79]–86 ''Archimedean solids''|isbn=0-521-55432-2}}
 {{refend}}
@@ 第389行： / 第390行： @@
 ** {{mathworld |urlname=TruncatedIcosahedralGraph |title=Truncated icosahedral graph}}
 * {{KlitzingPolytopes|polyhedra.htm|3D convex uniform polyhedra|x3x5o - ti}}
-* [http://www.dr-mikes-math-games-for-kids.com/polyhedral-nets.html?net=FcWqcgCFwJy8hdr7Tpr0E5bZt5x3cWEH6dwwKHCmiAYTfaZwqWF0uEVMInwLYVXfN8PDwpUyauaLHhwJXg40Gas6xdXAY3rsGHjcD6k2QDzGzKkuLMI31aQ26rI3uD7eErma5wI9FxPhfb5HKttbwy3zq25bAOIRYmGDfrCf7sgmOwZShNBxNitreNFUEgEVyUztA9NGYxLLw6iOI3DGr1wAFohIvfYJ9v6CVDw7VE0C0NFaLQlslQ6L5acUJqQrPut6CxdTdxW1jRbZLXocrlCgQRpfanYeiaOBkYeHTpTQ1A5A8vkLNMrMDBl02ot2TMhJrhkI5ignSVOJ5X4S7GyUQMhdnriBZb447C6DLmqsGccUdj3C87AInlwfzYEQZvAXy0RR5QIt7WdIlLlcQldm&name=Truncated+Icosahedron#applet Editable printable net of a truncated icosahedron with interactive 3D view]
+* [http://www.dr-mikes-math-games-for-kids.com/polyhedral-nets.html?net=FcWqcgCFwJy8hdr7Tpr0E5bZt5x3cWEH6dwwKHCmiAYTfaZwqWF0uEVMInwLYVXfN8PDwpUyauaLHhwJXg40Gas6xdXAY3rsGHjcD6k2QDzGzKkuLMI31aQ26rI3uD7eErma5wI9FxPhfb5HKttbwy3zq25bAOIRYmGDfrCf7sgmOwZShNBxNitreNFUEgEVyUztA9NGYxLLw6iOI3DGr1wAFohIvfYJ9v6CVDw7VE0C0NFaLQlslQ6L5acUJqQrPut6CxdTdxW1jRbZLXocrlCgQRpfanYeiaOBkYeHTpTQ1A5A8vkLNMrMDBl02ot2TMhJrhkI5ignSVOJ5X4S7GyUQMhdnriBZb447C6DLmqsGccUdj3C87AInlwfzYEQZvAXy0RR5QIt7WdIlLlcQldm&name=Truncated+Icosahedron#applet Editable printable net of a truncated icosahedron with interactive 3D view] {{Wayback|url=http://www.dr-mikes-math-games-for-kids.com/polyhedral-nets.html?net=FcWqcgCFwJy8hdr7Tpr0E5bZt5x3cWEH6dwwKHCmiAYTfaZwqWF0uEVMInwLYVXfN8PDwpUyauaLHhwJXg40Gas6xdXAY3rsGHjcD6k2QDzGzKkuLMI31aQ26rI3uD7eErma5wI9FxPhfb5HKttbwy3zq25bAOIRYmGDfrCf7sgmOwZShNBxNitreNFUEgEVyUztA9NGYxLLw6iOI3DGr1wAFohIvfYJ9v6CVDw7VE0C0NFaLQlslQ6L5acUJqQrPut6CxdTdxW1jRbZLXocrlCgQRpfanYeiaOBkYeHTpTQ1A5A8vkLNMrMDBl02ot2TMhJrhkI5ignSVOJ5X4S7GyUQMhdnriBZb447C6DLmqsGccUdj3C87AInlwfzYEQZvAXy0RR5QIt7WdIlLlcQldm&name=Truncated+Icosahedron#applet |date=20210318033549 }}
-* [http://www.mathconsult.ch/showroom/unipoly/ The Uniform Polyhedra]
+* [http://www.mathconsult.ch/showroom/unipoly/ The Uniform Polyhedra] {{Wayback|url=http://www.mathconsult.ch/showroom/unipoly/ |date=20080211233246 }}
-* [http://www.georgehart.com/virtual-polyhedra/vp.html Virtual Reality Polyhedra] The Encyclopedia of Polyhedra
+* [http://www.georgehart.com/virtual-polyhedra/vp.html Virtual Reality Polyhedra] {{Wayback|url=http://www.georgehart.com/virtual-polyhedra/vp.html |date=20080223132026 }} The Encyclopedia of Polyhedra
-* [http://visualoop.com/blog/22881/3d-world-cup-dataviz-ball-by-times-of-oman 3D paper data visualization World Cup ball]
+* [http://visualoop.com/blog/22881/3d-world-cup-dataviz-ball-by-times-of-oman 3D paper data visualization World Cup ball] {{Wayback|url=http://visualoop.com/blog/22881/3d-world-cup-dataviz-ball-by-times-of-oman |date=20210318033609 }}
 {{阿基米德立體}}
 {{康威多面體}}
-[[Category:多面體]]
 [[Category:半正多面體]]
 [[category:康威多面體]]
 [[Category:阿基米德立體]]
+[[Category:均勻多面體]]

對稱群: [5,3]（英语：Icosahedral symmetry）, (*532)							[5,3]⁺, (532)


{5,3}	t_0,1{5,3}	t₁{5,3}	t_0,1{3,5}	{3,5}	t_0,2{5,3}	t_0,1,2{5,3}	s{5,3}
半正多面体对偶


V5.5.5	V3.10.10	V3.5.3.5	V5.6.6	V3.3.3.3.3	V3.4.5.4	V4.6.10	V3.3.3.3.5

截角鑲嵌對稱性 *n32 的變種: n.6.6
Sym. *n42 [n,3]	球面鑲嵌（英语：List of spherical symmetry groups）				歐氏鑲嵌（英语：List_of_planar_symmetry_groups）	緊湊雙曲		仿緊雙曲	非緊雙曲
Sym. *n42 [n,3]	*232 [2,3]	*332 [3,3]	*432 [4,3]	*532 [5,3]	*632 [6,3]	*732 [7,3]	*832 [8,3]...	*∞32 [∞,3]	[12i,3]	[9i,3]	[6i,3]
截角鑲嵌圖
頂點（英语：Vertex configuration）	2.6.6	3.6.6	4.6.6	5.6.6	6.6.6	7.6.6	8.6.6（英语：Truncated order-8 triangular tiling）	∞.6.6（英语：Truncated infinite-order triangular tiling）	12i.6.6	9i.6.6	6i.6.6
n-kis鑲嵌圖
頂點（英语：Vertex configuration）	V2.6.6	V3.6.6	V4.6.6	V5.6.6	V6.6.6	V7.6.6	V8.6.6	V∞.6.6	V12i.6.6	V9i.6.6	V6i.6.6

查论编康威多面體
四面體變體	六角化截角四面體截角三角化四面體六角化五角化截角三角化四面體
六面體變體	四角化扭棱立方體四角化截半立方體
八面體變體	截角菱形十二面體
十二面體變體	五角化十二面體五角化截半二十面體五角六十面體五角化扭棱十二面體截角五角六十面體
二十面體變體	截半截角二十面體菱形九十面體截角五角六十面體截角菱形三十面體六角化五角化倒角十二面體

大斜方六百胞體	過截角一百二十胞體
（展開圖）	（展開圖）