第305行:
第305行:
: <math>\cos\frac{\pi}{4}=\cos 45^\circ=\frac{\sqrt2}{2}=\frac{1}{\sqrt2}\,</math>
: <math>\cos\frac{\pi}{4}=\cos 45^\circ=\frac{\sqrt2}{2}=\frac{1}{\sqrt2}\,</math>
: <math>\tan\frac{\pi}{4}=\tan 45^\circ=1</math>
: <math>\tan\frac{\pi}{4}=\tan 45^\circ=1</math>
===54°:27°与27°的和===
:<math>\sin\frac{3\pi}{10}=\sin 54^\circ=\frac{\sqrt5+1}{4}\,\!</math>
:<math>\cos\frac{3\pi}{10}=\cos 54^\circ=\frac{\sqrt{10-2\sqrt{5}}}{4}</math>
:<math>\tan\frac{3\pi}{10}=\tan 54^\circ=\frac{\sqrt{25+10\sqrt{5}}}{5}\,</math>
:<math>\cot\frac{3\pi}{10}=\cot 54^\circ=\sqrt{5-\sqrt{20}}\,</math>
===60°:等边三角形===
:<math>\sin\frac{\pi}{3}=\sin 60^\circ=\frac{\sqrt3}{2}\,</math>
:<math>\cos\frac{\pi}{3}=\cos 60^\circ=\frac{1}{2}\,</math>
:<math>\tan\frac{\pi}{3}=\tan 60^\circ=\sqrt3\,</math>
:<math>\cot\frac{\pi}{3}=\cot 60^\circ=\frac{\sqrt3}{3}=\frac{1}{\sqrt3}\,</math>
===67.5°:7.5°与60°的和===
:<math>\sin\frac{3\pi}{8}=\sin 67.5^\circ=\tfrac{1}{2}\sqrt{2+\sqrt{2}}\,</math>
:<math>\cos\frac{3\pi}{8}=\cos 67.5^\circ=\tfrac{1}{2}\sqrt{2-\sqrt{2}}\,</math>
:<math>\tan\frac{3\pi}{8}=\tan 67.5^\circ=\sqrt{2}+1\,</math>
:<math>\cot\frac{3\pi}{8}=\cot 67.5^\circ=\sqrt{2}-1\,</math>
===72°:36°与36°的和===
:<math>\sin\frac{2\pi}{5}=\sin 72^\circ=\tfrac{1}{4}\sqrt{2\left(5+\sqrt5\right)}\,</math>
:<math>\cos\frac{2\pi}{5}=\cos 72^\circ=\tfrac{1}{4}\left(\sqrt5-1\right)\,</math>
:<math>\tan\frac{2\pi}{5}=\tan 72^\circ=\sqrt{5+2\sqrt 5}\,</math>
:<math>\cot\frac{2\pi}{5}=\cot 72^\circ=\tfrac{1}{5}\sqrt{5\left(5-2\sqrt5\right)}\,</math>
=== 75°: 30°与45°的和 ===
:<math>\sin\frac{5\pi}{12}=\sin 75^\circ=\tfrac{1}{4}\left(\sqrt6+\sqrt2\right)\,</math>
:<math>\cos\frac{5\pi}{12}=\cos 75^\circ=\tfrac{1}{4}\left(\sqrt6-\sqrt2\right)\,</math>
:<math>\tan\frac{5\pi}{12}=\tan 75^\circ=2+\sqrt3\,</math>
:<math>\cot\frac{5\pi}{12}=\cot 75^\circ=2-\sqrt3\,</math>
=== 90°:根本 ===
:<math>\sin \frac{\pi}{2}=\sin 90^\circ=1\,</math>
:<math>\cos \frac{\pi}{2}=\cos 90^\circ=0\,</math>
:<math>\cot \frac{\pi}{2}=\cot 90^\circ=0\,</math>
==列表==
==列表==
三角函數精確值 是利用三角函數的公式 將特定的三角函數 值加以化簡,並以數學根式 或分數 表示
用根式 或分數 表達的精確三角函數 有時很有用,主要用於簡化的解決某些方程式 能進一步化簡。
根据尼云定理 ,有理数度数的角的正弦值,其中的有理数仅有0,
±
1
2
{\displaystyle \pm {\frac {1}{2}}}
相同角度的轉換表
角度單位
值
轉
0
{\displaystyle 0}
1
12
{\displaystyle {\frac {1}{12}}}
1
8
{\displaystyle {\frac {1}{8}}}
1
6
{\displaystyle {\frac {1}{6}}}
1
4
{\displaystyle {\frac {1}{4}}}
1
2
{\displaystyle {\frac {1}{2}}}
3
4
{\displaystyle {\frac {3}{4}}}
1
{\displaystyle 1}
角度
0
∘
{\displaystyle 0^{\circ }}
30
∘
{\displaystyle 30^{\circ }}
45
∘
{\displaystyle 45^{\circ }}
60
∘
{\displaystyle 60^{\circ }}
90
∘
{\displaystyle 90^{\circ }}
180
∘
{\displaystyle 180^{\circ }}
270
∘
{\displaystyle 270^{\circ }}
360
∘
{\displaystyle 360^{\circ }}
弧度
0
{\displaystyle 0}
π
6
{\displaystyle {\frac {\pi }{6}}}
π
4
{\displaystyle {\frac {\pi }{4}}}
π
3
{\displaystyle {\frac {\pi }{3}}}
π
2
{\displaystyle {\frac {\pi }{2}}}
π
{\displaystyle \pi }
3
π
2
{\displaystyle {\frac {3\pi }{2}}}
2
π
{\displaystyle 2\pi }
梯度
0
g
{\displaystyle 0^{g}}
33
1
3
g
{\displaystyle 33{\frac {1}{3}}^{g}}
50
g
{\displaystyle 50^{g}}
66
2
3
g
{\displaystyle 66{\frac {2}{3}}^{g}}
100
g
{\displaystyle 100^{g}}
200
g
{\displaystyle 200^{g}}
300
g
{\displaystyle 300^{g}}
400
g
{\displaystyle 400^{g}}
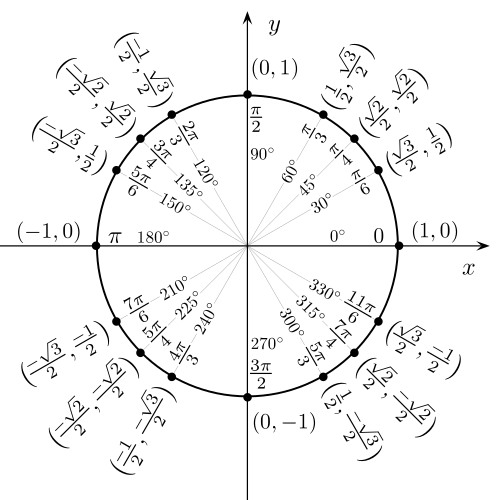
例如:0°、30°、45°
單位圓
例如:15°、22.5°
sin
(
x
2
)
=
±
1
2
(
1
−
cos
x
)
{\displaystyle \sin \left({\frac {x}{2}}\right)=\pm \,{\sqrt {{\tfrac {1}{2}}(1-\cos x)}}}
cos
(
x
2
)
=
±
1
2
(
1
+
cos
x
)
{\displaystyle \cos \left({\frac {x}{2}}\right)=\pm \,{\sqrt {{\tfrac {1}{2}}(1+\cos x)}}}
1
3
{\displaystyle {\frac {1}{3}}\,}
例如:10°、20°、7°......等等,非三的倍數的角的精確值。
sin
3
θ
=
3
sin
θ
−
4
sin
3
θ
{\displaystyle \sin 3\theta =3\sin \theta -4\sin ^{3}\theta \,}
cos
3
θ
=
4
cos
3
θ
−
3
cos
θ
{\displaystyle \cos 3\theta =4\cos ^{3}\theta -3\cos \theta \,}
把它改為
sin
θ
=
3
sin
1
3
θ
−
4
sin
3
1
3
θ
{\displaystyle \sin \theta =3\sin {\frac {1}{3}}\theta -4\sin ^{3}{\frac {1}{3}}\theta \,}
cos
θ
=
4
cos
3
1
3
θ
−
3
cos
1
3
θ
{\displaystyle \cos \theta =4\cos ^{3}{\frac {1}{3}}\theta -3\cos {\frac {1}{3}}\theta \,}
把
cos
1
3
θ
{\displaystyle \cos {\frac {1}{3}}\theta \,}
cos
θ
{\displaystyle \cos \theta \,}
一元三次方程式 即可求出
例如:
sin
π
9
=
sin
20
∘
=
−
3
16
+
−
1
256
3
+
−
3
16
−
−
1
256
3
{\displaystyle \sin {\frac {\pi }{9}}=\sin 20^{\circ }={\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}+{\sqrt {-{\frac {1}{256}}}}}}+{\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}-{\sqrt {-{\frac {1}{256}}}}}}}
同樣地,若角度代未知數,則會得到三分之一角公式 。
cos
θ
n
=
ℜ
(
cos
θ
+
i
sin
θ
n
)
=
1
2
(
cos
θ
+
i
sin
θ
n
+
cos
θ
−
i
sin
θ
n
)
{\displaystyle \cos {\frac {\theta }{n}}=\Re \left({\sqrt[{n}]{\cos \theta +i\sin \theta }}\right)={\frac {1}{2}}\left({\sqrt[{n}]{\cos \theta +i\sin \theta }}+{\sqrt[{n}]{\cos \theta -i\sin \theta }}\right)}
sin
θ
n
=
ℑ
(
cos
θ
+
i
sin
θ
n
)
=
1
2
i
(
cos
θ
+
i
sin
θ
n
−
cos
θ
−
i
sin
θ
n
)
{\displaystyle \sin {\frac {\theta }{n}}=\Im \left({\sqrt[{n}]{\cos \theta +i\sin \theta }}\right)={\frac {1}{2i}}\left({\sqrt[{n}]{\cos \theta +i\sin \theta }}-{\sqrt[{n}]{\cos \theta -i\sin \theta }}\right)}
例如:
sin
1
∘
=
1
2
i
(
cos
3
∘
+
i
sin
3
∘
3
−
cos
3
∘
−
i
sin
3
∘
3
)
{\displaystyle \sin {1^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {3^{\circ }}+i\sin {3^{\circ }}}}-{\sqrt[{3}]{\cos {3^{\circ }}-i\sin {3^{\circ }}}}\right)}
=
1
4
2
3
i
{
[
2
(
1
+
3
)
5
+
5
+
2
(
5
−
1
)
(
3
−
1
)
]
+
i
[
2
(
1
−
3
)
5
+
5
+
2
(
5
−
1
)
(
3
+
1
)
]
3
{\displaystyle ={\frac {1}{4{\sqrt[{3}]{2}}i}}{\Bigg \{}{\sqrt[{3}]{\left[2(1+{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}-1)\right]+i\left[2(1-{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}+1)\right]}}}
−
[
2
(
1
+
3
)
5
+
5
+
2
(
5
−
1
)
(
3
−
1
)
]
−
i
[
2
(
1
−
3
)
5
+
5
+
2
(
5
−
1
)
(
3
+
1
)
]
3
}
{\displaystyle -{\sqrt[{3}]{\left[2(1+{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}-1)\right]-i\left[2(1-{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}+1)\right]}}{\Bigg \}}}
[ 1]
例如:21° = 9° + 12°
sin
(
x
±
y
)
=
sin
(
x
)
cos
(
y
)
±
cos
(
x
)
sin
(
y
)
{\displaystyle \sin(x\pm y)=\sin(x)\cos(y)\pm \cos(x)\sin(y)\,}
cos
(
x
±
y
)
=
cos
(
x
)
cos
(
y
)
∓
sin
(
x
)
sin
(
y
)
{\displaystyle \cos(x\pm y)=\cos(x)\cos(y)\mp \sin(x)\sin(y)\,}
Chord(36°) = a/b = 1/φ, 根据托勒密定理 例如:18°
根據托勒密定理,在圓內接四邊形 ABCD中,
a
2
+
a
b
=
b
2
{\displaystyle a^{2}+ab=b^{2}}
(
a
b
)
2
+
a
b
=
1
{\displaystyle \left({\frac {a}{b}}\right)^{2}+{\frac {a}{b}}=1}
c
r
d
36
∘
=
c
r
d
(
∠
A
D
B
)
=
a
b
=
5
−
1
2
{\displaystyle \mathrm {crd} \ {36^{\circ }}=\mathrm {crd} \left(\angle \mathrm {ADB} \right)={\frac {a}{b}}={\frac {{\sqrt {5}}-1}{2}}}
c
r
d
θ
=
2
sin
θ
2
{\displaystyle \mathrm {crd} \ {\theta }=2\sin {\frac {\theta }{2}}\,}
sin
18
∘
=
5
−
1
4
{\displaystyle \sin {18^{\circ }}={\frac {{\sqrt {5}}-1}{4}}}
由于三角函数的特性,大于45°角度的三角函数值,可以经由自0°~45°的角度的三角函数值的相关的计算取得。
sin
0
=
0
{\displaystyle \sin 0=0\,}
cos
0
=
1
{\displaystyle \cos 0=1\,}
tan
0
=
0
{\displaystyle \tan 0=0\,}
sin
1
∘
=
1
+
3
i
16
4
30
−
8
15
+
3
5
+
8
5
+
5
+
4
10
−
4
6
−
4
2
+
(
4
30
+
8
15
+
3
5
+
8
5
+
5
−
4
10
−
4
6
+
4
2
)
i
3
+
{\displaystyle \sin {1^{\circ }}={\frac {1+{\sqrt {3}}i}{16}}{\sqrt[{3}]{4{\sqrt {30}}-8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}+4{\sqrt {10}}-4{\sqrt {6}}-4{\sqrt {2}}+\left(4{\sqrt {30}}+8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}-4{\sqrt {10}}-4{\sqrt {6}}+4{\sqrt {2}}\right)i}}+}
1
−
3
i
16
4
30
−
8
15
+
3
5
+
8
5
+
5
+
4
10
−
4
6
−
4
2
−
(
4
30
+
8
15
+
3
5
+
8
5
+
5
−
4
10
−
4
6
+
4
2
)
i
3
{\displaystyle {\frac {1-{\sqrt {3}}i}{16}}{\sqrt[{3}]{4{\sqrt {30}}-8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}+4{\sqrt {10}}-4{\sqrt {6}}-4{\sqrt {2}}-\left(4{\sqrt {30}}+8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}-4{\sqrt {10}}-4{\sqrt {6}}+4{\sqrt {2}}\right)i}}}
[ 2]
sin
(
π
120
)
=
sin
(
1.5
∘
)
=
(
2
+
2
)
(
15
+
3
−
10
−
2
5
)
−
(
2
−
2
)
(
30
−
6
5
+
5
+
1
)
16
{\displaystyle \sin \left({\frac {\pi }{120}}\right)=\sin \left(1.5^{\circ }\right)={\frac {\left({\sqrt {2+{\sqrt {2}}}}\right)\left({\sqrt {15}}+{\sqrt {3}}-{\sqrt {10-2{\sqrt {5}}}}\right)-\left({\sqrt {2-{\sqrt {2}}}}\right)\left({\sqrt {30-6{\sqrt {5}}}}+{\sqrt {5}}+1\right)}{16}}}
cos
(
π
120
)
=
cos
(
1.5
∘
)
=
(
2
+
2
)
(
30
−
6
5
+
5
+
1
)
+
(
2
−
2
)
(
15
+
3
−
10
−
2
5
)
16
{\displaystyle \cos \left({\frac {\pi }{120}}\right)=\cos \left(1.5^{\circ }\right)={\frac {\left({\sqrt {2+{\sqrt {2}}}}\right)\left({\sqrt {30-6{\sqrt {5}}}}+{\sqrt {5}}+1\right)+\left({\sqrt {2-{\sqrt {2}}}}\right)\left({\sqrt {15}}+{\sqrt {3}}-{\sqrt {10-2{\sqrt {5}}}}\right)}{16}}}
sin
(
π
96
)
=
sin
(
1.875
∘
)
=
1
2
2
−
2
+
2
+
2
+
3
{\displaystyle \sin \left({\frac {\pi }{96}}\right)=\sin \left(1.875^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {3}}}}}}}}}}}
cos
(
π
96
)
=
cos
(
1.875
∘
)
=
1
2
2
+
2
+
2
+
2
+
3
{\displaystyle \cos \left({\frac {\pi }{96}}\right)=\cos \left(1.875^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {3}}}}}}}}}}}
sin
2
∘
=
1
2
i
(
cos
6
∘
+
i
sin
6
∘
3
−
cos
6
∘
−
i
sin
6
∘
3
)
{\displaystyle \sin {2^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {6^{\circ }}+i\sin {6^{\circ }}}}-{\sqrt[{3}]{\cos {6^{\circ }}-i\sin {6^{\circ }}}}\right)}
=
1
4
i
{
[
2
(
5
−
5
)
+
3
(
5
+
1
)
]
+
i
[
6
(
5
−
5
)
−
5
−
1
]
3
{\displaystyle ={\frac {1}{4i}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]+i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}}
−
[
2
(
5
−
5
)
+
3
(
5
+
1
)
]
−
i
[
6
(
5
−
5
)
−
5
−
1
]
3
}
{\displaystyle -{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]-i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}{\Bigg \}}}
cos
2
∘
=
1
2
(
cos
6
∘
+
i
sin
6
∘
3
+
cos
6
∘
−
i
sin
6
∘
3
)
{\displaystyle \cos {2^{\circ }}={\frac {1}{2}}\left({\sqrt[{3}]{\cos {6^{\circ }}+i\sin {6^{\circ }}}}+{\sqrt[{3}]{\cos {6^{\circ }}-i\sin {6^{\circ }}}}\right)}
=
1
4
{
[
2
(
5
−
5
)
+
3
(
5
+
1
)
]
+
i
[
6
(
5
−
5
)
−
5
−
1
]
3
{\displaystyle ={\frac {1}{4}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]+i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}}
+
[
2
(
5
−
5
)
+
3
(
5
+
1
)
]
−
i
[
6
(
5
−
5
)
−
5
−
1
]
3
}
{\displaystyle +{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]-i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}{\Bigg \}}}
sin
(
π
80
)
=
sin
(
2.25
∘
)
=
1
2
2
−
2
+
2
+
5
+
5
2
{\displaystyle \sin \left({\frac {\pi }{80}}\right)=\sin \left(2.25^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {\frac {5+{\sqrt {5}}}{2}}}}}}}}}}
cos
(
π
80
)
=
cos
(
2.25
∘
)
=
1
2
2
+
2
+
2
+
5
+
5
2
{\displaystyle \cos \left({\frac {\pi }{80}}\right)=\cos \left(2.25^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {\frac {5+{\sqrt {5}}}{2}}}}}}}}}}
sin
(
π
64
)
=
sin
(
2.8125
∘
)
=
1
2
2
−
2
+
2
+
2
+
2
{\displaystyle \sin \left({\frac {\pi }{64}}\right)=\sin \left(2.8125^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}}}}}
cos
(
π
64
)
=
cos
(
2.8125
∘
)
=
1
2
2
+
2
+
2
+
2
+
2
{\displaystyle \cos \left({\frac {\pi }{64}}\right)=\cos \left(2.8125^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}}}}}
sin
π
60
=
sin
3
∘
=
1
4
8
−
3
−
15
−
10
−
2
5
{\displaystyle \sin {\frac {\pi }{60}}=\sin 3^{\circ }={\tfrac {1}{4}}{\sqrt {8-{\sqrt {3}}-{\sqrt {15}}-{\sqrt {10-2{\sqrt {5}}}}}}\,}
cos
π
60
=
cos
3
∘
=
1
4
8
+
3
+
15
+
10
−
2
5
{\displaystyle \cos {\frac {\pi }{60}}=\cos 3^{\circ }={\tfrac {1}{4}}{\sqrt {8+{\sqrt {3}}+{\sqrt {15}}+{\sqrt {10-2{\sqrt {5}}}}}}\,}
tan
π
60
=
tan
3
∘
=
1
4
[
(
2
−
3
)
(
3
+
5
)
−
2
]
[
2
−
2
(
5
−
5
)
]
{\displaystyle \tan {\frac {\pi }{60}}=\tan 3^{\circ }={\tfrac {1}{4}}\left[(2-{\sqrt {3}})(3+{\sqrt {5}})-2\right]\left[2-{\sqrt {2(5-{\sqrt {5}})}}\right]\,}
sin
(
π
48
)
=
sin
(
3.75
∘
)
=
1
2
2
−
2
+
2
+
3
{\displaystyle \sin \left({\frac {\pi }{48}}\right)=\sin \left(3.75^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {3}}}}}}}}}
cos
(
π
48
)
=
cos
(
3.75
∘
)
=
1
2
2
+
2
+
2
+
3
{\displaystyle \cos \left({\frac {\pi }{48}}\right)=\cos \left(3.75^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {3}}}}}}}}}
sin
4
∘
=
1
2
i
(
cos
12
∘
+
i
sin
12
∘
3
−
cos
12
∘
−
i
sin
12
∘
3
)
{\displaystyle \sin {4^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {12^{\circ }}+i\sin {12^{\circ }}}}-{\sqrt[{3}]{\cos {12^{\circ }}-i\sin {12^{\circ }}}}\right)}
=
1
4
i
{
[
6
(
5
+
5
)
+
5
−
1
]
+
i
[
2
(
5
+
5
)
−
3
(
5
−
1
)
]
3
{\displaystyle ={\frac {1}{4i}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]+i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}}
−
[
6
(
5
+
5
)
+
5
−
1
]
−
i
[
2
(
5
+
5
)
−
3
(
5
−
1
)
]
3
}
{\displaystyle -{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]-i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}{\Bigg \}}}
cos
4
∘
=
1
2
(
cos
12
∘
+
i
sin
12
∘
3
+
cos
12
∘
−
i
sin
12
∘
3
)
{\displaystyle \cos {4^{\circ }}={\frac {1}{2}}\left({\sqrt[{3}]{\cos {12^{\circ }}+i\sin {12^{\circ }}}}+{\sqrt[{3}]{\cos {12^{\circ }}-i\sin {12^{\circ }}}}\right)}
=
1
4
{
[
6
(
5
+
5
)
+
5
−
1
]
+
i
[
2
(
5
+
5
)
−
3
(
5
−
1
)
]
3
{\displaystyle ={\frac {1}{4}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]+i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}}
+
[
6
(
5
+
5
)
+
5
−
1
]
−
i
[
2
(
5
+
5
)
−
3
(
5
−
1
)
]
3
}
{\displaystyle +{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]-i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}{\Bigg \}}}
sin
(
π
40
)
=
sin
(
4.5
∘
)
=
1
2
2
−
2
+
5
+
5
2
{\displaystyle \sin \left({\frac {\pi }{40}}\right)=\sin \left(4.5^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {\frac {5+{\sqrt {5}}}{2}}}}}}}}
cos
(
π
40
)
=
cos
(
4.5
∘
)
=
1
2
2
+
2
+
5
+
5
2
{\displaystyle \cos \left({\frac {\pi }{40}}\right)=\cos \left(4.5^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {\frac {5+{\sqrt {5}}}{2}}}}}}}}
sin
π
36
=
sin
5
∘
=
2
−
2
3
i
2
2
(
2
−
6
)
3
−
2
−
3
−
(
1
+
3
i
)
2
(
2
−
6
)
3
−
2
−
3
8
{\displaystyle \sin {\frac {\pi }{36}}=\sin 5^{\circ }={\frac {2-2{\sqrt {3}}\mathrm {i} }{2{\sqrt[{3}]{2({\sqrt {2}}-{\sqrt {6}})}}-2-{\sqrt {3}}}}-{\frac {(1+{\sqrt {3}}\mathrm {i} ){\sqrt[{3}]{2({\sqrt {2}}-{\sqrt {6}})}}-2-{\sqrt {3}}}{8}}\,}
sin
(
π
32
)
=
sin
(
5.625
∘
)
=
1
2
2
−
2
+
2
+
2
{\displaystyle \sin \left({\frac {\pi }{32}}\right)=\sin \left(5.625^{\circ }\right)={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}}}
cos
(
π
32
)
=
cos
(
5.625
∘
)
=
1
2
2
+
2
+
2
+
2
{\displaystyle \cos \left({\frac {\pi }{32}}\right)=\cos \left(5.625^{\circ }\right)={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}}}
sin
π
30
=
sin
6
∘
=
1
8
[
6
(
5
−
5
)
−
5
−
1
]
{\displaystyle \sin {\frac {\pi }{30}}=\sin 6^{\circ }={\tfrac {1}{8}}\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]\,}
cos
π
30
=
cos
6
∘
=
1
8
[
2
(
5
−
5
)
+
3
(
5
+
1
)
]
{\displaystyle \cos {\frac {\pi }{30}}=\cos 6^{\circ }={\tfrac {1}{8}}\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]\,}
tan
π
30
=
tan
6
∘
=
1
2
[
2
(
5
−
5
)
−
3
(
5
−
1
)
]
{\displaystyle \tan {\frac {\pi }{30}}=\tan 6^{\circ }={\tfrac {1}{2}}\left[{\sqrt {2(5-{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]\,}
cot
π
30
=
cot
6
∘
=
1
2
(
50
+
22
5
+
3
3
+
15
)
{\displaystyle \cot {\frac {\pi }{30}}=\cot 6^{\circ }={\tfrac {1}{2}}\left({\sqrt {50+22{\sqrt {5}}}}+3{\sqrt {3}}+{\sqrt {15}}\right)\,}
sec
π
30
=
sec
6
∘
=
3
−
5
−
2
5
{\displaystyle \sec {\frac {\pi }{30}}=\sec 6^{\circ }={\sqrt {3}}-{\sqrt {5-2{\sqrt {5}}}}\,}
csc
π
30
=
csc
6
∘
=
2
+
5
+
15
+
6
5
{\displaystyle \csc {\frac {\pi }{30}}=\csc 6^{\circ }=2+{\sqrt {5}}+{\sqrt {15+6{\sqrt {5}}}}\,}
sin
π
24
=
sin
7.5
∘
=
1
4
8
−
2
6
−
2
2
{\displaystyle \sin {\frac {\pi }{24}}=\sin 7.5^{\circ }={\tfrac {1}{4}}{\sqrt {8-2{\sqrt {6}}-2{\sqrt {2}}}}\,}
cos
π
24
=
cos
7.5
∘
=
1
4
8
+
2
6
+
2
2
{\displaystyle \cos {\frac {\pi }{24}}=\cos 7.5^{\circ }={\tfrac {1}{4}}{\sqrt {8+2{\sqrt {6}}+2{\sqrt {2}}}}\,}
tan
π
24
=
tan
7.5
∘
=
6
+
2
−
2
−
3
{\displaystyle \tan {\frac {\pi }{24}}=\tan 7.5^{\circ }={\sqrt {6}}+{\sqrt {2}}-2-{\sqrt {3}}\,}
cot
π
24
=
cot
7.5
∘
=
6
+
2
+
2
+
3
{\displaystyle \cot {\frac {\pi }{24}}=\cot 7.5^{\circ }={\sqrt {6}}+{\sqrt {2}}+2+{\sqrt {3}}\,}
sec
π
24
=
sec
7.5
∘
=
16
−
6
6
−
10
2
+
8
3
{\displaystyle \sec {\frac {\pi }{24}}=\sec 7.5^{\circ }={\sqrt {16-6{\sqrt {6}}-10{\sqrt {2}}+8{\sqrt {3}}}}\,}
csc
π
24
=
csc
7.5
∘
=
16
+
6
6
+
10
2
+
8
3
{\displaystyle \csc {\frac {\pi }{24}}=\csc 7.5^{\circ }={\sqrt {16+6{\sqrt {6}}+10{\sqrt {2}}+8{\sqrt {3}}}}\,}
sin
π
20
=
sin
9
∘
=
1
4
8
−
2
10
+
2
5
{\displaystyle \sin {\frac {\pi }{20}}=\sin 9^{\circ }={\tfrac {1}{4}}{\sqrt {8-2{\sqrt {10+2{\sqrt {5}}}}}}\,}
cos
π
20
=
cos
9
∘
=
1
4
8
+
2
10
+
2
5
{\displaystyle \cos {\frac {\pi }{20}}=\cos 9^{\circ }={\tfrac {1}{4}}{\sqrt {8+2{\sqrt {10+2{\sqrt {5}}}}}}\,}
tan
π
20
=
tan
9
∘
=
5
+
1
−
5
+
2
5
{\displaystyle \tan {\frac {\pi }{20}}=\tan 9^{\circ }={\sqrt {5}}+1-{\sqrt {5+2{\sqrt {5}}}}\,}
cot
π
20
=
cot
9
∘
=
5
+
1
+
5
+
2
5
{\displaystyle \cot {\frac {\pi }{20}}=\cot 9^{\circ }={\sqrt {5}}+1+{\sqrt {5+2{\sqrt {5}}}}\,}
tan
10
∘
=
−
−
1
−
3
i
6
−
12
3
+
36
i
3
−
−
1
+
3
i
6
−
12
3
−
36
i
3
+
1
3
{\displaystyle {\tan 10^{\circ }=-{\frac {-1-{\sqrt {3}}{\rm {i}}}{6}}{\sqrt[{3}]{-12{\sqrt {3}}+36{\rm {i}}}}-{\frac {-1+{\sqrt {3}}{\rm {i}}}{6}}{\sqrt[{3}]{-12{\sqrt {3}}-36{\rm {i}}}}+{\frac {1}{\sqrt {3}}}}\,}
sin
π
16
=
sin
11.25
∘
=
1
2
2
−
2
+
2
{\displaystyle \sin {\frac {\pi }{16}}=\sin 11.25^{\circ }={\frac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2}}}}}}}
cos
π
16
=
cos
11.25
∘
=
1
2
2
+
2
+
2
{\displaystyle \cos {\frac {\pi }{16}}=\cos 11.25^{\circ }={\frac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}
tan
π
16
=
tan
11.25
∘
=
4
+
2
2
−
2
−
1
{\displaystyle \tan {\frac {\pi }{16}}=\tan 11.25^{\circ }={\sqrt {4+2{\sqrt {2}}}}-{\sqrt {2}}-1}
cot
π
16
=
cot
11.25
∘
=
4
+
2
2
+
2
+
1
{\displaystyle \cot {\frac {\pi }{16}}=\cot 11.25^{\circ }={\sqrt {4+2{\sqrt {2}}}}+{\sqrt {2}}+1}
sin
π
15
=
sin
12
∘
=
1
8
[
2
(
5
+
5
)
−
3
(
5
−
1
)
]
{\displaystyle \sin {\frac {\pi }{15}}=\sin 12^{\circ }={\tfrac {1}{8}}\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]\,}
cos
π
15
=
cos
12
∘
=
1
8
[
6
(
5
+
5
)
+
5
−
1
]
{\displaystyle \cos {\frac {\pi }{15}}=\cos 12^{\circ }={\tfrac {1}{8}}\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]\,}
tan
π
15
=
tan
12
∘
=
1
2
[
3
(
3
−
5
)
−
2
(
25
−
11
5
)
]
{\displaystyle \tan {\frac {\pi }{15}}=\tan 12^{\circ }={\tfrac {1}{2}}\left[{\sqrt {3}}(3-{\sqrt {5}})-{\sqrt {2(25-11{\sqrt {5}})}}\right]\,}
sin
π
12
=
sin
15
∘
=
1
4
2
(
3
−
1
)
{\displaystyle \sin {\frac {\pi }{12}}=\sin 15^{\circ }={\tfrac {1}{4}}{\sqrt {2}}\left({\sqrt {3}}-1\right)\,}
cos
π
12
=
cos
15
∘
=
1
4
2
(
3
+
1
)
{\displaystyle \cos {\frac {\pi }{12}}=\cos 15^{\circ }={\tfrac {1}{4}}{\sqrt {2}}\left({\sqrt {3}}+1\right)\,}
tan
π
12
=
tan
15
∘
=
2
−
3
{\displaystyle \tan {\frac {\pi }{12}}=\tan 15^{\circ }=2-{\sqrt {3}}\,}
sin
π
10
=
sin
18
∘
=
1
4
(
5
−
1
)
=
1
2
φ
−
1
{\displaystyle \sin {\frac {\pi }{10}}=\sin 18^{\circ }={\tfrac {1}{4}}\left({\sqrt {5}}-1\right)={\tfrac {1}{2}}\varphi ^{-1}\,}
cos
π
10
=
cos
18
∘
=
1
4
2
(
5
+
5
)
{\displaystyle \cos {\frac {\pi }{10}}=\cos 18^{\circ }={\tfrac {1}{4}}{\sqrt {2\left(5+{\sqrt {5}}\right)}}\,}
tan
π
10
=
tan
18
∘
=
1
5
5
(
5
−
2
5
)
{\displaystyle \tan {\frac {\pi }{10}}=\tan 18^{\circ }={\tfrac {1}{5}}{\sqrt {5\left(5-2{\sqrt {5}}\right)}}\,}
sin
π
9
=
sin
20
∘
=
−
3
16
+
−
1
256
3
+
−
3
16
−
−
1
256
3
=
{\displaystyle \sin {\frac {\pi }{9}}=\sin 20^{\circ }={\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}+{\sqrt {-{\frac {1}{256}}}}}}+{\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}-{\sqrt {-{\frac {1}{256}}}}}}=}
2
−
4
3
(
i
−
3
3
−
i
+
3
3
)
{\displaystyle 2^{-{\frac {4}{3}}}\left({\sqrt[{3}]{i-{\sqrt {3}}}}-{\sqrt[{3}]{i+{\sqrt {3}}}}\right)}
cos
π
9
=
cos
20
∘
=
{\displaystyle \cos {\frac {\pi }{9}}=\cos 20^{\circ }=}
2
−
4
3
(
1
+
i
3
3
+
1
−
i
3
3
)
{\displaystyle 2^{-{\frac {4}{3}}}\left({\sqrt[{3}]{1+i{\sqrt {3}}}}+{\sqrt[{3}]{1-i{\sqrt {3}}}}\right)}
sin
7
π
60
=
sin
21
∘
=
1
4
8
+
3
−
15
−
10
+
2
5
{\displaystyle \sin {\frac {7\pi }{60}}=\sin 21^{\circ }={\tfrac {1}{4}}{\sqrt {8+{\sqrt {3}}-{\sqrt {15}}-{\sqrt {10+2{\sqrt {5}}}}}}\,}
cos
7
π
60
=
cos
21
∘
=
1
4
8
−
3
+
15
+
10
+
2
5
{\displaystyle \cos {\frac {7\pi }{60}}=\cos 21^{\circ }={\tfrac {1}{4}}{\sqrt {8-{\sqrt {3}}+{\sqrt {15}}+{\sqrt {10+2{\sqrt {5}}}}}}\,}
tan
7
π
60
=
tan
21
∘
=
1
4
[
2
−
(
2
+
3
)
(
3
−
5
)
]
[
2
−
2
(
5
+
5
)
]
{\displaystyle \tan {\frac {7\pi }{60}}=\tan 21^{\circ }={\tfrac {1}{4}}\left[2-\left(2+{\sqrt {3}}\right)\left(3-{\sqrt {5}}\right)\right]\left[2-{\sqrt {2\left(5+{\sqrt {5}}\right)}}\right]\,}
(
21
3
17
)
∘
{\displaystyle \mathbf {\left(21{\frac {3}{17}}\right)^{\circ }} }
(
360
17
)
∘
{\displaystyle \mathbf {\left({\frac {360}{17}}\right)^{\circ }} }
cos
2
π
17
=
−
1
+
17
+
34
−
2
17
+
2
17
+
3
17
−
34
−
2
17
−
2
34
+
2
17
16
{\displaystyle \operatorname {cos} {2\pi \over 17}={\frac {-1+{\sqrt {17}}+{\sqrt {34-2{\sqrt {17}}}}+2{\sqrt {17+3{\sqrt {17}}-{\sqrt {34-2{\sqrt {17}}}}-2{\sqrt {34+2{\sqrt {17}}}}}}}{16}}}
sin
π
8
=
sin
22.5
∘
=
1
2
(
2
−
2
)
{\displaystyle \sin {\frac {\pi }{8}}=\sin 22.5^{\circ }={\tfrac {1}{2}}({\sqrt {2-{\sqrt {2}}}})}
cos
π
8
=
cos
22.5
∘
=
1
2
(
2
+
2
)
{\displaystyle \cos {\frac {\pi }{8}}=\cos 22.5^{\circ }={\tfrac {1}{2}}({\sqrt {2+{\sqrt {2}}}})\,}
tan
π
8
=
tan
22.5
∘
=
2
−
1
{\displaystyle \tan {\frac {\pi }{8}}=\tan 22.5^{\circ }={\sqrt {2}}-1\,}
sin
2
π
15
=
sin
24
∘
=
1
8
[
3
(
5
+
1
)
−
2
5
−
5
]
{\displaystyle \sin {\frac {2\pi }{15}}=\sin 24^{\circ }={\tfrac {1}{8}}\left[{\sqrt {3}}({\sqrt {5}}+1)-{\sqrt {2}}{\sqrt {5-{\sqrt {5}}}}\right]\,}
cos
2
π
15
=
cos
24
∘
=
1
8
(
6
5
−
5
+
5
+
1
)
{\displaystyle \cos {\frac {2\pi }{15}}=\cos 24^{\circ }={\tfrac {1}{8}}\left({\sqrt {6}}{\sqrt {5-{\sqrt {5}}}}+{\sqrt {5}}+1\right)\,}
tan
2
π
15
=
tan
24
∘
=
1
2
[
2
(
25
+
11
5
)
−
3
(
3
+
5
)
]
{\displaystyle \tan {\frac {2\pi }{15}}=\tan 24^{\circ }={\tfrac {1}{2}}\left[{\sqrt {2(25+11{\sqrt {5}})}}-{\sqrt {3}}(3+{\sqrt {5}})\right]\,}
(
25
5
7
)
∘
{\displaystyle \mathbf {\left(25{\frac {5}{7}}\right)^{\circ }} }
(
180
7
)
∘
{\displaystyle \mathbf {\left({\frac {180}{7}}\right)^{\circ }} }
cos
π
7
=
cos
180
7
∘
=
cos
25
5
7
∘
=
1
6
+
1
−
3
i
24
28
−
84
3
i
3
+
1
+
3
i
24
28
−
84
3
i
3
{\displaystyle \cos {\frac {\pi }{7}}=\cos {\frac {180}{7}}^{\circ }=\cos 25{\frac {5}{7}}^{\circ }={\frac {1}{6}}+{\frac {1-{\sqrt {3}}i}{24}}{\sqrt[{3}]{28-84{\sqrt {3}}i}}+{\frac {1+{\sqrt {3}}i}{24}}{\sqrt[{3}]{28-84{\sqrt {3}}i}}}
sin
3
π
20
=
sin
27
∘
=
1
8
[
2
5
+
5
−
2
(
5
−
1
)
]
{\displaystyle \sin {\frac {3\pi }{20}}=\sin 27^{\circ }={\tfrac {1}{8}}\left[2{\sqrt {5+{\sqrt {5}}}}-{\sqrt {2}}\;({\sqrt {5}}-1)\right]\,}
cos
3
π
20
=
cos
27
∘
=
1
8
[
2
5
+
5
+
2
(
5
−
1
)
]
{\displaystyle \cos {\frac {3\pi }{20}}=\cos 27^{\circ }={\tfrac {1}{8}}\left[2{\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}\;\left({\sqrt {5}}-1\right)\right]\,}
tan
3
π
20
=
tan
27
∘
=
5
−
1
−
5
−
2
5
{\displaystyle \tan {\frac {3\pi }{20}}=\tan 27^{\circ }={\sqrt {5}}-1-{\sqrt {5-2{\sqrt {5}}}}\,}
sin
π
6
=
sin
30
∘
=
1
2
{\displaystyle \sin {\frac {\pi }{6}}=\sin 30^{\circ }={\tfrac {1}{2}}\,}
cos
π
6
=
cos
30
∘
=
1
2
3
{\displaystyle \cos {\frac {\pi }{6}}=\cos 30^{\circ }={\tfrac {1}{2}}{\sqrt {3}}\,}
tan
π
6
=
tan
30
∘
=
1
3
3
{\displaystyle \tan {\frac {\pi }{6}}=\tan 30^{\circ }={\tfrac {1}{3}}{\sqrt {3}}\,}
sin
11
π
60
=
sin
33
∘
=
1
4
8
−
3
−
15
+
10
−
2
5
{\displaystyle \sin {\frac {11\pi }{60}}=\sin 33^{\circ }={\tfrac {1}{4}}{\sqrt {8-{\sqrt {3}}-{\sqrt {15}}+{\sqrt {10-2{\sqrt {5}}}}}}\,}
cos
11
π
60
=
cos
33
∘
=
1
4
8
+
3
+
15
−
10
−
2
5
{\displaystyle \cos {\frac {11\pi }{60}}=\cos 33^{\circ }={\tfrac {1}{4}}{\sqrt {8+{\sqrt {3}}+{\sqrt {15}}-{\sqrt {10-2{\sqrt {5}}}}}}\,}
tan
11
π
60
=
tan
33
∘
=
1
4
(
2
3
−
5
−
1
)
(
2
5
+
2
5
+
3
+
5
)
{\displaystyle \tan {\frac {11\pi }{60}}=\tan 33^{\circ }={\tfrac {1}{4}}\left(2{\sqrt {3}}-{\sqrt {5}}-1\right)\left(2{\sqrt {5+2{\sqrt {5}}}}+3+{\sqrt {5}}\right)\,}
cot
11
π
60
=
cot
33
∘
=
1
4
(
2
3
+
5
+
1
)
(
2
5
+
2
5
−
3
−
5
)
{\displaystyle \cot {\frac {11\pi }{60}}=\cot 33^{\circ }={\tfrac {1}{4}}\left(2{\sqrt {3}}+{\sqrt {5}}+1\right)\left(2{\sqrt {5+2{\sqrt {5}}}}-3-{\sqrt {5}}\right)\,}
sin
π
5
=
sin
36
∘
=
1
4
[
2
(
5
−
5
)
]
{\displaystyle \sin {\frac {\pi }{5}}=\sin 36^{\circ }={\tfrac {1}{4}}\left[{\sqrt {2\left(5-{\sqrt {5}}\right)}}\right]\,}
cos
π
5
=
cos
36
∘
=
1
+
5
4
=
1
2
φ
{\displaystyle \cos {\frac {\pi }{5}}=\cos 36^{\circ }={\frac {1+{\sqrt {5}}}{4}}={\tfrac {1}{2}}\varphi \,}
tan
π
5
=
tan
36
∘
=
5
−
2
5
{\displaystyle \tan {\frac {\pi }{5}}=\tan 36^{\circ }={\sqrt {5-2{\sqrt {5}}}}\,}
sin
13
π
60
=
sin
39
∘
=
1
4
8
−
3
+
15
+
10
+
2
5
{\displaystyle \sin {\frac {13\pi }{60}}=\sin 39^{\circ }={\tfrac {1}{4}}{\sqrt {8-{\sqrt {3}}+{\sqrt {15}}+{\sqrt {10+2{\sqrt {5}}}}}}\,}
cos
13
π
60
=
cos
39
∘
=
1
4
8
+
3
−
15
+
10
+
2
5
{\displaystyle \cos {\frac {13\pi }{60}}=\cos 39^{\circ }={\tfrac {1}{4}}{\sqrt {8+{\sqrt {3}}-{\sqrt {15}}+{\sqrt {10+2{\sqrt {5}}}}}}\,}
tan
13
π
60
=
tan
39
∘
=
1
4
[
(
2
−
3
)
(
3
−
5
)
−
2
]
[
2
−
2
(
5
+
5
)
]
{\displaystyle \tan {\frac {13\pi }{60}}=\tan 39^{\circ }={\tfrac {1}{4}}\left[\left(2-{\sqrt {3}}\right)\left(3-{\sqrt {5}}\right)-2\right]\left[2-{\sqrt {2\left(5+{\sqrt {5}}\right)}}\right]\,}
sin
7
π
30
=
sin
42
∘
=
6
5
+
5
−
5
+
1
8
{\displaystyle \sin {\frac {7\pi }{30}}=\sin 42^{\circ }={\frac {{\sqrt {6}}{\sqrt {5+{\sqrt {5}}}}-{\sqrt {5}}+1}{8}}\,}
cos
7
π
30
=
cos
42
∘
=
2
5
+
5
+
3
(
5
−
1
)
8
{\displaystyle \cos {\frac {7\pi }{30}}=\cos 42^{\circ }={\frac {{\sqrt {2}}{\sqrt {5+{\sqrt {5}}}}+{\sqrt {3}}\left({\sqrt {5}}-1\right)}{8}}\,}
tan
7
π
30
=
tan
42
∘
=
1
2
(
3
+
15
−
10
+
2
5
)
{\displaystyle \tan {\frac {7\pi }{30}}=\tan 42^{\circ }={\frac {1}{2}}\left({\sqrt {3}}+{\sqrt {15}}-{\sqrt {10+2{\sqrt {5}}}}\right)\,}
cot
7
π
30
=
cot
42
∘
=
1
2
(
3
3
−
15
+
50
−
22
5
)
{\displaystyle \cot {\frac {7\pi }{30}}=\cot 42^{\circ }={\frac {1}{2}}\left(3{\sqrt {3}}-{\sqrt {15}}+{\sqrt {50-22{\sqrt {5}}}}\right)\,}
sec
7
π
30
=
sec
42
∘
=
5
+
2
5
−
3
{\displaystyle \sec {\frac {7\pi }{30}}=\sec 42^{\circ }={\sqrt {5+2{\sqrt {5}}}}-{\sqrt {3}}\,}
sec
7
π
30
=
sec
42
∘
=
15
−
6
5
+
5
−
2
{\displaystyle \sec {\frac {7\pi }{30}}=\sec 42^{\circ }={\sqrt {15-6{\sqrt {5}}}}+{\sqrt {5}}-2\,}
sin
π
4
=
sin
45
∘
=
2
2
=
1
2
{\displaystyle \sin {\frac {\pi }{4}}=\sin 45^{\circ }={\frac {\sqrt {2}}{2}}={\frac {1}{\sqrt {2}}}\,}
cos
π
4
=
cos
45
∘
=
2
2
=
1
2
{\displaystyle \cos {\frac {\pi }{4}}=\cos 45^{\circ }={\frac {\sqrt {2}}{2}}={\frac {1}{\sqrt {2}}}\,}
tan
π
4
=
tan
45
∘
=
1
{\displaystyle \tan {\frac {\pi }{4}}=\tan 45^{\circ }=1}
sin
3
π
10
=
sin
54
∘
=
5
+
1
4
{\displaystyle \sin {\frac {3\pi }{10}}=\sin 54^{\circ }={\frac {{\sqrt {5}}+1}{4}}\,\!}
cos
3
π
10
=
cos
54
∘
=
10
−
2
5
4
{\displaystyle \cos {\frac {3\pi }{10}}=\cos 54^{\circ }={\frac {\sqrt {10-2{\sqrt {5}}}}{4}}}
tan
3
π
10
=
tan
54
∘
=
25
+
10
5
5
{\displaystyle \tan {\frac {3\pi }{10}}=\tan 54^{\circ }={\frac {\sqrt {25+10{\sqrt {5}}}}{5}}\,}
cot
3
π
10
=
cot
54
∘
=
5
−
20
{\displaystyle \cot {\frac {3\pi }{10}}=\cot 54^{\circ }={\sqrt {5-{\sqrt {20}}}}\,}
sin
π
3
=
sin
60
∘
=
3
2
{\displaystyle \sin {\frac {\pi }{3}}=\sin 60^{\circ }={\frac {\sqrt {3}}{2}}\,}
cos
π
3
=
cos
60
∘
=
1
2
{\displaystyle \cos {\frac {\pi }{3}}=\cos 60^{\circ }={\frac {1}{2}}\,}
tan
π
3
=
tan
60
∘
=
3
{\displaystyle \tan {\frac {\pi }{3}}=\tan 60^{\circ }={\sqrt {3}}\,}
cot
π
3
=
cot
60
∘
=
3
3
=
1
3
{\displaystyle \cot {\frac {\pi }{3}}=\cot 60^{\circ }={\frac {\sqrt {3}}{3}}={\frac {1}{\sqrt {3}}}\,}
sin
3
π
8
=
sin
67.5
∘
=
1
2
2
+
2
{\displaystyle \sin {\frac {3\pi }{8}}=\sin 67.5^{\circ }={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2}}}}\,}
cos
3
π
8
=
cos
67.5
∘
=
1
2
2
−
2
{\displaystyle \cos {\frac {3\pi }{8}}=\cos 67.5^{\circ }={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2}}}}\,}
tan
3
π
8
=
tan
67.5
∘
=
2
+
1
{\displaystyle \tan {\frac {3\pi }{8}}=\tan 67.5^{\circ }={\sqrt {2}}+1\,}
cot
3
π
8
=
cot
67.5
∘
=
2
−
1
{\displaystyle \cot {\frac {3\pi }{8}}=\cot 67.5^{\circ }={\sqrt {2}}-1\,}
sin
2
π
5
=
sin
72
∘
=
1
4
2
(
5
+
5
)
{\displaystyle \sin {\frac {2\pi }{5}}=\sin 72^{\circ }={\tfrac {1}{4}}{\sqrt {2\left(5+{\sqrt {5}}\right)}}\,}
cos
2
π
5
=
cos
72
∘
=
1
4
(
5
−
1
)
{\displaystyle \cos {\frac {2\pi }{5}}=\cos 72^{\circ }={\tfrac {1}{4}}\left({\sqrt {5}}-1\right)\,}
tan
2
π
5
=
tan
72
∘
=
5
+
2
5
{\displaystyle \tan {\frac {2\pi }{5}}=\tan 72^{\circ }={\sqrt {5+2{\sqrt {5}}}}\,}
cot
2
π
5
=
cot
72
∘
=
1
5
5
(
5
−
2
5
)
{\displaystyle \cot {\frac {2\pi }{5}}=\cot 72^{\circ }={\tfrac {1}{5}}{\sqrt {5\left(5-2{\sqrt {5}}\right)}}\,}
sin
5
π
12
=
sin
75
∘
=
1
4
(
6
+
2
)
{\displaystyle \sin {\frac {5\pi }{12}}=\sin 75^{\circ }={\tfrac {1}{4}}\left({\sqrt {6}}+{\sqrt {2}}\right)\,}
cos
5
π
12
=
cos
75
∘
=
1
4
(
6
−
2
)
{\displaystyle \cos {\frac {5\pi }{12}}=\cos 75^{\circ }={\tfrac {1}{4}}\left({\sqrt {6}}-{\sqrt {2}}\right)\,}
tan
5
π
12
=
tan
75
∘
=
2
+
3
{\displaystyle \tan {\frac {5\pi }{12}}=\tan 75^{\circ }=2+{\sqrt {3}}\,}
cot
5
π
12
=
cot
75
∘
=
2
−
3
{\displaystyle \cot {\frac {5\pi }{12}}=\cot 75^{\circ }=2-{\sqrt {3}}\,}
sin
π
2
=
sin
90
∘
=
1
{\displaystyle \sin {\frac {\pi }{2}}=\sin 90^{\circ }=1\,}
cos
π
2
=
cos
90
∘
=
0
{\displaystyle \cos {\frac {\pi }{2}}=\cos 90^{\circ }=0\,}
cot
π
2
=
cot
90
∘
=
0
{\displaystyle \cot {\frac {\pi }{2}}=\cot 90^{\circ }=0\,}
在下表中,
i
{\displaystyle i}
虛數單位 ,
ω
=
exp
(
π
i
3
)
=
−
1
2
+
1
2
i
3
{\displaystyle \omega =\exp({\frac {\pi i}{3}})=-{\frac {1}{2}}+{\frac {1}{2}}i{\sqrt {3}}}
n
{\displaystyle n}
sin
(
2
π
n
)
{\displaystyle \sin \left({\frac {2\pi }{n}}\right)}
cos
(
2
π
n
)
{\displaystyle \cos \left({\frac {2\pi }{n}}\right)}
tan
(
2
π
n
)
{\displaystyle \tan \left({\frac {2\pi }{n}}\right)}
1
0
{\displaystyle 0}
1
{\displaystyle 1}
0
{\displaystyle 0}
2
0
{\displaystyle 0}
−
1
{\displaystyle -1}
0
{\displaystyle 0}
3
1
2
3
{\displaystyle {\frac {1}{2}}{\sqrt {3}}}
−
1
2
{\displaystyle -{\frac {1}{2}}}
−
3
{\displaystyle -{\sqrt {3}}}
4
1
{\displaystyle 1}
0
{\displaystyle 0}
±
∞
{\displaystyle \pm \infty }
5
1
4
(
10
+
2
5
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {10+2{\sqrt {5}}}}\right)}
1
4
(
5
−
1
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {5}}-1\right)}
5
+
2
5
{\displaystyle {\sqrt {5+2{\sqrt {5}}}}}
6
1
2
3
{\displaystyle {\frac {1}{2}}{\sqrt {3}}}
1
2
{\displaystyle {\frac {1}{2}}}
3
{\displaystyle {\sqrt {3}}}
7
1
2
1
3
(
7
−
ω
2
7
+
21
−
3
2
3
−
ω
7
−
21
−
3
2
3
)
{\displaystyle {\frac {1}{2}}{\sqrt {{\frac {1}{3}}\left(7-\omega ^{2}{\sqrt[{3}]{\frac {7+21{\sqrt {-3}}}{2}}}-\omega {\sqrt[{3}]{\frac {7-21{\sqrt {-3}}}{2}}}\right)}}}
1
6
(
−
1
+
7
+
21
−
3
2
3
+
7
−
21
−
3
2
3
)
{\displaystyle {\frac {1}{6}}\left(-1+{\sqrt[{3}]{\frac {7+21{\sqrt {-3}}}{2}}}+{\sqrt[{3}]{\frac {7-21{\sqrt {-3}}}{2}}}\right)}
8
1
2
2
{\displaystyle {\frac {1}{2}}{\sqrt {2}}}
1
2
2
{\displaystyle {\frac {1}{2}}{\sqrt {2}}}
1
{\displaystyle 1}
9
1
4
(
4
(
−
1
+
−
3
)
3
+
4
(
−
1
−
−
3
)
3
)
{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4(-1+{\sqrt {-3}})}}+{\sqrt[{3}]{4(-1-{\sqrt {-3}})}}\right)}
10
1
4
(
10
−
2
5
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {10-2{\sqrt {5}}}}\right)}
1
4
(
5
+
1
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {5}}+1\right)}
5
−
2
5
{\displaystyle {\sqrt {5-2{\sqrt {5}}}}}
11
12
1
2
{\displaystyle {\frac {1}{2}}}
1
2
3
{\displaystyle {\frac {1}{2}}{\sqrt {3}}}
1
3
3
{\displaystyle {\frac {1}{3}}{\sqrt {3}}}
13
14
1
24
3
(
112
−
14336
+
−
5549064193
3
−
14336
−
−
5549064193
3
)
{\displaystyle {\frac {1}{24}}{\sqrt {3\left(112-{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}-{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}\right)}}}
1
24
3
(
80
+
14336
+
−
5549064193
3
+
14336
−
−
5549064193
3
)
{\displaystyle {\frac {1}{24}}{\sqrt {3\left(80+{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}+{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}\right)}}}
112
−
14336
+
−
5549064193
3
−
14336
−
−
5549064193
3
80
+
14336
+
−
5549064193
3
+
14336
−
−
5549064193
3
{\displaystyle {\sqrt {\frac {112-{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}-{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}}{80+{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}+{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}}}}}
15
1
8
(
15
+
3
−
10
−
2
5
)
{\displaystyle {\frac {1}{8}}\left({\sqrt {15}}+{\sqrt {3}}-{\sqrt {10-2{\sqrt {5}}}}\right)}
1
8
(
1
+
5
+
30
−
6
5
)
{\displaystyle {\frac {1}{8}}\left(1+{\sqrt {5}}+{\sqrt {30-6{\sqrt {5}}}}\right)}
1
2
(
−
3
3
−
15
+
50
+
22
5
)
{\displaystyle {\frac {1}{2}}\left(-3{\sqrt {3}}-{\sqrt {15}}+{\sqrt {50+22{\sqrt {5}}}}\right)}
16
1
2
(
2
−
2
)
{\displaystyle {\frac {1}{2}}\left({\sqrt {2-{\sqrt {2}}}}\right)}
1
2
(
2
+
2
)
{\displaystyle {\frac {1}{2}}\left({\sqrt {2+{\sqrt {2}}}}\right)}
2
−
1
{\displaystyle {\sqrt {2}}-1}
17
1
16
(
−
1
+
17
+
34
−
2
17
+
2
17
+
3
17
−
34
−
2
17
−
2
34
+
2
17
)
{\displaystyle {\frac {1}{16}}\left(-1+{\sqrt {17}}+{\sqrt {34-2{\sqrt {17}}}}+2{\sqrt {17+3{\sqrt {17}}-{\sqrt {34-2{\sqrt {17}}}}-2{\sqrt {34+2{\sqrt {17}}}}}}\right)}
18
1
4
(
4
−
1
−
4
3
3
−
4
−
1
+
4
3
3
)
{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4{\sqrt {-1}}-4{\sqrt {3}}}}-{\sqrt[{3}]{4{\sqrt {-1}}+4{\sqrt {3}}}}\right)}
1
4
(
4
+
4
−
3
3
+
4
−
4
−
3
3
)
{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4+4{\sqrt {-3}}}}+{\sqrt[{3}]{4-4{\sqrt {-3}}}}\right)}
19
20
1
4
(
5
−
1
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {5}}-1\right)}
1
4
(
10
+
2
5
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {10+2{\sqrt {5}}}}\right)}
1
5
(
25
−
10
5
)
{\displaystyle {\frac {1}{5}}\left({\sqrt {25-10{\sqrt {5}}}}\right)}
21
22
23
24
1
4
(
6
−
2
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {6}}-{\sqrt {2}}\right)}
1
4
(
6
+
2
)
{\displaystyle {\frac {1}{4}}\left({\sqrt {6}}+{\sqrt {2}}\right)}
2
−
3
{\displaystyle 2-{\sqrt {3}}}
埃里克·韦斯坦因 . Constructible polygon . MathWorld . 埃里克·韦斯坦因 . Trigonometry angles . MathWorld . Bracken, Paul; Cizek, Jiri. Evaluation of quantum mechanical perturbation sums in terms of quadratic surds and their use in approximation of zeta(3)/pi^3. Int. J. Quantum Chemistry. 2002, 90 (1): 42–53. doi:10.1002/qua.1803 Conway, John H.; Radin, Charles; Radun, Lorenzo. On angles whose squared trigonometric functions are rational. 1998. arXiv:math-ph/9812019 Conway, John H.; Radin, Charles; Radun, Lorenzo. On angles whose squared trigonometric functions are rational. Disc. Comput. Geom. 1999, 22 (3): 321–332. doi:10.1007/PL00009463 MR 1706614 Girstmair, Kurt. Some linear relations between values of trigonometric functions at k*pi/n. Acta Arithmetica. 1997, 81 : 387–398. MR 1472818 Gurak, S. On the minimal polynomial of gauss periods for prime powers. Mathematics of Computation. 2006, 75 (256): 2021–2035. Bibcode:2006MaCom..75.2021G doi:10.1090/S0025-5718-06-01885-0 MR 2240647 Servi, L. D. Nested square roots of 2. Am. Math. Monthly. 2003, 110 (4): 326–330. doi:10.2307/3647881 MR 1984573
^ 由Wolfram Alpha 验算:[1]
^ 使用Mathematica驗算,代碼為N[ArcSin[(1 + Sqrt[3] I)/16 Power[4 Sqrt[30] - 8 Sqrt[15 + 3 Sqrt[5]] + 8 Sqrt[5 + Sqrt[5]] + 4 Sqrt[10] - 4 Sqrt[6] - 4 Sqrt[2] + (4 Sqrt[30] + 8 Sqrt[15 + 3 Sqrt[5]] + 8 Sqrt[5 + Sqrt[5]] - 4 Sqrt[10] - 4 Sqrt[6] + 4 Sqrt[2]) I, (3)^-1] + (1 - Sqrt[3] I)/16 Power[4 Sqrt[30] - 8 Sqrt[15 + 3 Sqrt[5]] + 8 Sqrt[5 + Sqrt[5]] + 4 Sqrt[10] - 4 Sqrt[6] - 4 Sqrt[2] - (4 Sqrt[30] + 8 Sqrt[15 + 3 Sqrt[5]] + 8 Sqrt[5 + Sqrt[5]] - 4 Sqrt[10] - 4 Sqrt[6] + 4 Sqrt[2]) I, (3)^-1]], 100]/Degree結果為1與原角度無誤差


 .
.









































![{\displaystyle \sin {\frac {\pi }{9}}=\sin 20^{\circ }={\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}+{\sqrt {-{\frac {1}{256}}}}}}+{\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}-{\sqrt {-{\frac {1}{256}}}}}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/6019908a9d7a84324bc27625b5e5213830d24d8a)
![{\displaystyle \cos {\frac {\theta }{n}}=\Re \left({\sqrt[{n}]{\cos \theta +i\sin \theta }}\right)={\frac {1}{2}}\left({\sqrt[{n}]{\cos \theta +i\sin \theta }}+{\sqrt[{n}]{\cos \theta -i\sin \theta }}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/71c3d32e31b02c92e787505c9eafa8fa4e8cd828)
![{\displaystyle \sin {\frac {\theta }{n}}=\Im \left({\sqrt[{n}]{\cos \theta +i\sin \theta }}\right)={\frac {1}{2i}}\left({\sqrt[{n}]{\cos \theta +i\sin \theta }}-{\sqrt[{n}]{\cos \theta -i\sin \theta }}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/2ea663e7086395418f2d54876843352a2d0bf147)
![{\displaystyle \sin {1^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {3^{\circ }}+i\sin {3^{\circ }}}}-{\sqrt[{3}]{\cos {3^{\circ }}-i\sin {3^{\circ }}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/1c521d6e55fec7801936d21449aaaeb321b28e58)
![{\displaystyle ={\frac {1}{4{\sqrt[{3}]{2}}i}}{\Bigg \{}{\sqrt[{3}]{\left[2(1+{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}-1)\right]+i\left[2(1-{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}+1)\right]}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/2b16a535e1158d40e820a6c9cce5ca093eca02fe)
![{\displaystyle -{\sqrt[{3}]{\left[2(1+{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}-1)\right]-i\left[2(1-{\sqrt {3}}){\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}({\sqrt {5}}-1)({\sqrt {3}}+1)\right]}}{\Bigg \}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/066614d2502295fa13145c41b345358bf2238739)










![{\displaystyle \sin {1^{\circ }}={\frac {1+{\sqrt {3}}i}{16}}{\sqrt[{3}]{4{\sqrt {30}}-8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}+4{\sqrt {10}}-4{\sqrt {6}}-4{\sqrt {2}}+\left(4{\sqrt {30}}+8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}-4{\sqrt {10}}-4{\sqrt {6}}+4{\sqrt {2}}\right)i}}+}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/b9a7f8cda0d69d750a416bca1207841bab26cd6f)
![{\displaystyle {\frac {1-{\sqrt {3}}i}{16}}{\sqrt[{3}]{4{\sqrt {30}}-8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}+4{\sqrt {10}}-4{\sqrt {6}}-4{\sqrt {2}}-\left(4{\sqrt {30}}+8{\sqrt {15+3{\sqrt {5}}}}+8{\sqrt {5+{\sqrt {5}}}}-4{\sqrt {10}}-4{\sqrt {6}}+4{\sqrt {2}}\right)i}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/36c528db784d06bd39b38158572c4ae931517615)




![{\displaystyle \sin {2^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {6^{\circ }}+i\sin {6^{\circ }}}}-{\sqrt[{3}]{\cos {6^{\circ }}-i\sin {6^{\circ }}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/9cb6011d35cbc8d89b146f7fa0a014321c9f1d09)
![{\displaystyle ={\frac {1}{4i}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]+i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/76088f823ff50097d119f0df28cff18ea03e6c6e)
![{\displaystyle -{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]-i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}{\Bigg \}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/bc8b004b90010622da546669426b9a57564c5903)
![{\displaystyle \cos {2^{\circ }}={\frac {1}{2}}\left({\sqrt[{3}]{\cos {6^{\circ }}+i\sin {6^{\circ }}}}+{\sqrt[{3}]{\cos {6^{\circ }}-i\sin {6^{\circ }}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/6e40bf7865e0a5ceef13869ffa29c74560bced35)
![{\displaystyle ={\frac {1}{4}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]+i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/0ed4dc0c22095244d2f44b1a13799f3d249ebca5)
![{\displaystyle +{\sqrt[{3}]{\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]-i\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]}}{\Bigg \}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/57b380930ccaf8f99838d8352bcfc963be7680bd)






![{\displaystyle \tan {\frac {\pi }{60}}=\tan 3^{\circ }={\tfrac {1}{4}}\left[(2-{\sqrt {3}})(3+{\sqrt {5}})-2\right]\left[2-{\sqrt {2(5-{\sqrt {5}})}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/9afc8cae3b0181ce3ca0fa38cd9737a9096fc5de)


![{\displaystyle \sin {4^{\circ }}={\frac {1}{2i}}\left({\sqrt[{3}]{\cos {12^{\circ }}+i\sin {12^{\circ }}}}-{\sqrt[{3}]{\cos {12^{\circ }}-i\sin {12^{\circ }}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/622ba2d2d653fa9a61ba26ef726e4a46cc2acafb)
![{\displaystyle ={\frac {1}{4i}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]+i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/896906922b19b21a780a055f38801474ecafd495)
![{\displaystyle -{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]-i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}{\Bigg \}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/e2d8e586d6453abd5ac515018fcbfd022fa81011)
![{\displaystyle \cos {4^{\circ }}={\frac {1}{2}}\left({\sqrt[{3}]{\cos {12^{\circ }}+i\sin {12^{\circ }}}}+{\sqrt[{3}]{\cos {12^{\circ }}-i\sin {12^{\circ }}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/2100bbeb5c6a431e34e47bf0f26e4c67bddd1cbb)
![{\displaystyle ={\frac {1}{4}}{\Bigg \{}{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]+i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/d98084504214bdee5478f5af195113cb7476e2db)
![{\displaystyle +{\sqrt[{3}]{\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]-i\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]}}{\Bigg \}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/cbd37da55c50e7c12ca7ba6ebd337630973cf60c)


![{\displaystyle \sin {\frac {\pi }{36}}=\sin 5^{\circ }={\frac {2-2{\sqrt {3}}\mathrm {i} }{2{\sqrt[{3}]{2({\sqrt {2}}-{\sqrt {6}})}}-2-{\sqrt {3}}}}-{\frac {(1+{\sqrt {3}}\mathrm {i} ){\sqrt[{3}]{2({\sqrt {2}}-{\sqrt {6}})}}-2-{\sqrt {3}}}{8}}\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/74aa3cf4e94e893298f0dd9edba498d11b4a2249)


![{\displaystyle \sin {\frac {\pi }{30}}=\sin 6^{\circ }={\tfrac {1}{8}}\left[{\sqrt {6(5-{\sqrt {5}})}}-{\sqrt {5}}-1\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/e32eed64add3521d7d7c9bf695482e8c3add4c36)
![{\displaystyle \cos {\frac {\pi }{30}}=\cos 6^{\circ }={\tfrac {1}{8}}\left[{\sqrt {2(5-{\sqrt {5}})}}+{\sqrt {3}}({\sqrt {5}}+1)\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/f90aff5eb92b8553f8dfd11cd76f3f30bd223560)
![{\displaystyle \tan {\frac {\pi }{30}}=\tan 6^{\circ }={\tfrac {1}{2}}\left[{\sqrt {2(5-{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/ca95e17f7bfec511a0d9029c1aa1a0c8e5cc5630)













![{\displaystyle {\tan 10^{\circ }=-{\frac {-1-{\sqrt {3}}{\rm {i}}}{6}}{\sqrt[{3}]{-12{\sqrt {3}}+36{\rm {i}}}}-{\frac {-1+{\sqrt {3}}{\rm {i}}}{6}}{\sqrt[{3}]{-12{\sqrt {3}}-36{\rm {i}}}}+{\frac {1}{\sqrt {3}}}}\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/bff3bab5cf2730334855083f4576b03c09c9dd07)




![{\displaystyle \sin {\frac {\pi }{15}}=\sin 12^{\circ }={\tfrac {1}{8}}\left[{\sqrt {2(5+{\sqrt {5}})}}-{\sqrt {3}}({\sqrt {5}}-1)\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/55fb1a72c84d5dec8bf231d619c534df13c261ef)
![{\displaystyle \cos {\frac {\pi }{15}}=\cos 12^{\circ }={\tfrac {1}{8}}\left[{\sqrt {6(5+{\sqrt {5}})}}+{\sqrt {5}}-1\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/4923130317e1553f2cfd21ca42c4e29cd2f1f836)
![{\displaystyle \tan {\frac {\pi }{15}}=\tan 12^{\circ }={\tfrac {1}{2}}\left[{\sqrt {3}}(3-{\sqrt {5}})-{\sqrt {2(25-11{\sqrt {5}})}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/a207e48f104ece1821014496f7b32e371956076d)






![{\displaystyle \sin {\frac {\pi }{9}}=\sin 20^{\circ }={\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}+{\sqrt {-{\frac {1}{256}}}}}}+{\sqrt[{3}]{-{\frac {\sqrt {3}}{16}}-{\sqrt {-{\frac {1}{256}}}}}}=}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/1e39576a98052e848f2f9291c5d5da2437d6d056)
![{\displaystyle 2^{-{\frac {4}{3}}}\left({\sqrt[{3}]{i-{\sqrt {3}}}}-{\sqrt[{3}]{i+{\sqrt {3}}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/4fb7a6cadc9f67c9fc96b0b6bb7165af68824e9d)

![{\displaystyle 2^{-{\frac {4}{3}}}\left({\sqrt[{3}]{1+i{\sqrt {3}}}}+{\sqrt[{3}]{1-i{\sqrt {3}}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/c1fd794ae0ddf0128bdcedcca67ead75c8174e89)


![{\displaystyle \tan {\frac {7\pi }{60}}=\tan 21^{\circ }={\tfrac {1}{4}}\left[2-\left(2+{\sqrt {3}}\right)\left(3-{\sqrt {5}}\right)\right]\left[2-{\sqrt {2\left(5+{\sqrt {5}}\right)}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/92f16397799bd47711ac25e404f6de198c9bb28c)






![{\displaystyle \sin {\frac {2\pi }{15}}=\sin 24^{\circ }={\tfrac {1}{8}}\left[{\sqrt {3}}({\sqrt {5}}+1)-{\sqrt {2}}{\sqrt {5-{\sqrt {5}}}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/748d70aaab6ed6e9d613d6ef799e612174f96970)

![{\displaystyle \tan {\frac {2\pi }{15}}=\tan 24^{\circ }={\tfrac {1}{2}}\left[{\sqrt {2(25+11{\sqrt {5}})}}-{\sqrt {3}}(3+{\sqrt {5}})\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/751f49b7e0a84e36750d6df47e69f7e5ca2f4ba2)


![{\displaystyle \cos {\frac {\pi }{7}}=\cos {\frac {180}{7}}^{\circ }=\cos 25{\frac {5}{7}}^{\circ }={\frac {1}{6}}+{\frac {1-{\sqrt {3}}i}{24}}{\sqrt[{3}]{28-84{\sqrt {3}}i}}+{\frac {1+{\sqrt {3}}i}{24}}{\sqrt[{3}]{28-84{\sqrt {3}}i}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/9e6f0da045735bbe37ae69faa6cb417ce734400d)
![{\displaystyle \sin {\frac {3\pi }{20}}=\sin 27^{\circ }={\tfrac {1}{8}}\left[2{\sqrt {5+{\sqrt {5}}}}-{\sqrt {2}}\;({\sqrt {5}}-1)\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/56f979b3d0d06f3124dd266ee7860dd955e654c1)
![{\displaystyle \cos {\frac {3\pi }{20}}=\cos 27^{\circ }={\tfrac {1}{8}}\left[2{\sqrt {5+{\sqrt {5}}}}+{\sqrt {2}}\;\left({\sqrt {5}}-1\right)\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/96ed3130d2995af89ffd7fed92ca75b79e11b580)








![{\displaystyle \sin {\frac {\pi }{5}}=\sin 36^{\circ }={\tfrac {1}{4}}\left[{\sqrt {2\left(5-{\sqrt {5}}\right)}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/a5a125bab3d72d8e6ca59b000aa0de58afe4e645)




![{\displaystyle \tan {\frac {13\pi }{60}}=\tan 39^{\circ }={\tfrac {1}{4}}\left[\left(2-{\sqrt {3}}\right)\left(3-{\sqrt {5}}\right)-2\right]\left[2-{\sqrt {2\left(5+{\sqrt {5}}\right)}}\right]\,}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/3f5d40feb83e05d4be7784892beb0641f8740e59)















































![{\displaystyle {\frac {1}{2}}{\sqrt {{\frac {1}{3}}\left(7-\omega ^{2}{\sqrt[{3}]{\frac {7+21{\sqrt {-3}}}{2}}}-\omega {\sqrt[{3}]{\frac {7-21{\sqrt {-3}}}{2}}}\right)}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/8cc7dc542d7b26bf260027485f6a30538ffeffed)
![{\displaystyle {\frac {1}{6}}\left(-1+{\sqrt[{3}]{\frac {7+21{\sqrt {-3}}}{2}}}+{\sqrt[{3}]{\frac {7-21{\sqrt {-3}}}{2}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/b9770256721ca5edf98ee18d025cdcea444febf4)

![{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4(-1+{\sqrt {-3}})}}+{\sqrt[{3}]{4(-1-{\sqrt {-3}})}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/559fcd1611f8dce6e41277a61ed398ae11b67dad)




![{\displaystyle {\frac {1}{24}}{\sqrt {3\left(112-{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}-{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}\right)}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/6bcd3c3b7acbdcdc966c3f52fdb381166dd64a02)
![{\displaystyle {\frac {1}{24}}{\sqrt {3\left(80+{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}+{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}\right)}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/7b8523974b4edd2a69791141d1fbcd23955afabe)
![{\displaystyle {\sqrt {\frac {112-{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}-{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}}{80+{\sqrt[{3}]{14336+{\sqrt {-5549064193}}}}+{\sqrt[{3}]{14336-{\sqrt {-5549064193}}}}}}}}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/aac315bbf180a0a43ce39e951361a10dfb9e7d8f)







![{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4{\sqrt {-1}}-4{\sqrt {3}}}}-{\sqrt[{3}]{4{\sqrt {-1}}+4{\sqrt {3}}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/4af28bc5cf3d141c3815a9ca2f3ff7c0a10b3a57)
![{\displaystyle {\frac {1}{4}}\left({\sqrt[{3}]{4+4{\sqrt {-3}}}}+{\sqrt[{3}]{4-4{\sqrt {-3}}}}\right)}](https://wikimedia.org/zhwiki/api/rest_v1/media/math/render/svg/a3ac79b40df411129942e7df1ac3e9d2b805240f)




