跳转到内容

擬詹森多面體：修订间差异

本页使用了标题或全文手工转换
维基百科，自由的百科全书
以互动方式浏览历史
←上一版本下一版本→
删除的内容 添加的内容
可视化wikitext
标签：移除或更換文件
第193行： 第193行：
|  
|  
| ''I'', [5,3]<sup>+</sup><br>order 60
| ''I'', [5,3]<sup>+</sup><br>order 60
|}



{| class="wikitable"
! 名稱
! 圖像
![[頂點圖]]
! V
! E
! F
! F<sub>3</sub>
! F<sub>4</sub>
! F<sub>5</sub>
! F<sub>6</sub>
! F<sub>8</sub>
! F<sub>9</sub>
! F<sub>10</sub>
! [[三維點群|對稱群]]
|-
| [[截角三角化四面體]]
|[[Image:Truncated triakis tetrahedron.png|80px]]
|4 (5.5.5)<BR>24 (5.5.6)
| 28
| 42
| 16
| &nbsp;
| &nbsp;
| 12
| 4
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>
|-
| --
| [[Image:Dh3 symmetry dodecahedral nearmiss johnson.png|80px]]
|6 (5.5.5)<br>9 (3.5.3.5)<br>12 (3.3.5.5)
| 27
| 51
| 26
| 14
| &nbsp;
| 12
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>3h</sub>
|-
| [[四階十二面體]]
|[[Image:Tetrated Dodecahedron.gif|80px]]
|4 (5.5.5)<BR>12 (3.5.3.5)<BR>12 (3.3.5.5)
| 28
| 54
| 28
| 16
| &nbsp;
| 12
| &nbsp;
| &nbsp;
| &nbsp;
| &nbsp;
| ''T''<sub>d</sub>
|-
| --
| [[Image:Hexpenttri near-miss Johnson solid.png|80px]]
|12 (5.5.6)<BR>6 (3.5.3.5)<BR>12 (3.3.5.5)
| 30
| 54
| 26
| 12
| &nbsp;
| 12
| 2
| &nbsp;
| &nbsp;
| &nbsp;
| ''D''<sub>6h</sub>

|}
|}


2023年1月23日 (一) 13:08的版本

在幾何學中，擬詹森多面體是嚴格凸多面體，其面幾乎都是正多邊形，但其中有部分或全部的面不是正多邊形但很接近正多邊形。這種多面體也包含詹森多面體，即所有的面都是正多邊形，而擬詹森多面體經常會有在物理構造沒有注意到的差異在正多邊形與非正多邊形之間[1]。近似的精確值取決於這樣一個多面體的面逼近正多邊形的程度。

例子

名稱
康威多面體表示法		 圖像 頂點布局（英语：Vertex configuration） 頂點 邊 面 F3 F4 F5 F6 F8 F10 F12 對稱性（英语：List of spherical symmetry groups）
底面截角雙三角錐
t4dP3		 2 (5.5.5)
12 (4.5.5)		 14 21 9 3 6 Dih3
order 12
截角三角化四面體
t6kT 		4 (5.5.5)
24 (5.5.6) 		28 42 16     12 4       Td, [3,3]
order 24
五邊形六邊形五角十二面七十四面體 12 (3.5.3.6)
24 (3.3.5.6)
24 (3.3.3.3.5) 		60 132 74 56 12 6 Th, [3+,4]
order 24
倒角立方體
cC 		24 (4.6.6)
8 (6.6.6) 		32 48 18   6   12       Oh, [4,3]
order 48
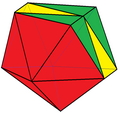
-- 12 (5.5.6)
6 (3.5.3.5)
12 (3.3.5.5) 		30 54 26 12   12 2       D6h, [6,2]
order 24
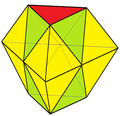
-- 6 (5.5.5)
9 (3.5.3.5)
12 (3.3.5.5) 		27 51 26 14   12         D3h, [3,2]
order 12
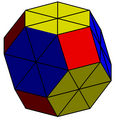
四階十二面體 4 (5.5.5)
12 (3.5.3.5)
12 (3.3.5.5) 		28 54 28 16   12         Td, [3,3]
order 24
部分截半截角八面體 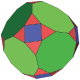24 (3.4.3.9)
24 (3.9.9) 		38 84 48 24 6           Oh, [4,3]
倒角十二面體
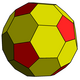
cD 		60 (5.6.6)
20 (6.6.6) 		80 120 42     12 30       Ih, [5,3]
order 120
截半截角二十面體
atI 		60 (3.5.3.6)
30 (3.6.3.6) 		90 180 92 60   12 20       Ih, [5,3]
order 120
截角截角二十面體
ttI 		120 (3.10.12)
60 (3.12.12) 		180 270 92 60         12 20 Ih, [5,3]
order 120
擴展截角二十面體
etI 		60 (3.4.5.4)
120 (3.4.6.4) 		180 360 182 60 90 12 20       Ih, [5,3]
order 120
扭稜截角二十面體
stI 		60 (3.3.3.3.5)
120 (3.3.3.3.6) 		180 450 272 240   12 20       I, [5,3]+
order 60

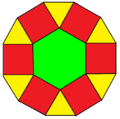
共面擬詹森多面體

有些未能成為詹森多面體的候選多面體是因為其存在有兩個以上共面的面。這些多面體可被看做是凸的面切非常接近正多邊形。

例如： 3.3...

4.4.4.4

3.4.6.4:

  • 正六角帳塔 (退化)
    正六角帳塔
    (退化)

參見

參考文獻

  1. ^ Kaplan, Craig S.; Hart, George W., Symmetrohedra: Polyhedra from Symmetric Placement of Regular Polygons, Bridges: Mathematical Connections in Art, Music and Science (PDF), 2001 [2014-05-01], （原始内容存档 (PDF)于2015-09-23） .
检索自“https://zh.wikipedia.org/zhwiki/w/index.php?title=擬詹森多面體&oldid=75653140”