Talk:Pythagorean theorem/Archive 7
| This is an archive of past discussions about Pythagorean theorem. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. |
| Archive 1 | ← | Archive 5 | Archive 6 | Archive 7 |
Semi-protected edit request on 9 September 2016
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
I think the section Pythagorean_theorem#Sets_of_m-dimensional_objects_in_n-dimensional_space is excessively long, too informal, sometimes ambiguous and sometimes poorly worded. For example, the notion of "objects" is never defined, the use of "x" for the binomial coefficient is non-standard and the statement of the theorem is excessively complicated by multiple objects and parallel planes, even though these don't appear in Conant-Beyer's paper. So I suggest the entire section (including its name) be replaced by the text below.
I previously suggested a similar change to this section, and this was rejected. The comments justifying the rejection are very terse, but I think the only problem was a reference to my own paper in the last sentence. I think the other Wikipedian was being a bit too hard-line about this... I gain very little from this personally, I think the last sentence is quite illuminating about the nature of the Conant-Beyer theorem, and this sentence nicely brings the reader back to the amazing utility of the Pythagorean theorem after a journey through a number of generalizations. But if this is a sticking point then simply delete the last sentence from the text below.
The Conant-Beyer theorem
One of the broadest generalizations of the Pythagorean theorem is due to Donald R. Conant and William A. Beyer, and can be stated as follows.[1]
Let U be a measurable subset of a k-dimensional affine subspace of (so ). For any subset with exactly k elements, let be the orthogonal projection of U onto the linear span of , where and is the standard basis for . Then
where is the k-dimensional volume of U and the sum is over all subsets with exactly k elements.
For example, suppose n = 3, k = 2 and U is the triangle in with vertices A, B and C lying on the -, - and -axes, respectively. The subsets of with exactly 2 elements are , and . By definition, is the orthogonal projection of onto the -plane, so is the triangle with vertices O, B and C, where O is the origin of . Similarly, and , so the Conant-Beyer theorem says
which is de Gua's theorem.
The Conant-Beyer theorem is essentially the inner-product-space version of the Pythagorean theorem applied to the kth exterior power of n-dimensional Euclidean space,[2] so it can be seen as both a generalization and a special case of the Pythagorean theorem.
Jgdowty (talk) 22:01, 9 September 2016 (UTC)
References
- ^
Donald R Conant; William A Beyer (Mar 1974). "Generalized Pythagorean Theorem". The American Mathematical Monthly. 81 (3). Mathematical Association of America: 262–265. doi:10.2307/2319528. JSTOR 2319528.
{{cite journal}}: Invalid|ref=harv(help); Unknown parameter|lastauthoramp=ignored (|name-list-style=suggested) (help) - ^ Lemma 8 and Theorem 9 of James G. Dowty (2014). Volumes of logistic regression models with applications to model selection. arXiv:1408.0881v3 [math.ST ]
![]() Not done. I concur with the above declining of it, but I would also add that compared to what is already there the above is far less approachable, too abstract and obscure for this article. That section is already one of the most advanced section in what is a mostly high-school level article. It does not need rewriting so it is even more advanced.--JohnBlackburnewordsdeeds 23:00, 9 September 2016 (UTC)
Not done. I concur with the above declining of it, but I would also add that compared to what is already there the above is far less approachable, too abstract and obscure for this article. That section is already one of the most advanced section in what is a mostly high-school level article. It does not need rewriting so it is even more advanced.--JohnBlackburnewordsdeeds 23:00, 9 September 2016 (UTC)
- Hi JohnBlackburne, I don't know how to comment on your comment below, sorry, so I'll do it here. I take your point about the version below being more advanced than high school level, but the current version uses all of the same concepts, it just looks less advanced because it is so imprecise (and therefore impenetrable for a different reason). For example, the current version talks about the "m-dimensional coordinate subspace i" without ever defining this, so it looks less complicated than my version, but to actually understand the theorem the reader would have to reconstruct something like my version themselves. Also, you ignored all of my criticisms of the current section, e.g. "the theorem is excessively complicated by multiple objects and parallel planes, even though these don't appear in Conant-Beyer's paper" wp:NOR. So while my changes might not be perfect, they are a lot better than the current version, in my opinion. Lastly, one way to make a precise statement of the theorem more accessible is to add parenthetical comments, but it looks like no-one likes my changes so I won't waste my time on this. — Preceding unsigned comment added by Jgdowty (talk • contribs) 8:35, 10 September 2016 (UTC)
Proposed changes to the section on the Conant-Beyer theorem
Hi everyone,
I'm thinking of replacing the current section on the Conant-Beyer theorem with the section below. I suggested very similar changes in two semi-protected edit requests above, and these were rejected for reasons which are arguably illegitimate (see below), but the mechanism of a semi-protected edit request does not allow a right of reply, as far as I can tell. So I am proposing these changes again here, and I'm hoping that through a full debate, the real merits and deficiencies of my proposal will be revealed.
I think the current section has a number of problems, which should be obvious to anyone who has ever tried to understand it. First, the section relies on a number of notions (e.g. "objects", the "m-dimensional coordinate subspace i") without defining these, which makes it non-rigorous and opaque to anyone who doesn't already understand the theorem. Second, the statement of the theorem involves multiple objects and parallel planes, even though these don't appear in Conant-Beyer's paper. This breaches wp:NOR and also makes the statement cluttered and difficult to follow. Third, the section is overly long (cf. my version) and too informal/imprecise in style for a mathematics article. Fourth, the article uses non-standard notation, such as "x" for the binomial coefficient.
A previous criticism of my proposal only pertains to the last sentence (I think). I don't agree with these criticisms (e.g. how can my result simultaneously contravene wp:primary sources and wp:NOR?) and I think the positives of this sentence outweigh the negatives, as argued here. But in any case, these criticisms are at most an argument for the deletion of the last sentence.
Another criticism of my proposal is that my version is inappropriate because it is more advanced than high school level. See my response to this here.
I don't have a lot of ego invested in my proposal, and I'm happy for it to stand or fall on its merits. I would hope, though, that anyone who contributes to this discussion will have at least read both sections, and that they won't hold my version to a higher standard than the current version. My section isn't perfect, but I think it's clearly superior to the current section.
Regards,
James.
The Conant-Beyer theorem
One of the broadest generalizations of the Pythagorean theorem is due to Donald R. Conant and William A. Beyer, and can be stated as follows.[1]
Let U be a measurable subset of a k-dimensional affine subspace of (so ). For any subset with exactly k elements, let be the orthogonal projection of U onto the linear span of , where and is the standard basis for . Then
where is the k-dimensional volume of U and the sum is over all subsets with exactly k elements.
For example, suppose n = 3, k = 2 and U is the triangle in with vertices A, B and C lying on the -, - and -axes, respectively. The subsets of with exactly 2 elements are , and . By definition, is the orthogonal projection of onto the -plane, so is the triangle with vertices O, B and C, where O is the origin of . Similarly, and , so the Conant-Beyer theorem says
which is de Gua's theorem.
The Conant-Beyer theorem is essentially the inner-product-space version of the Pythagorean theorem applied to the kth exterior power of n-dimensional Euclidean space,[2] so it can be seen as both a generalization and a special case of the Pythagorean theorem.
References
- ^
Donald R Conant; William A Beyer (Mar 1974). "Generalized Pythagorean Theorem". The American Mathematical Monthly. 81 (3). Mathematical Association of America: 262–265. doi:10.2307/2319528. JSTOR 2319528.
{{cite journal}}: Invalid|ref=harv(help); Unknown parameter|lastauthoramp=ignored (|name-list-style=suggested) (help) - ^ Lemma 8 and Theorem 9 of James G. Dowty (2014). Volumes of logistic regression models with applications to model selection. arXiv:1408.0881v3 [math.ST ]
Jgdowty (talk) 02:16, 13 September 2016 (UTC)
 Not done. The very first sentence "One of the broadest generalizations of the Pythagorean theorem is due to Donald R. Conant and William A. Beyer" is original research, and is not allowed—see our policy wp:Original research. Also, still wp:primary sources, wp:UNDUE, wp:PROMO, wp:NOR, wp:COI. When sufficient secondary sources can be found that cite your work, it might become fit to be included in Wikipedia—see wp:secondary sources, wp:DUE, and wp:NOTFORUM. - DVdm (talk) 08:23, 13 September 2016 (UTC)
Not done. The very first sentence "One of the broadest generalizations of the Pythagorean theorem is due to Donald R. Conant and William A. Beyer" is original research, and is not allowed—see our policy wp:Original research. Also, still wp:primary sources, wp:UNDUE, wp:PROMO, wp:NOR, wp:COI. When sufficient secondary sources can be found that cite your work, it might become fit to be included in Wikipedia—see wp:secondary sources, wp:DUE, and wp:NOTFORUM. - DVdm (talk) 08:23, 13 September 2016 (UTC)
- The first sentence is already in the current article version. And all the (potential) conflict with cited policies seem to apply to later sentences (particularly the last ones).--Kmhkmh (talk) 08:58, 13 September 2016 (UTC)
- Thx. Hadn't noticed. In that case the phrase should be tagged. Done. Feel free to tag more. - DVdm (talk) 06:26, 14 September 2016 (UTC)
- The first sentence is already in the current article version. And all the (potential) conflict with cited policies seem to apply to later sentences (particularly the last ones).--Kmhkmh (talk) 08:58, 13 September 2016 (UTC)
- Hi DVdm, can you be more specific in your criticisms? E.g. what parts of the the above section does each criticism refer to, what original research does your second wp:NOR refer to, what competing views does your wp:UNDUE refer to, etc. At the moment your criticisms are too vague to be useful. Also, your "
 Not done" suggests that that you think the current section is superior to the section above... if so then please directly address my criticisms of the current section. Lastly, please try to be constructive in your criticisms whenever possible, e.g. if a minor wording change (such as replacing "one of the broadest generalizations" with "a broad generalization") would address your concern, then just suggest this change. Jgdowty (talk) 23:46, 14 September 2016 (UTC)
Not done" suggests that that you think the current section is superior to the section above... if so then please directly address my criticisms of the current section. Lastly, please try to be constructive in your criticisms whenever possible, e.g. if a minor wording change (such as replacing "one of the broadest generalizations" with "a broad generalization") would address your concern, then just suggest this change. Jgdowty (talk) 23:46, 14 September 2016 (UTC)
- Hi DVdm, can you be more specific in your criticisms? E.g. what parts of the the above section does each criticism refer to, what original research does your second wp:NOR refer to, what competing views does your wp:UNDUE refer to, etc. At the moment your criticisms are too vague to be useful. Also, your "
- See also the comment in the previous section. Sorry, I don't think I can be more specific than I (or we) already have. Perhaps someone else can. - DVdm (talk) 06:34, 15 September 2016 (UTC)
- Are you saying you're not able to identify any specific parts of the proposed section that your criticisms wp:primary sources, wp:UNDUE, wp:PROMO, wp:NOR, wp:COI refer to? Jgdowty (talk) 06:40, 15 September 2016 (UTC)
- No, I am saying that your proposed change in general is not inline with these policies. - DVdm (talk) 07:23, 15 September 2016 (UTC)
- How can the entire section be wp:NOR??? You were able to identify a specific sentence above that was wp:NOR, but you said that wp:NOR applied to other parts of the section. If you can't identify any specific wp:OR then you should withdraw your criticism wp:NOR Jgdowty (talk) 07:33, 15 September 2016 (UTC)
- An entire section can be original research by not appearing anywhere in the literature, except perhaps in your own work. I am pointing out some basic Wikipedia policies here. Please don't take that as criticism. Just trying to help. - DVdm (talk) 08:06, 15 September 2016 (UTC)
- I'm not taking your wp:NOR as a criticism of me, just of the proposed section. So are you saying that the proposed change is wp:NOR because it hasn't been copied verbatim from the literature? But in that case, isn't almost all of Wikipedia's content wp:NOR? Jgdowty (talk) 08:35, 15 September 2016 (UTC)
- DVdm, you've been silent for almost 4 days now, so I think you probably see the absurdity of your claim. So either you didn't understand the policy wp:NOR, despite your experience and your self-appointed role as policy police, or you willfully misrepresented the policy to try to force an outcome to the detriment of Wikipedia. I'll let everyone make up their own minds about which possibility is more likely. So unless you are still claiming that almost all of Wikipedia is in breach of wp:NOR, then I'll consider your "in general" wp:NOR to be withdrawn. Jgdowty (talk) 00:40, 19 September 2016 (UTC)
- That would be a bad idea. I have nothing to add to the preceding comments (by myself and by others). Sorry for that. - DVdm (talk) 06:01, 19 September 2016 (UTC)
- Yes, I don't know about you, but I always find this sort of "if you don't keep repeating your opposition over and over I'm going to assume that you've changed your mind" tactic really irritating and counterproductive as a way of changing minds. (I agree btw that this proposed change is far too WP:TECHNICAL for this article, regardless of whether the sources can be found to justify it being non-OR.) —David Eppstein (talk) 06:23, 19 September 2016 (UTC)
- I'm not asking DVdm to repeat him- or herself, I'm just asking for basic information about his or her criticisms, without which it is impossible to address any of them. The statement of the theorem above is just a more explicit version of the statement in Conant and Beyer's paper, so the reference is their paper (as already cited in the proposed section). On the idea that the proposed change is too WP:TECHNICAL, see my previous comment. Or do you think the Conant-Beyer theorem itself is too advanced for Pythagorean_theorem, so the current section should simply be deleted? Jgdowty (talk) 07:33, 19 September 2016 (UTC)
- Yes, I don't know about you, but I always find this sort of "if you don't keep repeating your opposition over and over I'm going to assume that you've changed your mind" tactic really irritating and counterproductive as a way of changing minds. (I agree btw that this proposed change is far too WP:TECHNICAL for this article, regardless of whether the sources can be found to justify it being non-OR.) —David Eppstein (talk) 06:23, 19 September 2016 (UTC)
- That would be a bad idea. I have nothing to add to the preceding comments (by myself and by others). Sorry for that. - DVdm (talk) 06:01, 19 September 2016 (UTC)
- An entire section can be original research by not appearing anywhere in the literature, except perhaps in your own work. I am pointing out some basic Wikipedia policies here. Please don't take that as criticism. Just trying to help. - DVdm (talk) 08:06, 15 September 2016 (UTC)
- How can the entire section be wp:NOR??? You were able to identify a specific sentence above that was wp:NOR, but you said that wp:NOR applied to other parts of the section. If you can't identify any specific wp:OR then you should withdraw your criticism wp:NOR Jgdowty (talk) 07:33, 15 September 2016 (UTC)
- No, I am saying that your proposed change in general is not inline with these policies. - DVdm (talk) 07:23, 15 September 2016 (UTC)
- Are you saying you're not able to identify any specific parts of the proposed section that your criticisms wp:primary sources, wp:UNDUE, wp:PROMO, wp:NOR, wp:COI refer to? Jgdowty (talk) 06:40, 15 September 2016 (UTC)
- To sum up, I've now shown the absurdity of one of DVdm's criticisms, so he or she is refusing to discuss them any further. In an authentic intellectual environment, this sort of childish behaviour would not be tolerated, and DVdm would have to either defend his or her criticisms or withdraw them. But David Eppstein thinks DVdm's behaviour is just fine. Fortunately, I don't really know what motivates someone to act in the way that DVdm has here, but it's clear that rationality and improving Wikipedia aren't high on the list.
- Does anyone else have any comments? In particular, do you think the current section is inferior or superior to the proposed section? Jgdowty (talk) 23:45, 19 September 2016 (UTC)
- I'm not "anyone else", but I'll just note that you have failed to convince me that the added technicality of your proposed version (the fact that it is more technical should be obvious to all) has any matching benefit. But I'd like to address a different point: whether your changes are original research. I haven't determined for myself whether they are or are not, but the fact that you want to cite an unpublished arXiv preprint is a big red flag. Because they are not peer-reviewed, arXiv papers generally do not count as reliable sources, so if you are depending on this preprint for some information that was not already in the Conant–Beyer reference then it would indeed be original research. On the other hand, if the preprint does not add any information over the Conant–Beyer reference then why do you want to cite it? —David Eppstein (talk) 01:15, 20 September 2016 (UTC)
- All of this has already been covered, e.g. see my pre-amble before the proposed section. On your first point, the current section looks less technical because it is incomplete, so it is not understandable to anyone who doesn't independently know what the author means by, for example, the "m-dimensional coordinate subspace i". On your second point, I think the positives of the last sentence outweigh the negatives, but I've already said that if this is a sticking point then we should just delete the last sentence. I only opposed DVdm's claim above that the entire proposed section is WP:NOR because it hasn't been copied verbatim from a textbook. When I pointed out that this would mean that almost all of Wikipedia is WP:NOR, and asked DVdm to withdraw this absurd "in general" WP:NOR, you described my protest as "really irritating". But none of this is my problem anymore, I have better things to do with my time. So I'll leave you and DVdm all by yourselves to enjoy the intellectual desert that you've created here. Jgdowty (talk) 23:33, 20 September 2016 (UTC)
- I'm not "anyone else", but I'll just note that you have failed to convince me that the added technicality of your proposed version (the fact that it is more technical should be obvious to all) has any matching benefit. But I'd like to address a different point: whether your changes are original research. I haven't determined for myself whether they are or are not, but the fact that you want to cite an unpublished arXiv preprint is a big red flag. Because they are not peer-reviewed, arXiv papers generally do not count as reliable sources, so if you are depending on this preprint for some information that was not already in the Conant–Beyer reference then it would indeed be original research. On the other hand, if the preprint does not add any information over the Conant–Beyer reference then why do you want to cite it? —David Eppstein (talk) 01:15, 20 September 2016 (UTC)
- I've decided to not proceed with the proposed changes above. Anyone else who wants to is free to make the changes themselves, of course. As a reminder, here are my criticisms of the current section. First, the section relies on a number of notions (e.g. "objects", the "m-dimensional coordinate subspace i") without defining these, which makes it non-rigorous and opaque to anyone who doesn't already understand the theorem. Second, the statement of the theorem involves multiple objects and parallel planes, even though these don't appear in Conant-Beyer's paper. This breaches wp:NOR and also makes the statement cluttered and difficult to follow. Third, the section is overly long (cf. my version) and too informal/imprecise in style for a mathematics article. Fourth, the article uses non-standard notation, such as "x" for the binomial coefficient. Jgdowty (talk) 23:33, 20 September 2016 (UTC)
- Thanks for your de-facto constructive attitude. You have learned that Wikipedia is all about wp:consensus. - DVdm (talk) 06:33, 21 September 2016 (UTC)
Matrix version
The article doesn't include the n-dimensional equivalent, which is important in many numerical applications: given positive (semi-)definite matrices which fulfill , and given the matrix square roots (where a square root of a positive (semi-)definite matrix is a lower-triagonal matrix such that ) one can find the matrix square root of based on the n-dimensional pythagorean theorem as follows: first, we observe that , for some orthogonal matrix , i.e. , and therefore . The calculation then proceeds by performing a QR-decomposition of , i.e. where the upper square part of the right-hand side matrix is actually upper-triangular and the transpose of the sought-for square-root, .
The relation to the one-dimensional case can be established as follows: for scalar , we want to find such that holds. Given the above we therefore aim to find a orthogonal matrix and such that the equation holds, and thus find .
This procedure finds applications e.g. in the rank-one update of a Cholesky decomposition (where the lower-triangular matrix is composed of a vector in the first column and zeros elsewhere, which allows further simplifications of the calculation). Another application is the usual error-averaging formula for the covariance matrix of the weighted average of two multi-Gaussian distributed measurements with covariance matrices . Expressing this directly in terms of the square roots avoids the intermediate Cholesky decompositions for the calculations of the inverses, replacing them with the forward substitution and QR decomposition, simultaneously doubling the numerical range while ensuring positive-definiteness of the result even in presence of numerical inaccuracies. — Preceding unsigned comment added by 240D:2:551E:A500:F499:8B48:A2D7:5EF2 (talk) 07:14, 19 December 2017 (UTC)
In popular culture section
Is any of the In popular culture section of the article policy-compliant? It seems to me like none of the entries are substantial facts about public knowledge of the theorem. A section on the theorem's position in pedagogy, or statistics on how many people can recall (prove, understand) the theorem might be useful. But a collection of places where the theorem appears in songs or jokes (regardless of whether high culture like Brief Lives or low culture like The Simpsons) doesn't seem to aid understanding of anything relating to the subject.
I'll remove the section boldly if this doesn't get any replies, but as this is a good article, I thought I should post here first. — Bilorv(c)(talk) 15:47, 16 June 2018 (UTC)
- Okay, I've removed the section in this edit. — Bilorv(c)(talk) 20:58, 22 June 2018 (UTC)
A more economical proof using the dissection approach
I suggest to add the following proof, which is more economical comparing to the current proof in this page. The proof below disintegrates the rectangles into 5 pieces, while the current has 7 pieces (the figure with this text below: Proof using an elaborate rearrangement).
This is the suggested proof:
https://drive.google.com/open?id=19A79SXUQP2YrDRGg4X_kGRSiuUxnpwfH
Thanks
Valery
Vk1988 (talk) 13:17, 29 July 2018 (UTC)
Equation error
The first equation under “Algebraic Proofs” seems wrong: Currently it is (b-a)^2 +4(ab) = (b-a)^2 (^2 means squared) The right-hand side should be “c-squared” or c^2 , otherwise the equation results in “ab=0” BillBaity (talk) 17:02, 2 January 2019 (UTC)
- I do not see where you get this from. The first equation in that section is
- (b − a)2 + 4ab/2 = a2 + b2
- which is perfectly correct. --Bill Cherowitzo (talk) 23:18, 2 January 2019 (UTC)
History of Pythagorean theorem
I think History of Pythagorean theorem should be shown first rather than proofs and other things -IndianEditor (talk) 12:01, 4 September 2017 (UTC)
- I.m.o. the mathematical content of this article is more important than the historical content. - DVdm (talk) 12:11, 4 September 2017 (UTC)
- I concur with DVdm. This article is about an important mathematical result. The particulars of who discovered it first is just a minor aspect that does not increase anyone's understanding of the result. --Bill Cherowitzo (talk) 16:43, 4 September 2017 (UTC)
- "Evidence from megalithic monuments in Northern Europe shows that such triples were known before the discovery of writing." RefNec but the page is protected.80.72.90.183 (talk) 20:00, 5 September 2017 (UTC)
- Good catch, thanks. I removed it as unsourced but if a sufficiently reliable source can be turned up it can be added back in a more appropriate point of the article. —David Eppstein (talk) 22:45, 5 September 2017 (UTC)
- One place such a claim is made is in the book Geometry and Algebra in Ancient Civilizations by B. L. van der Waerden. He refers to the books Sun, Moon and Standing Stones by J. E. Wood and Megalithic Remains in Britain and Brittany by A. Thom and A. S. Thom, but, to judge by J. Høyrup's Zentralblatt review, draws conclusions beyond what is stated in those sources, specifically that the henge builders in Britain and France had theoretical understanding of Pythagorean triples. Even the Thoms' milder claim that the henge builders aimed for right triangles with integer side lengths in their constructions—for example, that they constructed true ellipses in which the right triangle formed by the focal distance, the semi-minor axis, and the semi-major axis was a 3-4-5, 5-12-13, or 12-35-37 triangle—would seem to rely on the soundness of the statistical analyses that led to the hypothesis of the megalithic yard. The linked Wikipedia articles on Alexander Thom and on the megalithic yard both contain discussions of the scholarly reaction to that hypothesis. My personal, not-so-well-informed feeling is that the skeptics are likely right, and that this may all be too controversial for Wikipedia. Will Orrick (talk) 19:05, 13 January 2019 (UTC)
- Good catch, thanks. I removed it as unsourced but if a sufficiently reliable source can be turned up it can be added back in a more appropriate point of the article. —David Eppstein (talk) 22:45, 5 September 2017 (UTC)
- "Evidence from megalithic monuments in Northern Europe shows that such triples were known before the discovery of writing." RefNec but the page is protected.80.72.90.183 (talk) 20:00, 5 September 2017 (UTC)
- The history paragraph is simply awful. It clearly written by someone with the the pre-determined goal of defending Pythagoras as the deserved name of the theorem rather than being objective. It says "it is he who, by tradition, is credited with its first recorded proof". There is NO recorded proof. The writer slaps on 3 sources that do not even support this. The Heath source clearly states, "no really trustworthy evidence exists that it was discovered by him" in the first line! This is too much of a mess to fix: See Brittanica for an example of what it should look like. — Preceding unsigned comment added by JPKowal (talk • contribs) 20:23, 21 April 2018 (UTC)
Einstein's proof
Imho could the proof description could be a bit more concrete/explicit and probably use a better graphic illustration. Also following the description in the link below, it is not quite clear whether this is Einstein's (published) proof or not, although it seems to be the most likely candidate. The article should make that clear rather than giving it as a (cited) fact.
--Kmhkmh (talk) 23:45, 31 March 2019 (UTC)
Pythagorean proof
It is problematic to have a section called "Pythagorean proof". For one thing, the assertion that Pythagoras had a proof is already very controversial. Even among those who believe he did have a proof, there is great disagreement about what that proof might have been.
In the last paragraph of the section we say, "That Pythagoras originated this very simple proof is sometimes inferred from the writings of the later Greek philosopher and mathematician Proclus", and Maor is cited. I don't know where in Proclus this is supposed to come from, but the opening lines of Proclus's commentary on Proposition 47 of Book I of Elements (the Pythagorean theorem) say (in Glenn R. Morrow's translation),
If we listen to those who like to record antiquities, we shall find them attributing this theorem to Pythagoras and saying that he sacrificed an ox on its discovery. For my part, though I marvel at those who first noted the truth of this theorem, I admire more the author of the Elements, not only for the very lucid proof by which he made it fast, but also because in the sixth book he laid hold of a theorem even more general than this and secured it by irrefutable scientific arguments.
Scholars disagree on what Proclus is saying here about the attribution of either the statement or the proof of the theorem to Pythagoras, but one common interpretation is that he is willing to attribute at most the statement to Pythagoras. At any rate, he says nothing here, or in the rest of the commentary on Proposition 47, about a Pythagorean proof. Perhaps Maor is referring to some other passage in Proclus, but I don't know of any passage in Proclus that hints at such a proof.
It's possible that Maor is simply mistaken. What he says is, "It is from Proclus’s commentary that we may infer a possible proof of the Pythagorean theorem by Pythagoras, namely a proof by dissection". He then has a paragraph of indented text sketching the rearrangement proof, followed by a citation to pages 80–81 of the book, An Introduction to the History of Mathematics by Howard Eves. I believe he is citing the 6th edition. The indented paragraph is an abbreviated paraphrase of what's in Eves's book, but the statement about Proclus does not seem to appear in Eves's account.
Heath in his 1905 translation of Euclid's Elements, does add extensive speculation about possible Pythagorean proofs, and discusses the Proclus commentary quoted above. But none of Heath's speculation suggests that a Pythagorean proof can be inferred from Proclus's commentary. Our dissection proof is even shown in Heath's text, and the idea that Pythagoras might have used such a proof is attributed by Heath to "Bretschneider, followed by Hankel". Heath, however favors a different proof as the "Pythagorean" one. All this makes me wonder whether Maor simply misread or misremembered something in Heath's commentary as deriving from Proclus. Otherwise I cannot account for Maor's statement.
If Maor is the only source stating that this proof can be inferred from Proclus, then I think we ought to remove that statement as it doesn't seem to be supported by any real evidence. But more importantly, we need to make it clear that any "Pythagorean" proof is a modern imagining, and that Pythagoras may not have had a proof at all. Furthermore, there is a considerable body of scholarly work over the past several decades that casts doubt on the notion that Pythagoras made any contributions to mathematics, science, or rational philosophy. Much better attested are his establishment of a Pythagorean way of life and his doctrines on the afterlife. Will Orrick (talk) 20:01, 23 April 2019 (UTC)
- I have gone ahead and removed the assertion that something in Proclus implies this proof and a connection with Pythagoras. While I have not entirely removed all mention of the proposal that Pythagoras might have had this proof, I have rewritten the section to emphasize that this is speculative and not widely accepted. I have also retitled the section. Will Orrick (talk) 03:17, 7 June 2019 (UTC)
Rearrangement proof requires triangle postulate?
It is mentioned that the proof using similar triangles requires the triangle postulate. Doesn't the rearrangement proof also require the triangle postulate, in order to prove that the shape marked is in fact square? Grover cleveland (talk) 21:47, 10 January 2021 (UTC)
- Yes, I believe that is correct. Either there is a direct application of the postulate, evident in each corner of the final arrangement and where the three angles sum to 180 degrees, or by means of symmetry this rearrangement proves the postulate. Whichever the case, it seems the postulate is assumed. ChicoB83 (talk) 14:18, 4 February 2021 (UTC)
Proofs different in nature
Isn't there a fundamental difference between Euclid's and Einstein's proofs of the theorem? Euclid's proof, purely geometric, regards the equality of areas (a measure of surface extent) of squares built on the sides of a right triangle, regardless of any way of determining the areas themselves as a function of the length of their sides. Einstein's proof, on the other hand, is purely algebraic and regards the length (a measure of linear extent) of the sides of a right triangle, and need no mention of any measure of areas. In fact, one does not need to know the area of a square in terms of the length of its sides to accept Euclid's proof, or Einstein's proof for that matter, for they are simply different in nature. Shouldn't this be mentioned somewhere in the article? ChicoB83 (talk) 23:58, 18 November 2020 (UTC)
- Only if this distinction, between these two proofs out of hundreds or thousands, can be supported by reliable published sources. —David Eppstein (talk) 00:03, 19 November 2020 (UTC)
- This distinction stems only from the fact that one does not need to know the algebraic formula of the "sums of the squares" in order to prove the Pythagorean Theorem. This is an important aspect, since it depends on what came first: the geometric theory of area or the algebraic (as mentioned in the Area#Rectangles article. If the algebraic theory takes precedence, then the Einstein proof proves the Pythagorean Theorem. If not, one needs an extra axiom that allows for the computation of the area of a rectangle in order to state the "sum of squares" formulation. I understand your reply, and I believe it would be fairly easy to find reliable sources on this. My question was not so much whether this could be mentioned, but rather if this mention is pertinent. ChicoB83 (talk) 19:02, 9 February 2021 (UTC)
Semi-protected edit request on 28 June 2021
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
2600:1015:B143:8D54:6BE5:B3:A1B1:F7E6 (talk) 22:49, 28 June 2021 (UTC)
This needs to be changed to Pythagorean Law.
 Not done for now: please establish a consensus for this alteration before using the
Not done for now: please establish a consensus for this alteration before using the {{edit semi-protected}}template. ScottishFinnishRadish (talk) 23:00, 28 June 2021 (UTC)
Semi-protected edit request on 24 August 2021
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
Please allow me to edit 🙏🙏🙏🙏🙏🙏🙏🙏🙏🙏🙏 No one seriously (talk) 14:29, 24 August 2021 (UTC)
 Not done: requests for decreases to the page protection level should be directed to the protecting admin or to Wikipedia:Requests for page protection if the protecting admin is not active or has declined the request. ScottishFinnishRadish (talk) 14:48, 24 August 2021 (UTC)
Not done: requests for decreases to the page protection level should be directed to the protecting admin or to Wikipedia:Requests for page protection if the protecting admin is not active or has declined the request. ScottishFinnishRadish (talk) 14:48, 24 August 2021 (UTC)
Please allow me to edit No one seriously (talk) 05:57, 27 August 2021 (UTC)
Allow me to edit No one seriously (talk) 05:57, 27 August 2021 (UTC)
Allow me to edit No one seriously (talk) 05:57, 27 August 2021 (UTC)
I promise I'll not make any offensive edits in this page No one seriously (talk) 05:58, 27 August 2021 (UTC)
- You are gunning for a WP:NOTHERE block, unless someone recognizes you as a banned user and blocks you for that. You need to demonstrate that you can edit seriously before people will take such requests seriously. —David Eppstein (talk) 06:19, 27 August 2021 (UTC)
Si. 427
Another user cited The Guardian to include the discovery of Si. 427, and that wasn't considered a good enough source, but an article released on Physorg by the University of New South Wales Math department should be considered authoritative on the subject. I hope that source is more acceptable. CessnaMan1989 (talk) 15:40, 12 September 2021 (UTC)
- This is not an academic paper, it is an advertising announcement issued by the staff of a university. So this is far from a reliable source for an scientific subject. Moreover, this refers to a "mathematician", not a historian. For the interpretation of this cuneiform tablet, skills in elementary mathematics are needed, but this is not sufficient, as this is a work of specialists of cuneiform tablets. As apparently no such specialist is implied, this interpretation by a mathematician must be taken with great care. So, I have reverted the article edit. D.Lazard (talk) 16:17, 12 September 2021 (UTC)
- No, it's a university announcement, and the fact that it refers to a mathematician doesn't diminish its credibility. CessnaMan1989 (talk) 03:33, 1 October 2021 (UTC)
Semi-protected edit request on 23 October 2021
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
BlackDragon450 (talk) 22:43, 23 October 2021 (UTC)
Edit Request
 Not done: it's not clear what changes you want to be made. Please mention the specific changes in a "change X to Y" format and provide a reliable source if appropriate. Cannolis (talk) 22:48, 23 October 2021 (UTC)
Not done: it's not clear what changes you want to be made. Please mention the specific changes in a "change X to Y" format and provide a reliable source if appropriate. Cannolis (talk) 22:48, 23 October 2021 (UTC)
Semi-protected edit request on 6 December 2021
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
Change reference 10 "Elements 1.47 by Euclid. Retrieved 19 December 2006." used in Euclid's Proof section to another source, the link https://www.perseus.tufts.edu/cgi-bin/ptext?doc=Perseus:text:1999.01.0085:book=1:proposition=47 is broken. https://farside.ph.utexas.edu/Books/Euclid/Elements.pdf Seems to work fine. WhalingFish (talk) 11:16, 6 December 2021 (UTC)
 Done I have removed the dead link and added the suggested one. The format of the citation requires still to be fixed. D.Lazard (talk) 11:46, 6 December 2021 (UTC)
Done I have removed the dead link and added the suggested one. The format of the citation requires still to be fixed. D.Lazard (talk) 11:46, 6 December 2021 (UTC)
- I cleaned-up two citations. They may not be perfect now, but I think they are clearer.—Anita5192 (talk) 16:41, 6 December 2021 (UTC)
- Thanks. D.Lazard (talk) 17:35, 6 December 2021 (UTC)
- I cleaned-up two citations. They may not be perfect now, but I think they are clearer.—Anita5192 (talk) 16:41, 6 December 2021 (UTC)
Semi-protected edit request on 30 March 2022
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
Under the section "Rearrangement Proofs"
Change "This tringle will have an area of both" to "This square will have an area of both" SebastienSiva (talk) 00:35, 30 March 2022 (UTC)
 Done with a slight clarification of the sentence. Further edits would be welcome to clarify further the whole paragraph. D.Lazard (talk) 08:17, 30 March 2022 (UTC)
Done with a slight clarification of the sentence. Further edits would be welcome to clarify further the whole paragraph. D.Lazard (talk) 08:17, 30 March 2022 (UTC)
Semi-protected edit request on May 25 2022
This edit request to Pythagorean theorem has been answered. Set the |answered= or |ans= parameter to no to reactivate your request. |
Under the section "Similar figures on the three sides"
This sentence is ungrammatical / confused:
"The underlying notion is area formulas for any plane figure a proportionate to a length squared."
Possibly this sentence should be:
"The underlying notion is area formulas for any plane figure are proportionate to a length squared."
But I am not sure if that would make it correct... can a formula really be "proportionate"... only the terms in the formula could be proportional, surely? Maybe the sentence should be:
"The underlying notion is that the area for any plane figure is proportional to a length squared."
although in this latter case it is still not clear what "a length" refers to. Another possibility is:
"The underlying notion is that the area for any plane figure scales according to the square of its length, perimeter, or diameter."
Or possibly the sentence can just be cut.
- I just deleted it. There's a similar, but more grammatical sentence just two paragraphs later. Will Orrick (talk) 22:59, 25 May 2022 (UTC)
Pythagorean tiling
Would it be worth trying to add a section after Pythagorean theorem § Proofs by dissection and rearrangement for Pythagorean tiling? I always felt such tilings were one of the best ways to viscerally understand the Pythagorean theorem, and many proofs by dissection can be thought of as representing one c-squared tile from such a tiling. –jacobolus (t) 02:29, 22 November 2022 (UTC)
Simplifying the Lede
@Sugeeth_Jayara changes were reverted, but I think that there is merit in simplifying the lede.
Currently "In mathematics, the Pythagorean theorem, or Pythagoras' theorem, is a fundamental relation in Euclidean geometry among the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the legs a, b and the hypotenuse c, often called the Pythagorean equation:"
Euclid's Propositions for reference)
Book VI, Proposition 31:
In right-angled triangles the figure on the side opposite the right angle equals the sum of the similar and similarly described figures on the sides containing the right angle."
'Book I, Proposition 47:
In right-angled triangles the square on the side opposite the right angle equals the sum of the squares on the sides containing the right angle."
(wording from https://link.springer.com/article/10.1057/jt.2009.16
Issues
- Why specify "In mathematics" ? It implies that there is another domain with a different usage.
- In the lede, we should stick with common terms used in schools - square rather than area, side rather rather than legs/catheti
- fundamental relation - Is this the correct phrase? Euclid had axioms and notions, Are there non-Euclidean geometries, except for the modified version for spherical, where the theorem is true?
- We describe it in the lede as a theorem, a relation, and an equation (And as it's Euclidean, possibly imply a proposition as well :-).)
- I prefer bracketing the alternate name rather than commas( similar to also known for a person)
- Euclidean geometry - there is a version of it for spherical geometries. Are there variants in other non= Euclidean geometries?
- Is it between or among? a triangle definitely has a 'tween area
- more links are needed, e.g. https://en.wikipedia.org/wiki/Right_triangle
Suggested"
The Pythagorean (Pythagoras') theorem states that for right triangles the square of the hypotenuse (the side opposite the right angle) is equal to the square of the other two sides. In algebraic form this is called the Pythagorean Equation and is written
a^2+b^2=c^2.
where c is the hypotenuse, and a or b are the other two sides. This holds true for Euclidean Geometry, and modified versions of this equation are used in spherical geometry. Wakelamp d[@-@]b (talk) 10:56, 14 November 2022 (UTC)
- As "theorem" is used in mathematics, readers are not supposed to know that this is mathematics.
- The "area of a square" is commonly taught at elementary level; for the use of "leg", and the link to "cathetus", I have removed them.
- Euclid did not have axioms, but postulates, and had also theorems, and many other meta concepts. In any case, this is a relation, and it is fundamental.
- An equation is special type of relation, and the truth of this relation is a theorem.
- I agree that the commas are not needed, and I have removed them.
- Possible extensions to non-Euclidean geometries are far too technical for the lead.
- Right triangle is already linked.
- D.Lazard (talk) 11:45, 14 November 2022 (UTC)
- As "theorem" is used in mathematics, readers are
notsupposed to know that this is mathematics. SO I Assume not was accidental - The "area of a square" is commonly taught at elementary level; for the use of "leg", and the link to "cathetus", I have removed them. QUESTION is leg commonly used in the United States?
- Euclid did not have axioms, but postulates, and had also theorems, and many other meta concepts. In any case, this is a relation, and it is fundamental. BUT I agree that area of the square is elementary, however it is not Euclid's formulation or the common wording (at least in Australian education). The Euclidean_geometry article refers to axioms. Still uncertain what a fundamental theorem is/is not, With cathetus, it should be mentioned, later on in the article. Is leg commonly used
- An equation is special type of relation, and the truth of this relation is a theorem. BUT I am uncertain of the wording that the truth of a relation has to be a theorem AND the split is beyond the knowledge of a general reader
I agree that the commas are not needed, and I have removed them.- Possible extensions to non-Euclidean geometries are far too technical for the lead. SO I reluctantly agree, but it is such a nice extension. The article does however have sections on non-Euclidean geometry, and other case (3D and non-right triangles. so the Euclidean geometry should be mentioned later just before the equation.
Right triangle is already linked.Wakelamp d[@-@]b (talk) 21:48, 14 November 2022 (UTC)- Readers who already know what “theorem” is might know that this is mathematics. In the event some readers don’t already know what a theorem is, then in theory the context of of 'in mathematics' and a wiki-link could be helpful (there is also of course a wiki-link to ‘theorem’). A reasonable argument could be made that an initial "in mathematics, ..." could be dropped from many Wikipedia math articles without much loss. –jacobolus (t) 01:55, 15 November 2022 (UTC)
- I think editors of mathematics articles start articles this way because the Manual of Style tells them to. I just clicked on the "Random article" link twenty times and discovered that in no other field do editors do this, and their articles are enjoyable to read, while still being clear. Will Orrick (talk) 02:17, 15 November 2022 (UTC)
is leg commonly used in the United States?
From some quick literature searches it seems like the name 'leg' for one of the two shorter sides of a right triangle is used/understood in many parts of the world, at least when writing in English. YMMV. –jacobolus (t) 01:55, 15 November 2022 (UTC)[area of squares] is not Euclid’s formulation or the common wording (at least in Australian education)
– what do you think it means to sum two geometric squares? What do they say in Australia? Talking about the areas of two-dimensional shapes seems to me like the nearest modern phrasing of the Euclidean concept (to use Euclid’s text you need to introduce a lot of additional concepts which are no longer in currency); a more modern conceptual approach (since maybe the 18th century) would be to talk about the distance squared as a number per se. –jacobolus (t) 01:55, 15 November 2022 (UTC)- I agree that Euclid's descriptions are very complicated viewed by modern eyes, as we are so used to algebra and we don't think in terms of the shapes and terms he describes. Randomly browsing through google books, I think the current wording of "the area of square of the hypotenuse" seems to refer to diagrams such as the article, rather than to the theorem.
- BTW "What do they say in Australia? G'day, :-) Wakelamp d[@-@]b (talk) 07:58, 16 November 2022 (UTC)
- There's no single authority who can say how the theorem should be worded. The connection between the square of a length and the area of a square with side of that length is immediate, so the two statements of the theorem are equivalent. For most of human history, the statement in terms of area was the one used. This is true of the statement in the Sulbasūtras, which is very direct, and most likely in the Chinese and Babylonian versions as well, although one has to do a bit of reading between the lines in those cases. The Greek situation is somewhat special, as Euclid completely avoided the notions of length and area. But again, Euclid's statement is about geometric squares, treated as what Euclid's system refers to as "magnitudes". And it's not so much that Euclid's formulation is complicated as that his usage of words is unfamiliar to people educated in the modern way.
- Which formulation would be clearest to the typical Wikipedia reader is an empirical question that I don't think can be settled by debate between editors. It would be nice to get more feedback on this question. I do, however, think we would be remiss not to include the area formulation very close to the beginning of the article. In addition to its historical resonance, it is needed to follow many of the proofs given in the article. Will Orrick (talk) 16:45, 18 November 2022 (UTC)
- Avoiding the google search option, would checking the stats for google books/scholar, or the most popular textbooks/syllabus for the syllabus be acceptable? Wakelamp d[@-@]b (talk) 15:50, 21 November 2022 (UTC)
- What stats? In terms of sheer numbers, the majority of books that contain a statement of the Pythagorean theorem will be middle school textbooks, followed by popular expositions of mathematics. The theorem is so deeply enmeshed in the fabric of mathematics that no research-level mathematics book or paper is going to contain an explicit statement of it. If you do find a statement of the theorem on Google Scholar it will likely be either a work on history of mathematics or on mathematics pedagogy. I'm not sure what a majority vote among such disparate sources is going to tell us. We should weight sources according to how closely their goals align with what we are trying to accomplish in this article. I also believe in using our own editorial judgement.
- In trying your experiment, I did run across
- Chambers, P. (1999). Teaching Pythagoras’ Theorem. Mathematics in School, 28(4), 22–24. [1].
- You will need a (free) jstor account to read past the first page, but the first page already contains an in-depth discussion of the issues that concern you. Will Orrick (talk) 03:56, 22 November 2022 (UTC)
- Avoiding the google search option, would checking the stats for google books/scholar, or the most popular textbooks/syllabus for the syllabus be acceptable? Wakelamp d[@-@]b (talk) 15:50, 21 November 2022 (UTC)
- Readers who already know what “theorem” is might know that this is mathematics. In the event some readers don’t already know what a theorem is, then in theory the context of of 'in mathematics' and a wiki-link could be helpful (there is also of course a wiki-link to ‘theorem’). A reasonable argument could be made that an initial "in mathematics, ..." could be dropped from many Wikipedia math articles without much loss. –jacobolus (t) 01:55, 15 November 2022 (UTC)
- As "theorem" is used in mathematics, readers are
- In Euclid it is the convention that "adding squares" means adding their areas, or perhaps cutting them into pieces and rearranging them (see dissection problem). The modern way to express this is as area addition. –jacobolus (t) 18:57, 14 November 2022 (UTC)
Should the converse (that implies is a right angle) perhaps be mentioned in the lead section? –jacobolus (t) 18:59, 14 November 2022 (UTC)
- And while we are at it, I really don’t like the phrasing of the converse in the relevant section:
For any three positive numbers a, b, and c such that a² + b² = c², there exists a triangle with sides a, b and c, and every such triangle has a right angle between the sides of lengths a and b.
And alternatelyFor any triangle with sides a, b, c, if a² + b² = c², then the angle between a and b measures 90°.
- I think better than either would be to say:
For any triangle with side lengths {a, b, c}, if a² + b² = c², then the angle opposite side c is a right angle.
Angle measure per se is unnecessary here (and not found in Euclid), and the business about "there exists a triangle" is true but overcomplicated. The existence of a triangle from such lengths (guaranteed for the much broader class of lengths satisfying the triangle inequality) seems like a separate topic. –jacobolus (t) 19:06, 14 November 2022 (UTC)- Okay, I made this change. Does that seem okay to everyone? –jacobolus (t) 20:36, 18 November 2022 (UTC)
- Overall I think the more concise formulation is better, but it seems to belabor the point to have an additional paragraph discussing the "generalization". One could just have a single sentence noting that the hypothesis that the triangle exists can be omitted since existence follows from the converse of the triangle inequality. Will Orrick (talk) 22:12, 18 November 2022 (UTC)
- Please feel free to write that sentence. :-) –jacobolus (t) 17:57, 21 November 2022 (UTC)
- Overall I think the more concise formulation is better, but it seems to belabor the point to have an additional paragraph discussing the "generalization". One could just have a single sentence noting that the hypothesis that the triangle exists can be omitted since existence follows from the converse of the triangle inequality. Will Orrick (talk) 22:12, 18 November 2022 (UTC)
- Okay, I made this change. Does that seem okay to everyone? –jacobolus (t) 20:36, 18 November 2022 (UTC)
To add some more info about pythagorean theorem
Let's add some credit and info regarding Indian mathematician Budhayan who mentioned and shown in his text about the exact theorem way prior than Phythagorus. This edit wouldn't affect any credits to Pythagorus, it would be fair if we mention about those mathematician who already knew about the pythagorean theorem prior to him. Just an request to mention name of Budhayan in this article without affecting Pythagorus. Thanks. Monnagaur99299 (talk) 03:01, 22 December 2022 (UTC)
- Did you read the section Pythagorean theorem § History? It talks about Baudhayana. –jacobolus (t) 06:18, 22 December 2022 (UTC)
Edit Request - Typography correction
Refer to the second set of equations in the section about "Other Proofs - Proofs Using Similiar Triangles". The left hand term of each equation is incorrect/confusing. BC^2 is usually interpreted as "B times C-squared" but in this context what is wanted is "the square of the length of line segment BC". I realize that this section uses explicit multiplication symbols rather than multiplication-implied-by-adjacency, but it is still confusing in context of the rest of the page. I suggest the typography of the left-hand sides of these two equations be changed to [BC]^2 and [AC]^2 respectively, or use over-bars over all the line segment letter-pairs, which is the standard line-segment notation. Thanks, Craig H Collins 26 Jan 2023
 Fixed by adding a hatnote. D.Lazard (talk) 10:43, 26 January 2023 (UTC)
Fixed by adding a hatnote. D.Lazard (talk) 10:43, 26 January 2023 (UTC)
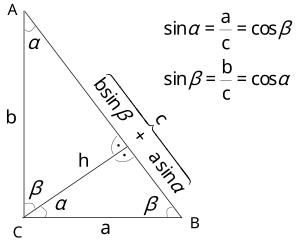
Proof using trigonometry
@Kencf0618 added this section, but the proof itself is not available in any of the links attached. 181.167.210.101 (talk) 16:24, 25 March 2023 (UTC)
- I removed the section. D.Lazard (talk) 16:53, 25 March 2023 (UTC)
Granted, we'd all like to see the proof, but in the meantime, why shouldn't we at least note the two students who have made the claim?98.149.97.245 (talk) 14:29, 26 March 2023 (UTC)
- To mention that, we need an independent source atesting that the claim is true and that the the proof is correct and not circular. As far as one can see for the provided summary, the use the law of sines, without establishing that this law can be proved without the Pythagorean theorem. This makes the claim very dubious. D.Lazard (talk) 16:30, 26 March 2023 (UTC)
- It’s cool that these students got to present their work to mathematicians, but as of yet any claims about it are not verifiable, because no details have been published anywhere. Claims that this is the “first trigonometric proof” are clearly false, based on the students’ reliance on outdated sources / lack of a literature review. (Not trying to knock the students here.) Ultimately this seems mostly like a feel-good news report rather than significant mathematical news. If the news reports inspire other students to be curious and make their own discoveries (whether or not they turn out to be novel), that’s great. But Wikipedia shouldn’t exaggerate. –jacobolus (t) 20:00, 26 March 2023 (UTC)
Here's a reference to a non-circular proof using trigonometry (Zimba, 2009):
https://forumgeom.fau.edu/FG2009volume9/FG200925.pdf Tkircher (talk) 18:13, 26 March 2023 (UTC)
Upload the triangles to Wikimedia Commons. Youtuber reconstructed the proof. https://m.youtube.com/watch?v=nQD6lDwFmCc71.201.78.227 (talk) 05:28, 1 April 2023 (UTC)
Proof by Jackson and Johnson (2023): I support the inclusion of the trigonometric proofs in the main article.[1][2] The proof by Zimba in 2009 is correct.[2] Possibly others have also noticed the error in Loomis book,[3] however, it is great that now the high school students have provided another quite beautiful proof.[1] I have prepared an SVG sketch of their construction and already have uploaded it to Wikipedia commons:
The proof is valid for almost all right triangles, except for the case when a=b, i.e. Isosceles Right Triangle.
For the case when , we can always choose to orient the right triangle so that a<b as shown in the figure. Then we reflect the right triangle to obtain point D. We extend leg BE perpendicular to AB, and extend AD until it crosses BE, here is why it is necessary for . Then, we need to sum a convergent infinite geometric series where in order to compute from the large right triangle :
Finally, we employ the Law of sines in to find out:
which upon substituion with and gives the Pythagorean theorem:
.
For the special case when , the geometric series does not converge because , however, the proof is purely algebraic using the areas of triangles , and , namely: , but since , it follows that .
Now, I would like to roast the text written by Loomis on page 244 in his book,[3] freely accessible from ERIC:
NO TRIGONOMETRIC PROOFS
Facing forward the thoughtful reader may raise the question: Are there any proofs based upon the science of trigonometry or analytical geometry?
There are no trigonometric proofs, because all the fundamental formulae of trigonometry are themselves based upon the truth of the Pythagorean Theorem; because of this theorem we say , etc. Trigonometry is because the Pythagorean Theorem is.
This shows poor understanding by Elisha Scott Loomis of what trigonometry is. The sine and cosine are originally usually defined (in high school textbooks) as ratio of two sides inside a right triangle and do not necessitate any knowledge of the equality . Apparently the mistake by author, Loomis, was clearly pointed out by Zimba in 2009[2] but it is possible that others have been aware of this error even before 2009.
I hope that part of my notes can be reused by other editors to update the main article. Danko Georgiev (talk) 22:43, 1 April 2023 (UTC)
- Your Jackson and Johnson link is just an announcement of a presentation with no details. Where’d the figure come from? –jacobolus (t) 02:28, 2 April 2023 (UTC)
sine and cosine are originally defined as ratio of two sides inside a right triangle
– what do you mean by “originally defined”? Historically sine and cosine were defined as particular line segments relative to a reference circle. –jacobolus (t) 02:29, 2 April 2023 (UTC)- The figure comes from the news video from WWL-TV, exactly frame 1:30 min [4] Of course, the labeling and notation in my Figure is mine, but I have prepared it in the most pedagogical style that I came up with for possible usage in Wikipedia.
- The "original" should have been: "the first definition ever encountered in high school textbook by an average person living on Earth". See, Wikibooks.org: High School Trigonometry/Defining Trigonometric Functions/The Sine, Cosine, and Tangent Functions. In other words, I was not talking about "historical" definitions, as historical events have no bearing on whether a modern mathematical proof is circular or not. By the way, what I have meant as "original definition" of sine is already in the Figure: Danko Georgiev (talk) 08:43, 2 April 2023 (UTC)
- A Wikipedian's speculative reconstruction of an unpublished presentation based on a blurry slide shown on a TV news program doesn’t really seem like it meets WP:RS or WP:OR. Why don’t we just wait for these two students to publish their work? –jacobolus (t) 09:02, 2 April 2023 (UTC)
- "speculative reconstruction" - you are the most pleasant person on Earth, so I will tell you this: if you are incompetent to check whether a mathematical proof is correct or not, you should not edit mathematical pages. Also, my reconstruction is "exact" and there is nothing speculative in it. Danko Georgiev (talk) 09:14, 2 April 2023 (UTC)
- I do not know whether the above proof is that of the two students (this is the meaning of “speculative”). But I see that, although rather ingenious, this proof uses two tools that do not belong to trigonometry, namely the concept of the sum of an infinite series, and the parallel postulate, which is equivalent with the widely used fact that the sum of the acute angles of a right triangle is So this proof does not add nothing to the classical proof of the Pythagorean theorem, directly based on the parallel postulate, and nothing allows saying that this is a trigonometric proof. D.Lazard (talk) 10:06, 2 April 2023 (UTC)
- This proof is "trigonometric" because it uses the definition of "sine" to compute the lengths of the sides in the infinite chain or right triangles. Also, pointing out that the proof depends on the parallel postulate is a dismissive and meaningless remark, and it is not different from making the remark that the proof also depends on the Peano axioms of arithmetic, because one uses addition, subtraction, multiplication and division. To that, I would just say: "so what?". In the axiomatization of any theory, one has the freedom to choose "what is an axiom" and "what is a theorem", i.e., there are some axiomatizations in which a certain statement is an axiom, and there are other axiomatizations in which the same statement in proven as a theorem from some other axioms. In summary, the proof by the New Orleans students is "trigonometric" because it heavily relies on repeated use of the definition of sine as ratio of two sides in right triangle. The rest in the proof is just algebra, e.g. Peano axioms which have nothing to do with the Pythagorean theorem. Also, the issue is not so much about the new proof, but the toxic culture by Wikipedia editors who are incompetent, but bully others as explained by Zimba in his article. And another issue, is the apparently influential error done by Elisha Scott Loomis which is mindlessly recited by others, for more see User:Danko_Georgiev/sandbox. Danko Georgiev (talk) 10:27, 2 April 2023 (UTC)
- Every proof of the Pythagorean theorem necessarily relies on the parallel postulate (often filtered through intermediate concepts like a notion of similar triangles of different sizes). The two are logically equivalent and removing the parallel postulate breaks the Pythagorean relation. –jacobolus (t) 18:15, 2 April 2023 (UTC)
- It is “speculative” in the sense that you are only working from one blurry photograph of one slide of a talk for which you are missing the other slides and the oral content of the talk. It is impossible to verify that the reconstruction is the same as the original. –jacobolus (t) 18:16, 2 April 2023 (UTC)
- I do not know whether the above proof is that of the two students (this is the meaning of “speculative”). But I see that, although rather ingenious, this proof uses two tools that do not belong to trigonometry, namely the concept of the sum of an infinite series, and the parallel postulate, which is equivalent with the widely used fact that the sum of the acute angles of a right triangle is So this proof does not add nothing to the classical proof of the Pythagorean theorem, directly based on the parallel postulate, and nothing allows saying that this is a trigonometric proof. D.Lazard (talk) 10:06, 2 April 2023 (UTC)
- "speculative reconstruction" - you are the most pleasant person on Earth, so I will tell you this: if you are incompetent to check whether a mathematical proof is correct or not, you should not edit mathematical pages. Also, my reconstruction is "exact" and there is nothing speculative in it. Danko Georgiev (talk) 09:14, 2 April 2023 (UTC)
- The reason I asked about "original definition" was not just to nitpick, but because the definitions and conceptual scope here matter a lot if we are trying to figure out which concepts are built on which others. If you read old geometry books or old trigonometry books (up through the 17th century or so) they do not have a concept of lengths as numbers or even ratios of lengths as numbers, but only a concept of pairs of ratios of straight lines (what Euclid calls a 'straight line' we now consider in terms of modern concepts to mean the length of a line segment) in proportion. So you can have AB : CD :: EF : GH, for straight lines AB &c., but it was not considered meaningful to write AB / CD or AB × CD (the former could be used as a ratio in proportion and the latter would be instead described as "the rectangle with sides AB and CD or the like). (I would link the relevant Wikipedia articles but they currently do a very poor job of explaining the conceptual distinctions / historical development).
- Trigonometry was originally developed by Hipparchus and Ptolemy inter alia, and later by Indians, Arabs, & al. in the form of spherical trigonometry, a branch of astronomy, first with tables of chords and later with tables of sines describing (approximately) the relation between arc length and chord length. From what I understand, the concepts were not entirely well developed/established in the style of Euclid, and were treated as a kind of applied/practical approximate subject, not really part of geometry per se. In antiquity what we now do with "planar trigonometry" (of the style found in high school books) would instead be accomplished by Euclid-style constructions / theorems. For example Elements propositions 2.12–13 are what we now call the law of cosines:
2.12: In obtuse-angled triangles the square on the side opposite the obtuse angle is greater than the sum of the squares on the sides containing the obtuse angle by twice the rectangle contained by one of the sides about the obtuse angle, namely that on which the perpendicular falls, and the straight line cut off outside by the perpendicular towards the obtuse angle.
2.13: In acute-angled triangles the square on the side opposite the acute angle is less than the sum of the squares on the sides containing the acute angle by twice the rectangle contained by one of the sides about the acute angle, namely that on which the perpendicular falls, and the straight line cut off within by the perpendicular towards the acute angle.
- But notice there's no concept of angle measure or cosine (some scalar number as the ratio of sides) involved here.
- So the main reason that there were historically no "trigonometric proofs" of the Pythagorean theorem is that the subject of trigonometry was treated as part of a separate tradition with separate methods, standards, and scope than the subject of geometry.
- If you want to call a proof "trigonometric" that depends on first carefully defining what "trigonometric" means, then establishing all of the relevant theorems of such trigonometry independent from the Pythagorean theorem. This is going to necessarily be a bit of an affectation, because "trigonometry" in the sense of ratios in triangles is based on the concept of similar right-angled triangles which is logically equivalent to the Pythagorean theorem. So it’s always going to be an indirect route to proving something that you could just as easily prove more directly from the same axioms. But of course, the same can also be said for many other kinds of proofs of the Pythagorean theorem, such as those built on integral calculus.
- Anyway, I am not just trying to be a party pooper. I will wholeheartedly agree that it’s great when high school students get excited about math. –jacobolus (t) 21:14, 2 April 2023 (UTC)
- "because "trigonometry" in the sense of ratios in triangles is based on the concept of similar right-angled triangles which is logically equivalent to the Pythagorean theorem" -- this is false. To define ratio of two sides you do NOT need the the Pythagorean theorem. And I can prove it to you immediately: to define the ratio, you need only the notion of "scale" and "similarity". For example, I use a triangle that does not have a right angle and define the notions of "scaling" and "similarity". These cannot be "presuming" right angle, because you will arrive at contradiction when talking about similar non-right triangles. This establishes, that the concepts "scale" and "similarity" are independent of the right triangle. Now, it is easy to define the functions "sine" and "cosine" as ratios, but you specify that you will be using right triangle. These functions are independent from the Pythagorean theorem because the "scale" and "similarity" were independent and the only new thing added was the "right angle". Using a simple argument of removal of independent things, if you say that the definition of "sine" and "cosine" already imply the Pythagorean theorem, then you will be stating in effect that the existence of the right triangle (or right angle) is implying the Pythagorean theorem, which is provably false -- namely, there are non-Euclidean geometries in which the Pythagorean theorem is false, but there exist right triangles and right angles. In summary, I have just proved to you that you are talking nonsense. The definition of sine and cosine as is usually done in high school textbooks, does not imply the Pythagorean theorem. Even, I can give you counterexample so that it becomes crystal clear to you: draw a triangle on a sphere! The definition of "sine" and "cosine" in the curved triangle is perfectly well defined as the ratio of the curved triangle sides. So, the Pythagorean theorem is absolutely false on the sphere and the "sine" and "cosine" functions have nothing to do with the "sine" and cosine" of the Euclidean space. So back-off: the definition of the sine and cosine as ratio of sides depends on the curvature of the space. I am using the parallel postulate to impose "flatness", not the Pythagorean theorem. What you are claiming is demonstrably false, and has nothing to say on the correctness of the proof that I have attributed to Jackson and Johnson above. Danko Georgiev (talk) 00:06, 3 April 2023 (UTC)
- P.S. in case if you are wondering what I am saying is this: "the ratio definitions of sine and cosine" are independent on the Pythagorean theorem because in curved space sin^2 + cos^2 is NOT 1. So I feel that we have identified the origin of the whole debate, namely, there are different non-equivalent definitions of the trigonometric functions. If you define "sin^2 := 1 - cos^2", then this is indeed the same as the Pythagorean theorem. But if you define the sine function as ratio, this is much more general definition that is curvature-dependent and is NOT equivalent to the Pythagorean theorem. Do you agree? Danko Georgiev (talk) 00:23, 3 April 2023 (UTC)
- You are misunderstanding/misstating what I said. Perhaps I was not clear enough.
To define ratio of two sides you do NOT need the the Pythagorean theorem
– nobody has said this. However, to establish that the ratio of the sides is uniquely determined by the angle, and vice versa, you need the parallel postulate or something logically equivalent (the Pythagorean relation is one possible axiom you could start with; or you could start with some statement about similar triangles as your axiom; or various others).... define the notions of "scaling" and "similarity"
you are saying the same thing I just said: you could establish definitions of sine etc. by first establishing a notion of similar triangles. there are non-Euclidean geometries in which the Pythagorean theorem is false, but there exist right triangles and right angles. [...] definition of the sine and cosine as ratio of sides depends on the curvature of the space
You are repeating what I just said.- To be precise, in the context of the sphere, a right triangle with sides and right angle at where etc. ( being the center of the sphere, and meaning the measure of the central angle), the analogous Pythagorean theorem is instead the relation:
- where Because does not scale proportionally with both of its arguments (the way + does), there is no notion of similarity on the sphere. (The same formula holds in the hyperbolic plane, if you take to be the hyperbolic half-tangents instead of circular half-tangents of the sides. The Euclidean version is the limit of the spherical/hyperbolic formula when )
- If we don't want to rely on a concept of angle measure, we can let be points on a sphere in Euclidean space with meaning the point antipodes to , and define half-tangents of sides like etc., where is a vector and the means the geometric quotient so is the magnitude of a unitless bivector oriented in the plane of and We can even take the absolute value signs off if we want, because these bivectors square to scalars. But that's probably enough about the side question of Pythagoras in non-Euclidean planes. –jacobolus (t) 00:34, 3 April 2023 (UTC)
- OK, thanks for the extra clarification. Euclid's parallel postulate is needed in the proof because you need to establish that the sides AE and BE intersect when . You need the "flatness" of the space to prove the Pythagorean theorem. There was no dispute that you need the parallel postulate at all. Also, risking to repeat myself, I do not object to take Pythagorean axiom and prove the parallel postulate as theorem, i.e., exchanging which of the two statements is axiom and which is theorem. What I do not understand is to what exactly you are objecting in the proof in order NOT to classify it as "trigonometric"? A side note, based on your mentioning of integral calculus and Bogomolny's page: to sum convergent infinite series you only need to define limits - in contrast, to define differential and integral calculus you need to add so much extra theory that I am not sure (1) that all of this extra stuff is necessary for the proof of the Pythagorean theorem, and (2) whether some of this extra stuff does not actually require the Pythagorean theorem. Frankly, I no longer understand what your concerns were, and what definitions you wanted to have from me. Just take a basic high school textbook of trigonometry and let us call it a night. Danko Georgiev (talk) 00:59, 3 April 2023 (UTC)
- What I am saying is nobody has bothered too much in the past trying to invent "trigonometric" proofs here because there’s no particular reason (beyond amusement) to try to establish the tools of plane trigonometry independent from basic relations of Euclidean space (like the Pythagorean relation). Any trigonometric proof you end up with is more or less a slightly more cumbersome variant of an analogous proof you could make just in terms of similar triangles, and establishing the tools of trigonometry necessarily leans on already developing a concept of similar triangles. So whatever trigonometric proof you come up with somewhat has the flavor of a Rube Goldberg machine, adding extra indirection just for the sake of it. Whether such a proof is properly "trigonometric" or not is not really an empirical question but a semantic one, dependent on what someone means by "trigonometric proof", and whether the reader considers a proof that is same except for having some side ratios replaced with sines/cosines to be novel. –jacobolus (t) 01:12, 3 April 2023 (UTC)
- OK, thanks for the extra clarification. Euclid's parallel postulate is needed in the proof because you need to establish that the sides AE and BE intersect when . You need the "flatness" of the space to prove the Pythagorean theorem. There was no dispute that you need the parallel postulate at all. Also, risking to repeat myself, I do not object to take Pythagorean axiom and prove the parallel postulate as theorem, i.e., exchanging which of the two statements is axiom and which is theorem. What I do not understand is to what exactly you are objecting in the proof in order NOT to classify it as "trigonometric"? A side note, based on your mentioning of integral calculus and Bogomolny's page: to sum convergent infinite series you only need to define limits - in contrast, to define differential and integral calculus you need to add so much extra theory that I am not sure (1) that all of this extra stuff is necessary for the proof of the Pythagorean theorem, and (2) whether some of this extra stuff does not actually require the Pythagorean theorem. Frankly, I no longer understand what your concerns were, and what definitions you wanted to have from me. Just take a basic high school textbook of trigonometry and let us call it a night. Danko Georgiev (talk) 00:59, 3 April 2023 (UTC)
- "because "trigonometry" in the sense of ratios in triangles is based on the concept of similar right-angled triangles which is logically equivalent to the Pythagorean theorem" -- this is false. To define ratio of two sides you do NOT need the the Pythagorean theorem. And I can prove it to you immediately: to define the ratio, you need only the notion of "scale" and "similarity". For example, I use a triangle that does not have a right angle and define the notions of "scaling" and "similarity". These cannot be "presuming" right angle, because you will arrive at contradiction when talking about similar non-right triangles. This establishes, that the concepts "scale" and "similarity" are independent of the right triangle. Now, it is easy to define the functions "sine" and "cosine" as ratios, but you specify that you will be using right triangle. These functions are independent from the Pythagorean theorem because the "scale" and "similarity" were independent and the only new thing added was the "right angle". Using a simple argument of removal of independent things, if you say that the definition of "sine" and "cosine" already imply the Pythagorean theorem, then you will be stating in effect that the existence of the right triangle (or right angle) is implying the Pythagorean theorem, which is provably false -- namely, there are non-Euclidean geometries in which the Pythagorean theorem is false, but there exist right triangles and right angles. In summary, I have just proved to you that you are talking nonsense. The definition of sine and cosine as is usually done in high school textbooks, does not imply the Pythagorean theorem. Even, I can give you counterexample so that it becomes crystal clear to you: draw a triangle on a sphere! The definition of "sine" and "cosine" in the curved triangle is perfectly well defined as the ratio of the curved triangle sides. So, the Pythagorean theorem is absolutely false on the sphere and the "sine" and "cosine" functions have nothing to do with the "sine" and cosine" of the Euclidean space. So back-off: the definition of the sine and cosine as ratio of sides depends on the curvature of the space. I am using the parallel postulate to impose "flatness", not the Pythagorean theorem. What you are claiming is demonstrably false, and has nothing to say on the correctness of the proof that I have attributed to Jackson and Johnson above. Danko Georgiev (talk) 00:06, 3 April 2023 (UTC)
- A Wikipedian's speculative reconstruction of an unpublished presentation based on a blurry slide shown on a TV news program doesn’t really seem like it meets WP:RS or WP:OR. Why don’t we just wait for these two students to publish their work? –jacobolus (t) 09:02, 2 April 2023 (UTC)
- Edited text with references on the "Trigonometric Proof of the Pythagorean Theorem by Jackson and Johnson (2023)" and "Nonexistence of trigonometric proofs of the Pythagorean Theorem claimed by Elisha Scott Loomis" can be copy-pasted from my sandbox: User:Danko_Georgiev/sandbox. Danko Georgiev (talk) 10:56, 2 April 2023 (UTC)
- The content in your sandbox is about 5–10 times too long to be in scope for this article, in my opinion, in accord with WP:DUE. If you cut it down to ~1–3 short paragraphs including the shortest exemplary trigonometric proof that can be found in published literature, with alternatives discussed (not spelled out) in footnotes, it could fit in a section "Trigonometric proofs". This article currently picks about 3 or 4 exemplary proofs (the oldest and most famous ones) and then compresses discussion of the remaining hundreds of alternative proofs into a paragraph or two and maybe a couple figures about each other broad category. That seems like about the right strategy to keep the article legible to an anticipated typical reader, and keep the narrative at least somewhat moving along. –jacobolus (t) 19:05, 2 April 2023 (UTC)
Correcting a misleading statement by Loomis or removing it from the article is one thing, including the new proof is another. As long as the proof is not published in journal or book, I don't really see a good reason to include it here as an inclusion at this point collides with various Wikipedia policies. This has nothing to do with correctness of the proof or whether one considers it trigonometric or not. And even if such a publication has become available, that is still no reason for an automatic inclusion. Keep in mind our article contains only a small subset of the available proofs of the theorem, which primarily means those most common in literature and/or might representative for a larger class of proofs.--Kmhkmh (talk) 10:58, 2 April 2023 (UTC)
- The inclusion of the proof by Jackson and Johnson in the main article will serve several purposes: (1) it is mathematically "beautiful" construction, which cannot be said to the majority of say 370 proof in the book by Loomis. For example, if you just open the free online PDF copy of the Loomis book and browse through it, you may get the impression that some of the proofs are over-crowded constructions whose only purpose is to increase the count of proofs; (2) the fact that the proof is produced by high school students is remarkable in itself in terms of authorship, and (3) it is important to point out that there is a toxic environment in mathematics, so much so that since 1927 when the book by Loomis was published, it has been used to suppress correct arguments by simply "quoting" page 244, which says in all caps that there are "NO TRIGONOMETRIC PROOFS". With regard to notability concerns, the fact that the proof by Jackson and Johnson has been featured in The Guardian[5] is sufficient to merit coverage in Wikipedia. If you count the number of years since 1927, you can determine that the event of high school students disproving claims published in academic mathematical books is one event per 96 years. Danko Georgiev (talk) 11:28, 2 April 2023 (UTC)
- As far as I understand this article didn’t ever repeat Loomis’s claim, and whether a proof of the Pythagorean theorem can be "trigonometric" or not frankly doesn't seem that important (to me personally), being to a substantial degree a semantic dispute based on the imprecise definition of the word "trigonometric". But while we are here, plenty of sources can be found about "trigonometric" proofs and disputing Loomis's claim. Several are discussed/linked from Bogomolny (2012) "More Trigonometric Proofs of the Pythagorean Theorem". If someone wants to add a section about Trigonometric proofs after the section about Pythagorean Theorem § Algebraic proofs I would be indifferent to it. But it should stick to published claims, not original research by Wikipedians. Encouraging high school students is a valuable goal, but trying to force discussion of high school students into Wikipedia articles is not really the best mechanism for that in my opinion. –jacobolus (t) 18:43, 2 April 2023 (UTC)
- The proof given by Bogomolny is circular.
1. Consider a point .
2. and are the lengths of the legs of a right triangle.
3. Their projections onto the hypotenuse have lengths and .
4. Therefore,- To compute distances from coordinates e.g. to the origin (or any other point) requires the use of the distance formula which is exactly the Pythagorean theorem. There is no way to proceed from coordinates to lengths, because one does not have a distance function (or metric) that follows from these coordinates. Imposing the Euclidean distance formula is to assume the Pythagorean theorem from the start, hence, it is a perfect example of circular reasoning.
- By the way, the "nice" thing about the trigonometric proof by Jackson and Johnson is that you just need a ruler and compass to construct the right triangles, and then just some straightforward arithmetic. You may be summing infinite series, but at least you are not doing the "circular" business. Next time when you provide references, at least be sure that you are not putting forward questionable materials. Danko Georgiev (talk) 21:17, 2 April 2023 (UTC)
- You put the distance function in there, not Bogomolny. But I will agree that this page does not go through all of the details necessary to demonstrate to a skeptical reader that the proof is not circular. That would take developing many more pages of preliminary results. In particular, if you want to talk about an angle measure as an arclength of a unit-radius circle, and put it on solid rigorous footing, you probably need to bring in integral calculus. –jacobolus (t) 21:19, 2 April 2023 (UTC)
- I did not put anything anywhere. I ask only the question: how do you go from "coordinates" to "lengths". Option 1: you tell me that you use the distance function that I wrote above and you shoot yourself in the foot. Option 2: you tell me that I do not have a formula but I need to use some other method using ruler and compass. Then I will reply this: "who are you trying to bamboozle with the "coordinate terminology"?? If you put coordinates in point 1 in the proof, and then you are telling me that I cannot use these coordinates for anything, then you are not providing any proof. In fact, by inserting terminology that I cannot use later for anything I am going to conclude that you have no proof at all. period. Danko Georgiev (talk) 21:32, 2 April 2023 (UTC)
- Note this in polar coordinates, not rectangular ones. I think the author (in the context of a message to a newsgroup) was compressing their description of a point on the circle for the sake of concision/clarity because a detailed rigorous elaboration would be cumbersome. The ruler-and-compass way would be to just pick out a point on the circle. But whether this avoids the Pythagorean theorem or not is going to depend on making careful definitions of sine and cosine when starting from a point on the circle. –jacobolus (t) 21:35, 2 April 2023 (UTC)
- Wow, for the sake of clarity the argument has become so transparent that I can no longer see it. Wonderful job! Danko Georgiev (talk) 21:41, 2 April 2023 (UTC)
- P.S. can we try to avoid the sarcasm, insults, etc. here? –jacobolus (t) 21:47, 2 April 2023 (UTC)
- Wow, for the sake of clarity the argument has become so transparent that I can no longer see it. Wonderful job! Danko Georgiev (talk) 21:41, 2 April 2023 (UTC)
- Note this in polar coordinates, not rectangular ones. I think the author (in the context of a message to a newsgroup) was compressing their description of a point on the circle for the sake of concision/clarity because a detailed rigorous elaboration would be cumbersome. The ruler-and-compass way would be to just pick out a point on the circle. But whether this avoids the Pythagorean theorem or not is going to depend on making careful definitions of sine and cosine when starting from a point on the circle. –jacobolus (t) 21:35, 2 April 2023 (UTC)
- "That would take developing many more pages of preliminary results" -- this is exactly the point at which I stop talking with somebody. Danko Georgiev (talk) 21:50, 2 April 2023 (UTC)
- Your proof above also requires many unstated preliminary results. You need to carefully (1) define what you mean by a side labeled by some algebraic expression and prove that it is meaningful to perform algebra on your labels so defined, (2) define what you mean by an angle of label e.g. (3) define what you mean by and prove that it is equal to the quotient of two algebraically expressed sides irrespective of the particular sides/angles involved, (4) define what it means to add angles, (5) define what you mean by (6) establish that it is meaningful to sum an infinite series of algebraic expressions and have the sum be a meaningful label for the side that your segments placed end-to-end converge to, (7) prove that your specific series are convergent, (8) prove the law of sines, etc. If we think hard we could probably come up with at least half a dozen more prerequisite steps. If you rely only on what is found in a typical high school textbook, the result will assuredly have some holes that are not rigorously established to the satisfaction of modern mathematicians. Making sure that none of these involve any invocation of the Pythagorean theorem is possible but you’ll have to be careful about it because books describing all of these concepts usually take the Pythagorean theorem for granted and don’t bother avoiding all of its consequences or re-deriving them in a loopy way. Indeed, modern rigorous geometry books often side-step this whole mess by starting with a coordinate plane with "Euclidean structure", i.e. some distance function, bilinear form, or quadratic form, so that the Pythagorean theorem is essentially taken as an axiom, or for a general right triangle involves some trivial arithmetic. –jacobolus (t) 22:10, 2 April 2023 (UTC)
- Note, I’m not saying you should do this. My point is just that to prove a theorem we need to establish some context of previously proven results considered fair game to built from. Usually in proving new theorems mathematicians are happy to use any already-accepted results from anywhere in mathematics, while in writing a textbook an author will pick some set of assumed prerequisites and then try to make a logically rigorous path from those to everything else in the book, without internal circularity or dependence on concepts that are out of scope. But when making new decontextualized proofs of old theorems, especially with a constraint like “can’t rely on anything involving the Pythagorean theorem”, some kind of context needs to be established (otherwise readers such as yourself "can no longer see" the argument). The concepts and methods you are using here (or that the authors in Bogomolny's message group used) are not defined in the original context of the Pythagorean theorem, such as Euclid's Elements, which limits itself to a few simple axioms and only "geometric" reasoning, with no concept of angle measure and any algebra dressed up as geometry. –jacobolus (t) 22:55, 2 April 2023 (UTC)
- But there is no need to refer Euclid as the contextual framework (or prequisite) for our article and no need to rely on other historical contexts/prequisites for the proofs provided in our article. Instead we consider as prequisites/context what is currently commonly taught in schools (the knowledge most of our readers start off with) and what is given in modern geometry books (rather than Euclid). Euclid and other older sources (and their notations and approaches) are primarily of interest for historical reasons/aspects, but not really in terms of giving accessible proofs.--Kmhkmh (talk) 09:43, 3 April 2023 (UTC)
- The reason to bring up Euclid is that the whole of Book 1 of Elements leads up to the proof of the Pythagorean Theorem. (Indeed I think we could do a significantly better job of explaining the history/context about the proof of proposition I.47 in this article; I should perhaps try to write some more about it.) You can think of this as a proof from scratch. So if you look at Euclid you can see precisely what is required from axioms right up through the final theorem. On the other hand, trigonometry books, calculus books, etc. generally take the Pythagorean theorem and various other theorems built on top for granted already. –jacobolus (t) 14:54, 3 April 2023 (UTC)
- I understand that. However I'm just saying that at least from a non-historical perspective this isn't really the appropriate approach, instead one should use modern (synthetic) geometry books starting from scratch (essentially Hilbert and later) or as far as our WP article is concerned use as prequisite/context what is taught in middle/high school math. This matters for the discussion above as you do not have to deal with concepts in the same order as Euclid or some other historic sources did, in particular you do not have to consider historic approaches to trigonometry, but can you can approach it via the ratios in similar right angled triangles (as given in the forum geometricorum article above). Or in the bigger picture starting from scratch you can go the following route (which in my experience school geometry usually does, without necessarily spelling it out explicity): axioms (essentially still the same as Euclid's) -> concept of areas -> area of a triangles -> intercept theorem/thales' (basic similarity) theorem -> similar triangles -> Pythagorean theorem. Now instead of using similar triangles directly to prove the Pythagorean theorem (this proof is in our article), one can introduce sine and cosine first based on similar triangles and then use trigonometry to prove the Pythagorean theorem. That yields you a path to the Pythagorean from scratch (or first principles) but in different order than Euclid. And as I said from today's perspective you still want from scratch but there is no need to follow Euclid's order. Moreover with regards to the order (or prequisites) we should follow popular modern geometry books rather then Euclid. The order I outlined above btw was taken from Hans Schupp's Elementargeometrie (UTB, 1977).--Kmhkmh (talk) 23:10, 3 April 2023 (UTC)
- The reason to bring up Euclid is that the whole of Book 1 of Elements leads up to the proof of the Pythagorean Theorem. (Indeed I think we could do a significantly better job of explaining the history/context about the proof of proposition I.47 in this article; I should perhaps try to write some more about it.) You can think of this as a proof from scratch. So if you look at Euclid you can see precisely what is required from axioms right up through the final theorem. On the other hand, trigonometry books, calculus books, etc. generally take the Pythagorean theorem and various other theorems built on top for granted already. –jacobolus (t) 14:54, 3 April 2023 (UTC)
- But there is no need to refer Euclid as the contextual framework (or prequisite) for our article and no need to rely on other historical contexts/prequisites for the proofs provided in our article. Instead we consider as prequisites/context what is currently commonly taught in schools (the knowledge most of our readers start off with) and what is given in modern geometry books (rather than Euclid). Euclid and other older sources (and their notations and approaches) are primarily of interest for historical reasons/aspects, but not really in terms of giving accessible proofs.--Kmhkmh (talk) 09:43, 3 April 2023 (UTC)
- Note, I’m not saying you should do this. My point is just that to prove a theorem we need to establish some context of previously proven results considered fair game to built from. Usually in proving new theorems mathematicians are happy to use any already-accepted results from anywhere in mathematics, while in writing a textbook an author will pick some set of assumed prerequisites and then try to make a logically rigorous path from those to everything else in the book, without internal circularity or dependence on concepts that are out of scope. But when making new decontextualized proofs of old theorems, especially with a constraint like “can’t rely on anything involving the Pythagorean theorem”, some kind of context needs to be established (otherwise readers such as yourself "can no longer see" the argument). The concepts and methods you are using here (or that the authors in Bogomolny's message group used) are not defined in the original context of the Pythagorean theorem, such as Euclid's Elements, which limits itself to a few simple axioms and only "geometric" reasoning, with no concept of angle measure and any algebra dressed up as geometry. –jacobolus (t) 22:55, 2 April 2023 (UTC)
- Your proof above also requires many unstated preliminary results. You need to carefully (1) define what you mean by a side labeled by some algebraic expression and prove that it is meaningful to perform algebra on your labels so defined, (2) define what you mean by an angle of label e.g. (3) define what you mean by and prove that it is equal to the quotient of two algebraically expressed sides irrespective of the particular sides/angles involved, (4) define what it means to add angles, (5) define what you mean by (6) establish that it is meaningful to sum an infinite series of algebraic expressions and have the sum be a meaningful label for the side that your segments placed end-to-end converge to, (7) prove that your specific series are convergent, (8) prove the law of sines, etc. If we think hard we could probably come up with at least half a dozen more prerequisite steps. If you rely only on what is found in a typical high school textbook, the result will assuredly have some holes that are not rigorously established to the satisfaction of modern mathematicians. Making sure that none of these involve any invocation of the Pythagorean theorem is possible but you’ll have to be careful about it because books describing all of these concepts usually take the Pythagorean theorem for granted and don’t bother avoiding all of its consequences or re-deriving them in a loopy way. Indeed, modern rigorous geometry books often side-step this whole mess by starting with a coordinate plane with "Euclidean structure", i.e. some distance function, bilinear form, or quadratic form, so that the Pythagorean theorem is essentially taken as an axiom, or for a general right triangle involves some trivial arithmetic. –jacobolus (t) 22:10, 2 April 2023 (UTC)
- I did not put anything anywhere. I ask only the question: how do you go from "coordinates" to "lengths". Option 1: you tell me that you use the distance function that I wrote above and you shoot yourself in the foot. Option 2: you tell me that I do not have a formula but I need to use some other method using ruler and compass. Then I will reply this: "who are you trying to bamboozle with the "coordinate terminology"?? If you put coordinates in point 1 in the proof, and then you are telling me that I cannot use these coordinates for anything, then you are not providing any proof. In fact, by inserting terminology that I cannot use later for anything I am going to conclude that you have no proof at all. period. Danko Georgiev (talk) 21:32, 2 April 2023 (UTC)
- You put the distance function in there, not Bogomolny. But I will agree that this page does not go through all of the details necessary to demonstrate to a skeptical reader that the proof is not circular. That would take developing many more pages of preliminary results. In particular, if you want to talk about an angle measure as an arclength of a unit-radius circle, and put it on solid rigorous footing, you probably need to bring in integral calculus. –jacobolus (t) 21:19, 2 April 2023 (UTC)
- As far as I understand this article didn’t ever repeat Loomis’s claim, and whether a proof of the Pythagorean theorem can be "trigonometric" or not frankly doesn't seem that important (to me personally), being to a substantial degree a semantic dispute based on the imprecise definition of the word "trigonometric". But while we are here, plenty of sources can be found about "trigonometric" proofs and disputing Loomis's claim. Several are discussed/linked from Bogomolny (2012) "More Trigonometric Proofs of the Pythagorean Theorem". If someone wants to add a section about Trigonometric proofs after the section about Pythagorean Theorem § Algebraic proofs I would be indifferent to it. But it should stick to published claims, not original research by Wikipedians. Encouraging high school students is a valuable goal, but trying to force discussion of high school students into Wikipedia articles is not really the best mechanism for that in my opinion. –jacobolus (t) 18:43, 2 April 2023 (UTC)
- Here you are: (1) Ingredients of the proof (2) Steps of the actual proof. Nobody is required to read Euclid's Elements in order to understand what the ingredients of the proof are and what are the steps in which they are used. Danko Georgiev (talk) 09:54, 3 April 2023 (UTC)
References
- ^ a b Jackson, Ne'Kiya D. and Johnson, Calcea Rujean (2023). "An Impossible Proof Of Pythagoras". AMS Special Session on Undergraduate Mathematics and Statistics Research, I: SS30A.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - ^ a b c Zimba, Jason (2009). "On the possibility of trigonometric proofs of the Pythagorean theorem". Forum Geometricorum. 9: 275–278.
- ^ a b Loomis, Elisha Scott (1968). The Pythagorean Proposition. Washington, D.C.: National Council of Teachers of Mathematics.
- ^ WWL-TV (March 23, 2023). "New Orleans teens make mathematical discovery unproven for 2,000 years". New Orleans, Louisiana. WWL-TV Channel 4, Eyewitness News, CBS affiliate in New Orleans. Retrieved 2023-04-02.
- ^ Ramon Antonio Vargas (March 24, 2023). "US teens say they have new proof for 2,000-year-old mathematical theorem". The Guardian.
@Danko Georgiev: Imho neither of the three arguments for inclusion you raised above do really apply:
- a) Whether a proof is in Loomis or not (or the quality of Loomis' collection) was never an argument for including a proof here. In fact I'd argue Loomis is completely irrelevant for the inclusion of a proof. A reason for inclusion instead is that a proof (which may or may not be included in Loomis' book) is that the proof is popular/appreciated in external publications (rather than primarily appreciated by some Wikipedians with (almost) no external publications).
- b) It is great that some high school students came up with a new proof, but that doesn't necessarily yield a reason to iclude the proof here. If that proof becomes popular in publications down the road, then we will have a strong reason to include it, but not before. Also we don't do promotional stuff, in a sense that we do not promote new topics/content in WP, but merely reflect/summarize which new content/topic gets promoted in external publications.
- c) Whether there is a toxic atmosphere in WP or not clearly has no bearing on the decision whether some content should be included or not. We include material we regard as encyclopedically relevant and not to avoid a potential impression of toxicity. While I agree that the atmosphere and content disputes in WP can be quite toxic and that rules and formalities are often (mindlessly) overemphasized, I don't believe that to be the case here. Moreover imho the fact encyclopedic writing by its very nature often can be a dry, sober and even boring affair is sometimes confused with toxicity. This is simply how writing in WP differs from writing books, journal articles, blogs, other Wikis, etc. Those places allow for enthusiastically writing about new material, promoting it, taking a personal viewpoint/opinion/focus, but WP (for the most part) does not.
--Kmhkmh (talk) 01:10, 3 April 2023 (UTC)
"New Orleans teens’ Pythagorean proof gains compelling evidence"
BBC News -
- For generations, mathematicians maintained that any alleged proof of the Pythagorean theorem based in trigonometry would constitute a logical fallacy known as circular reasoning: seeking to validate an idea with the idea itself.
- In the abstract for their 18 March talk in Atlanta, at an event that drew presenters from prominent universities, Johnson and Jackson noted that the book thought to hold the largest known collection of proofs for the theorem, The Pythagorean Proposition by Elisha Loomis, “flatly states that ‘there are no trigonometric proofs because all the fundamental formulae of trigonometry are themselves based upon the truth of the Pythagorean theorem’”.
- But Johnson and Jackson said they found a way to use the trigonometry law of sines to prove Pythagoras’s theory in a way “independent of the Pythagorean trig identity sin2x+cos2x=1” – without resorting to circular reasoning.
https://www.theguardian.com/us-news/2023/apr/07/new-orleans-teens-pythagorean-theory
Depending on how this sorts out we might want to add something about it to the article.
- 186.215.16.37 (talk) 18:34, 8 April 2023 (UTC)
- This is lengty discussed above. D.Lazard (talk) 18:54, 8 April 2023 (UTC)
Trigonometric proof using Einstein's construction
There are already several videos in YouTube on Jackson and Johnson. Users @User:Kmhkmh and @user:jacobolus objected to inclusion because "the high school students have not published their result yet." OK, fine! Do not include Jackson and Johnson inside the main article as long as you wish! However, it is important to fix the content of the main article, because there is a repeated construction in Proof using similar triangles and Einstein's proof by dissection without rearrangement. In fact, the very same construction can be used to give a trigonometric proof of the Pythagorean_theorem without the need of summation of infinite geometric series.
Already students in math.stackexchange ask whether this is a valid "trigonometric" proof and whether it is correct. There were a number of trolls who immediately claimed that the proof is "circular" which it is not. So, I highly recommend that this trigonometric proof is discussed alongside the Einstein's proof and the proof with similar triangles. All these 3 proofs are essentially 3 different viewpoints on the same construction. Also, my previous discussion with @user:jacobolus applies - it needs to be pointed out that the high school definition of "sine" and "cosine" as ratios require as a special intermediate step the proof that non-congruent similar triangles exist based on Euclid's parallel postulate. Thus, the trigonometric proof is not circular as the concept of "similarity" is already needed in the proof by similar triangles and also Einstein's derivation based on proportionality of areas. Danko Georgiev (talk) 15:31, 7 April 2023 (UTC)
- Also, I would like to point out that the verbose textual drivel on Einstein's proof is not a proof at all, and will not be understandable to a high school student!!! Are you guys writing Wikipedia for yourselves? Bear in mind that that Wikipedia should not be understandable only for retired mathematicians, but to teenagers in high school too. So, if you do not object, I would like to insert the Trigonometric proof either inside Einstein's proof section or as a separate section immediately Einstein's proof section. Also, I would like to move all 3 proofs immediately following one after another. It makes no sense Einstein's proof not to be immediately following the proof with similar triangles. Also, being "Editor" implies that you should be taking care of the overall structure and logical flow of sections. I frankly do not understand who voted this article to be a "featured article" as the flow of logic is interrupted and related material appears all over the place. The identity of the two constructions mention by me is just one example of "duplicate" figure. In fact, from a single figure prepared by me above, one can write all 3 proofs in logical sequence one after another. Danko Georgiev (talk) 15:43, 7 April 2023 (UTC)
- This is the same proof about which you said above: “The proof given by Bogomolny is circular.” (Except that version used a unit-length hypotenuse.) I agree with your updated take that it’s fine (and also already essentially included in the article, just without labeling the sides as sines/cosines per se). As we were discussing above, whether this counts as “trigonometric” and whether proofs by similar triangles which re-label the sides as sines and cosines are different from the same proofs just using labels a, b, c is a semantic question rather than a mathematical question.
... fix the content of the main article,
– as far as I can tell the main article doesn’t make any false claims about this and doesn’t need to be “fixed”. But as I said above, a short section about “trigonometric proofs” could probably be added. –jacobolus (t) 15:49, 7 April 2023 (UTC)- The proof given by Bogomolny is circular because in step (1) he introduces "coordinates", in step (2) he uses "lengths", and in steps (3) and (4) he claims to prove the Pythagorean theorem. The circular reasoning comes from the fact that one cannot use the "coordinates" for calculation of "lengths" without using the Pythagorean theorem. If you already have the Pythagorean theorem in use between steps (1) and (2), you no longer need to prove it again in steps (3) or (4). The length of the line connecting two points with cartesian coordinates and is given by the Pythagorean theorem . The length of the line connecting two points with polar coordinates and is given by the Pythagorean theorem . Are we done with Bogomolny's proof? It is not the same as the proof given above by me because I do not introduce "coordinates" as step (1) in my proof. Also, in Eastern Europe where Bogomolny and myself are born and educated, if you introduce irrelevant stuff that you do not make use of in a math exam, you will get minus points from your overall score. In mathematical proofs you are not supposed to define and introduce stuff that you do not use or cannot use in your proof. Danko Georgiev (talk) 16:19, 7 April 2023 (UTC)
- “Coordinates" is a red herring. You are misinterpreting the statement, which is a shorthand for a geometrical argument essentially identical to the one you listed here. ––jacobolus (t) 19:43, 8 April 2023 (UTC)
- The proof given by Bogomolny is circular because in step (1) he introduces "coordinates", in step (2) he uses "lengths", and in steps (3) and (4) he claims to prove the Pythagorean theorem. The circular reasoning comes from the fact that one cannot use the "coordinates" for calculation of "lengths" without using the Pythagorean theorem. If you already have the Pythagorean theorem in use between steps (1) and (2), you no longer need to prove it again in steps (3) or (4). The length of the line connecting two points with cartesian coordinates and is given by the Pythagorean theorem . The length of the line connecting two points with polar coordinates and is given by the Pythagorean theorem . Are we done with Bogomolny's proof? It is not the same as the proof given above by me because I do not introduce "coordinates" as step (1) in my proof. Also, in Eastern Europe where Bogomolny and myself are born and educated, if you introduce irrelevant stuff that you do not make use of in a math exam, you will get minus points from your overall score. In mathematical proofs you are not supposed to define and introduce stuff that you do not use or cannot use in your proof. Danko Georgiev (talk) 16:19, 7 April 2023 (UTC)
I have now moved the three proofs in sequential order, one after another, and have replaced a somewhat duplicate image using the more informative one with labeled height to hypotenuse and lengths of sides. I have wikified a bit, but if the text can be made more comprehensible to a high school student, please go ahead and improve it. Danko Georgiev (talk) 18:06, 7 April 2023 (UTC)
Einstein's proof or Euclid's second proof?
Actually, Euclid has two proofs of the Pythagorean Theorem, namely Book I, Proposition 47 and Book VI, Proposition 31. The so-called Einstein's proof is, in fact, Euclid's second proof. Furthermore, this proof also appears in Loomis's collection of 1968, being attributed to Stanley Jashemski (contradicting Schroeder). Should this proof be credited to Einstein? Isn't this an example of Stigler's law of eponymy? Is Schroeder's reference more reliable than Loomis's? I personally think it should be entitled "Euclid's Second Proof" and perhaps state later that it was rediscovered by Stanley Jashemski (quoting Loomis) and/or Albert Einstein (quoting Schroeder). — Preceding unsigned comment added by 109.253.191.18 (talk) 20:36, 8 April 2023 (UTC)
- This article mentions the similarity-based proof from VI.31 but only in the "generalizations" section. It’s not quite the same as "Einstein's" proof (credited to Einstein by Schroeder (1991) because Einstein reportedly found it independently at age 11, not necessarily because he was the first to discover it) which is based on a dissection of the original right triangle's area, not just any arbitrary similar figures erected on each side. But I agree with you the VI.31 proof should probably be discussed earlier. There should probably also be some discussion about why Euclid included the first proof using only the propositions from Book I, considering that the second proof is so much less tricky to follow once the Book V theory of proportions has been developed. –jacobolus (t) 21:10, 8 April 2023 (UTC)
- I did not realise VI.31 is mentioned in the article, thanks for the correction. However, I still think that the proof of VI.31 is Einstein's proof word-for-word [2] and that it is unfair to attribute it to Einstein. Furthermore, this attribution spreads the misconception that confronts Einstein's "simple" proof against Euclid's "overcomplicated" proof, which is very unfortunate knowing about VI.31. 109.253.192.93 (talk) 21:56, 9 April 2023 (UTC)
- Continuing from my previous inconclusive reply (I am sorry my previous reply was so short and unconstructive), yes, my main suggested correction to the article is that VI.31 should be mentioned in the proofs section (and not only as a generalisation) because Euclid provided an alternative proof in VI.31 without citing I.47. Also, I agree that it might be nice to add a discussion briefly explaining why Euclid gave two different proofs. However, before making these modifications, it is worth clarifying whether or not Einstein's proof is the same as Euclid's second proof.
- If they are the same, as I think, they should share the same item. In that case, I suggest that this proof should be retitled as "Euclid's second proof", indicating that it is also known as Einstein's proof because it was rediscovered by Einstein. Alternatively, it can keep its current title, but the article should make clear that it is already VI.31 and it is not original of Einstein — so the reader does not get the misconception I mention in my previous comment.
- Otherwise, if I am wrong and Einstein's proof and VI.31 are different, then we should add something about VI.31 in the proofs section: either along with I.47 or as a new item. 109.253.192.93 (talk) 17:19, 10 April 2023 (UTC)
- They are not really the same. Both use the concept of similarity, but "Einstein's" proof (whoever may have first written it) is about decomposition of the triangle (that is, making one area by physically pasting the other two areas together), whereas Euclid's proof in VI.31 is based on using a sum of lengths of the two parts of side c, along with VI.19: "if three straight lines are proportional, then the first is to the third as the figure described on the first is to that which is similar and similarly described on the second." (In algebraic notation we might write this as though that is somewhat conceptually anachronistic.) –jacobolus (t) 18:13, 10 April 2023 (UTC)
- Ok, you are completely right. Although they are very similar, they are not the same. I can see the difference now. In Einstein's proof one compares the squares with the triangles, while in Euclid's proof one compares the squares with the two parts of the hypotenuse (the projections of the legs). I am writing them below in a parallel way to remark the similarities and where the difference is. Thank you very much for your reply.
- Einstein's proof: Let ABC be a right triangle with hypotenuse AC and BD be perpendicular to AC (with D point in AC). Note that ADB is the right triangle similar to ABC with hypotenuse AB and BDC is the right triangle similar to ABC with hypotenuse BC (by VI.8). Now, the square of AC is to the square of AB as ABC is to ADB and, similarly, the square of AC is to the square of BC as ABC is to BDC (by VI.20). Hence, ABC is to ADB,BDC as the square of AC is to the sum of the squares of AB and BC (by V.24). Now, the sum of ADB,BDC equals ABC, so the square of AC equals the sum of the squares of AB and BC.
- Euclid's second proof: Let ABC be a right triangle with hypotenuse AC and BD be perpendicular to AC (with D point in AC). Note that ADB is the right triangle similar to ABC with hypotenuse AB and CDB is the right triangle similar to ABC with hypotenuse CB (by VI.8). Hence, AC is to AD as twice AC is to AB and, similarly, AC is to DC as twice AC is to BC. Thus, the square of AC is to the square of AB as AC is to AD and, similarly, the square of AC is to the square of BC as AC is to DC (by VI.20). Hence, AC is to AD,DC as the square of AC is to the sum of the squares of AB and CB (by V.24). Now, the sum of AD,DC equals AC, so the square of AC equals the sum of the squares of AB and CB. 109.253.210.184 (talk) 21:28, 10 April 2023 (UTC)
- In my opinion this article would be improved by a bit of reorganization. I think it should start (in a section immediately after the lead) with a few diagram-heavy proof sketches and plain-language discussion about the basic historical and mathemtical context (and cut the "Other forms of the theorem" section which is trivial and distracting), explaining that we don't precisely know what the first proof may have been like, but mentioning some of the speculation about why Euclid included both the first proof I.47 as well as the second proof VI.31. I'm not sure what such an introductory section should be called; or maybe it could just be the top part of the proofs section.
- Then all of the proofs should IMO be moved into a section "proofs", starting first with spelled-out proofs I.47 (including some discussion of the other propositions in book I which it is built on), VI.31 (explaining the propositions / methods on which it is based, and perhaps also describing the "Einstein" variant), then continuing to a few graphical re-arrangement proofs, and then several more subsections about others of different styles. ––jacobolus (t) 22:19, 10 April 2023 (UTC)
- I completely agree, the article needs some reorganisation. Unfortunately, it is semiprotected and I cannot make editions. The section about "Other forms of the theorem" adds nothing, as you say. I would start with the "History" section first. Then, as you say, all the proofs should be given in one section called "Proofs", with an introduction indicating that this theorem has many proofs (it can cite Loomis for example) and that here we only collect a short amount. This section should start with a subsection called "Visual proofs" with a few diagram-heavy and animeted proofs — for instance, the proofs by rearrangement, dissection and area preserving shearing should be put together here. The next subsection should be "Euclid's proofs" containing I.31 and VI.31 and the speculation about why Euclid gave two proofs. After that, it can give as variations of VI.31 "Einstein's proof", "Proof using similar triangles" (which is a modern way of doing VI.31) and "Trigronometric proof using Einstein's construction" (which is just a rewriting of the previous one by replacing a/c by the sine and b/c by the cosine). Finally, it should end with a subsection about the algebraic proofs and a subsection about the analytic proofs. Next, the section "Consequences and uses of the theorem" should be probably retitled "Related results" and the "Converse" section could be a subsection of this section. The final section should be "Generalizations" and perhaps it should mention Parseval's identity at the end of "Inner product spaces" subsection. 109.253.210.184 (talk) 18:39, 11 April 2023 (UTC)
- They are not really the same. Both use the concept of similarity, but "Einstein's" proof (whoever may have first written it) is about decomposition of the triangle (that is, making one area by physically pasting the other two areas together), whereas Euclid's proof in VI.31 is based on using a sum of lengths of the two parts of side c, along with VI.19: "if three straight lines are proportional, then the first is to the third as the figure described on the first is to that which is similar and similarly described on the second." (In algebraic notation we might write this as though that is somewhat conceptually anachronistic.) –jacobolus (t) 18:13, 10 April 2023 (UTC)